Cumulus Parameterization and Mass-Flux Schemes in Atmospheric Science

Explore the significance of mass-flux schemes in cumulus parameterization, their interaction with grid-scale microphysics, and the key elements and assumptions involved. Learn about the objectives, components, and limitations of classical cumulus schemes for atmospheric modeling. Gain insights into the three elements of a mass-flux scheme, the five shared assumptions, and the scheme formulation.

• Cumulus parameterization
• Mass-flux schemes
• Grid-scale microphysics
• Atmospheric science
• Subgrid-scale processes

add_bie Follow

Uploaded on Sep 17, 2024 | 0 Views

Download Presentation

Please find below an Image/Link to download the presentation.

The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author. Download presentation by click this link. If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.

Presentation Transcript

1. April 12, 2018 Hing Ong ATM 419/563 Cumulus parameterization: Kain-Fritsch scheme and other mass-flux schemes This lecture is designed for students who have some background in cumulus parameterization.

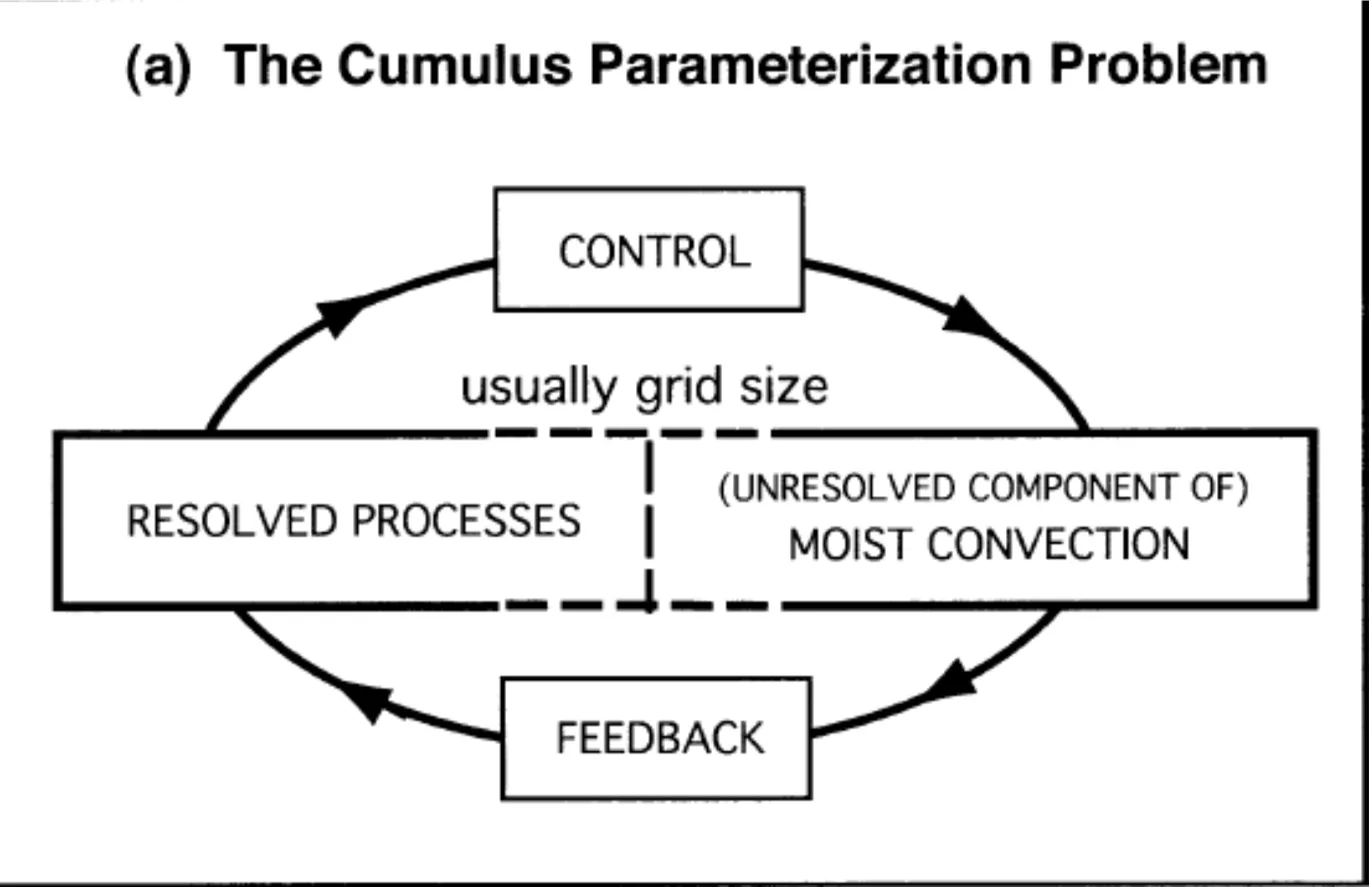
2. The cumulus parameterization problem Cumulus parameterization 04/12/2018 2

3. Why mass-flux schemes? They can interact with grid-scale microphysics schemes. For example, parameterized cumuliform clouds can produce resolved stratiform clouds through hydrometeor detrainment. They can transport any mass-coupled quantity. For example, momentum, aerosol contents, etc. Betts-Miller-Janjic scheme is the only non-mass-flux scheme used in WRF, and it can do none of the above. Cumulus parameterization 04/12/2018 3

4. Objective Students should become capable of the following: 1. Name the three elements comprising a mass-flux scheme. 2. Understand the five assumptions shared by classical mass- flux schemes. 3. Name the two subgrid-scale processes attributing apparent sources or sinks. 4. Know the iterative sequence of Kain-Fritsch scheme and how CUDT can affect the parameterization. 5. Understand the limits of classical cumulus schemes. Cumulus parameterization 04/12/2018 4

5. The Three Elements A trigger function Determining if the subgrid-scale convection may occur. A one-dimensional cloud model Calculating intensive quantities ( , qv, qc, etc.) and relative strengths between types of mass flux (?, ?, and ?) associated with the subgrid-scale convective drafts. A closure Adjusting the absolute strengths of the mass flux. Cumulus parameterization 04/12/2018 5

6. The Five Assumptions 1. Ensemble average is equal to grid-box average. 2. Each convective drafts and environment are horizontally homogeneous. 3. Convective drafts remain in steady states within a characteristic time of convective adjustment. 4. Fractional area covered by convective drafts in the grid cell is negligible. 5. Convective mass flux is locally compensated by environmental mass flux. Cumulus parameterization 04/12/2018 6

7. ??? ?? + ? ?? ? = ? ? + SSS? ? ? ??+ ?? ? = AS? Scheme Formulation ? unity or an intensive quantity Applying the five assumptions: SSS? 1 ? ? AS? ? +1 ? ? SSS? subgrid-scale sources or sinks ?? ? + ????+ ????? ????? AS? apparent sources or sinks grid box volume entrainment rate detrainment rate vertical mass flow rate convective draft over bar ensemble average environment (tilde) ? upper boundaries ? lower boundaries ???? ? + ???? ?? ? ?? ?? ? ? Cumulus parameterization 04/12/2018 7

8. Recaps The five assumptions are applied to formulate classical mass-flux schemes in a form of either the subgrid-scale sources (SSS) or the apparent sources (AS). The three elements are applied to determine the unknown variables in either SSS or AS formula. Detrainment or mass compensation attributes AS while entrainment does not. Kain-Fritsch scheme is formulated in the SSS form, but it is coupled with WRF in the AS form. Cumulus parameterization 04/12/2018 8

9. Iterative sequence of Kain-Fritsch scheme Trigger Updraft Downdraft Compensation Closure Every CUDT If convection is still active then Leave it unchanged. Else Start the trigger function Determine a parcel as a mixture of the lowest 50 hPa. Calculate ????. Cumulus parameterization 04/12/2018 9

10. Iterative sequence of Kain-Fritsch scheme Trigger Updraft Downdraft Compensation Closure Then, calculate ?????. 0.33K m-0.33 s0.33, where ?????= 4.64 ? ? ? ?? if CUDT ??,otherwise CUDT ?? CUDT ?? CUDT+ ? ? = 10 5???? s 1 if ???? 2000 m 0.02 m s 1 if ????> 2000 m Note that the trigger function is temporally nonlocal when CUDT > dt. Then, go on if ????+ ????? ????. Otherwise, go back and test the next 50 hPa if it is within the lowest 300 hPa. CUDT?? and ?? = ?? Cumulus parameterization 04/12/2018 10

11. Iterative sequence of Kain-Fritsch scheme Trigger Updraft Downdraft Compensation Closure Then, compute updraft properties. ??????= ??????+ ?? ? ????+ ?(no storage) Lift a parcel adiabatically from the updraft source layer (USL) to LCL, and assume ????= 1 + 0.5 1000????? If it exceeds 3 m s-1, set to 3 m s-1. ?2 2 = ? ??? ? ? 0.5 m s-1. ????? ?? and ?? are upstream quantities. ? ?? ??? ??? ? ??? ???2 Cumulus parameterization 04/12/2018 11

12. Iterative sequence of Kain-Fritsch scheme Trigger Updraft Downdraft Compensation Closure Then, compute updraft properties. ??????= ??????+ ?? ? ????+ ?(no storage) 0.03?? ? m Pa-1. ???= ??LCL 1000 m if ? ? ? < 0 m s 1 2000 m if ? ? ? > 0.1 m s 1 ? ? ? 0.1 m s 1 m else ?? and ?? are upstream quantities. ? = 1000 1 + ?? and ?? are diagnosed with a buoyancy sorting algorithm. Set ?? 0.5??? ? is diagnosed with a single moment five species (qv, qc, qr, qi, and qs) microphysics scheme. Accordingly, ?? is updated. Cumulus parameterization 04/12/2018 12

13. Iterative sequence of Kain-Fritsch scheme Trigger Updraft Downdraft Compensation Closure Then, compute updraft properties. Cloud top (TOP) is where upward motion stops. Detrain all updraft mass above level of equilibrium temperature (LET). TOP 4000 m if ????> 20 2000 m if ????< 0 2000 m + 100????m 1 else If updraft depth > ????, deep convection is activated, and if not, go back and test the next 50 hPa if it is within the lowest 300 hPa. If all the USLs in the lowest 300 hPa do not meet the ???? criterium, shallow convection is activated. LET ????= Cumulus parameterization 04/12/2018 13

14. Iterative sequence of Kain-Fritsch scheme Trigger Updraft Downdraft Compensation Closure If convection is activated, Compute convective timescale (TIMEC) For deep convection, TIMEC =?? ? = 0.5 ? ??? [30,60] mn, set to 30 or 60 mn. For shallow convection, TIMEC = 40 mn. Setup a countdown timer so that KF will not be called again until min TIMEC,?? Note that the CAPE, intensive quantities, and relative strengths between types of mass flux in the updraft has been determined. ?, where If it is beyond TOP + ? 0.5?? LET ?. Cumulus parameterization 04/12/2018 14

15. Iterative sequence of Kain-Fritsch scheme Trigger Updraft Downdraft Compensation Closure Then, compute downdraft properties. Downdraft source layer (DSL) lies 150 hPa above USL. Intensity quantities are constrained by 100 % above cloud base (?????) 100 % 1000 m????? ? ???= 20 % Mass flux is constrained by ??USL ??USL= 2 1 ?? Precipitation is hydrometeors that are generated minus detrained and evaporated. DSL USL Cumulus parameterization 04/12/2018 15

16. Iterative sequence of Kain-Fritsch scheme Trigger Updraft Downdraft Compensation Closure Then, compute mass compensation. ?? ? + ????+ ????? ?????= 0 Retrospect 1 ? ? ?? ? + ????+ ????? ?????. All the unknowns on RHS have been determined, aren t they? SSS? ?? and ?? are upstream quantities. Cumulus parameterization 04/12/2018 16

17. Iterative sequence of Kain-Fritsch scheme Trigger Updraft Downdraft Compensation Closure The unclosed mass flux does not guarantee removal of at least 90 % of CAPE within TIMEC! Iterate for closure. Use the unclosed SSS? to extrapolate ? after TIMEC, and compute adjusted CAPE. If at least 90 % of CAPE is removed, done. If not, multiply all the types of mass flux and precipitation by a constant and reiterate. ?? and ?? are upstream quantities. Cumulus parameterization 04/12/2018 17

18. Iterative sequence of Kain-Fritsch scheme Trigger Updraft Downdraft Compensation Closure WRF physics coupler does not support SSS? feedback but does AS? feedback. ?? ?? ????? AS?= ?? and ?? are upstream quantities. Cumulus parameterization 04/12/2018 18

19. Recaps The aim is to diagnose the unknowns on RHS of SSS? 1 ? ? ?? ? + ????+ ????? ????? CUDT controls the weight of the latest w in the trigger function, which is temporally local when CUDT dt. ?2 2 ? ?? dimensional cloud models. ??? ??? ??? ? ??? ???2 is used to integrate one- = ? CAPE closure is used. In WRF, KF scheme adjusts , qv, qc, qi, qr, qs, but not u, v Details are complicated and can vary from one version to another, this introduction is based on the code in WRFV3.7.1. Cumulus parameterization 04/12/2018 19

20. Limits of classical cumulus schemes. With the grid spacings shrinking from 27 km to 9 km, (1) convective draft fraction may become considerable, (2) compensating mass flux may spread to adjacent grid. Options dealing with issue (1) are as follows: Grell Freitas Ensemble Scheme (03) Multi scale Kain Fritsch Scheme (11) Options dealing with issue (2) are as follows: Grell 3D Ensemble Scheme (5) with artificial nonlocal compensation Hybrid Mass Flux Kain-Fritsch Scheme with dynamic compensation None of the options deal with both issues. Cumulus parameterization 04/12/2018 20

21. Conclusion 1. Name the three elements comprising a mass-flux scheme. A trigger function, a one-dimensional cloud model, and a closure 2. Understand the five assumptions shared by classical mass-flux schemes. Average, top hat, steady, fraction, compensation 3. Name the two subgrid-scale processes attributing apparent sources or sinks. Mass compensation and detrainment 4. Know the iterative sequence of Kain-Fritsch scheme and how CUDT can affect the parameterization. the trigger function is temporally nonlocal when CUDT > dt 5. Understand the limits of classical cumulus schemes. Convective draft fraction and local compensation Cumulus parameterization 04/12/2018 21

22. Questions & Discussion Cumulus parameterization 04/12/2018 22

23. Discussions: future development Eliminating assumptions for scheme formulation one by one, a hierarchy with six levels could be built. To eliminate assumption (1) and build a HYMACS These are NOT needed: More sophisticated assumptions Extra computer resources These are ALL needed: A cumulus scheme diagnosing convective mass flux A fully compressible nonhydrostatic model Cumulus parameterization 04/12/2018 23