Brackets] Brackets]
F
i
n
i
s
h
F
a
c
t
o
r
i
s
i
n
g
[
T
r
i
p
l
e
B
r
a
c
k
e
t
s
]
Silent
Teacher
Narration
Your Turn
Intelligent
Practice
Practice
Worked Example
Your Turn
@mansbridgemaths
Finish factorising:
Finish factorising:
Worked Example
Your Turn
@mansbridgemaths
Finish factorising:
Finish factorising:
Where possible, finish factorising:
@mansbridgemaths
Where possible, finish factorising:
@mansbridgemaths
Where possible, finish factorising:
@mansbridgemaths
Where possible, finish factorising:
@mansbridgemaths
In this factorising exercise, you will practice factoring expressions involving triple brackets by following worked examples and then attempting problems on your own. The narration guides you through different scenarios to help you improve your factorisation skills efficiently.
Download Presentation
Please find below an Image/Link to download the presentation.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author.If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.
You are allowed to download the files provided on this website for personal or commercial use, subject to the condition that they are used lawfully. All files are the property of their respective owners.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author.
E N D
Presentation Transcript
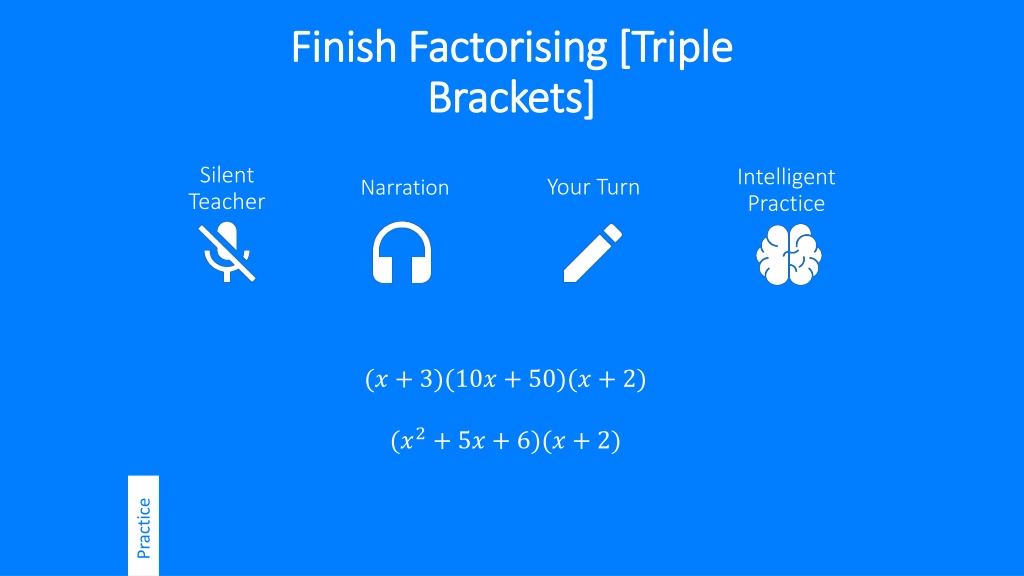
Finish Factorising [Triple Finish Factorising [Triple Brackets] Brackets] Silent Teacher Intelligent Practice Your Turn Narration (? + 3)(10? + 50)(? + 2) (?2+ 5? + 6)(? + 2) Practice
Worked Example Your Turn Finish factorising: Finish factorising: (? + 3)(10? + 50)(? + 2) (? + 3)(5? + 15)(? + 2) (?2+ 5? + 6)(? + 2) (?2+ 5? 6)(? + 2) @mansbridgemaths
Worked Example Your Turn Finish factorising: Finish factorising: (? + 3)(10? + 50)(? + 2) (? + 3)(10? + 15)(? + 2) 5(? + 3)(2? + 3)(? + 2) 10(? + 3)(? + 5)(? + 2) (?2+ 5? + 6)(? + 2) (?2+ 5? 6)(? + 2) (? + 6)(? 1)(? + 2) (? + 2)(? + 3)(? + 2) @mansbridgemaths
Where possible, finish factorising: 1. 2. 3. 4. 5. 6. 7. 8. 9. 10. 3 10? + 30?2(? + 10) 10? + 30 ? + 1 3? + 2 ? + 3 ? + 1 30? + 20 10? + 30 ? + 1 30? + 20 10? + 30 ? + 10 30? + 20 (10? + 30)(? + 10)(30? 20) 3 10? + 30 ? + 10 30? 20 3 10? + 30 ? + 10(30? 20) 3 10? + 30 ? + 10(10? + 30) 3 10? + 302(? + 10) @mansbridgemaths
Where possible, finish factorising: 1. 2. 3. 4. 5. 6. 7. 8. 9. 10. 3 10? + 30?2(? + 10) 10? + 30 ? + 1 3? + 2 ? + 3 ? + 1 30? + 20 10? + 30 ? + 1 30? + 20 10? + 30 ? + 10 30? + 20 (10? + 30)(? + 10)(30? 20) 3 10? + 30 ? + 10 30? 20 3 10? + 30 ? + 10(30? 20) 3 10? + 30 ? + 10(10? + 30) 3 10? + 302(? + 10) 10 ? + 3 ? + 1 3? + 2 10 ? + 3 ? + 1 3? + 2 100(? + 3)(? + 1)(3? + 2) 100(? + 3)(? + 10)(3? + 2) 100(? + 3)(? + 10)(3? 2) 300(? + 3)(? + 10)(3? 2) 30? ? + 3 + 100(3? 2) 10(3? + 10)(? + 3) 300 ? + 32(? + 10) 120?2(? + 10) @mansbridgemaths
Where possible, finish factorising: ? + 3 ?2 16 ? + 3 ?2+ 16 ? + 3 ?2+ 10? + 16 (? + 3)(?2+ 8? + 16) (? + 3)(4?2+ 8? + 16) (? + 3)(4?2+ 8? + 4) ? + 3 4?2+ 8? 5 5(? + 3)(4?2+ 8? 5) 5 ? + 3 4?2+ 8? 12 1. 2. 3. 4. 5. 6. 7. 8. 9. 10. 5(? + 3)(4?2+ 8?) @mansbridgemaths
Where possible, finish factorising: ? + 3 ?2 16 ? + 3 ?2+ 16 ? + 3 ?2+ 10? + 16 (? + 3)(?2+ 8? + 16) (? + 3)(4?2+ 8? + 16) (? + 3)(4?2+ 8? + 4) ? + 3 4?2+ 8? 5 5(? + 3)(4?2+ 8? 5) 5 ? + 3 4?2+ 8? 12 1. 2. 3. 4. 5. 6. 7. 8. 9. 10. 5(? + 3)(4?2+ 8?) (? + 3)(? 4)(? + 4) (? + 3)(?2+ 16) ? + 3 (? + 2)(? + 8) ? + 3 ? + 42 4(? + 3)(?2+ 2? + 4) 4 ? + 3 ? + 12 (? + 3)(2? + 5)(2? 1) 5(? + 3)(2? + 5)(2? 1) 20(? + 3)(? + 3)(? 1) 20?(? + 3)(? + 2) @mansbridgemaths
