Angular Distribution of Axial Vector Meson Decay
Explore the decay and angular distribution of (Axial) Vector mesons in different modes, aiming to distinguish between transverse and longitudinal modes through two-body decay distribution. Focus on K* and K1 as chiral partners. Efforts are made to differentiate mass differences in vacuum and medium for spin-1 particles. Discusses the effective Lagrangian, polarization tensor, and angular dependence in the context of chiral symmetry breaking.
Download Presentation

Please find below an Image/Link to download the presentation.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author. If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.
You are allowed to download the files provided on this website for personal or commercial use, subject to the condition that they are used lawfully. All files are the property of their respective owners.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author.
E N D
Presentation Transcript
(Axial)Vector meson decay and angular distribution In Woo Park Yonsei University Nuclear Theory and Hadron Group Author: Hiroyuki Sako, Kazuya Aoki, Philipp Gubler, Su Houng Lee
Purpose In vacuum, longitudinal and transverse modes of spin 1 are identical. In medium, their masses differ when they move with respect to the medium. Therefore we want to see whether if we can distinguish the transverse and longitudinal mode through their two body decay distribution. Here, we will concentrate on K* and K1 because they are chiral partners. S.H.Lee,``Chiral Symmetry Breaking and the Masses of Hadrons: A Review, Symmetry 15, 799 (2023)
Contents 1. Lagrangian and coupling constant for each decay process 2. Polarization tensor of the initial particle and angular dependence 3. General angular distribution calculation Phenomenological Lagrangian Wigner rotation matrix 4. Summary
Effective Lagrangian ? ?? ? ?? ?1 ??, ?1 ? ? Effective lagrangian reference: 10.1016/j.physletb.2021.136388
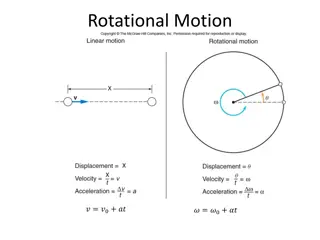
Kinematics Initial quantization axis is along the 3- momentum of the initial particle in its own rest frame.
Decay width and coupling constant ? ??,? ?? ?(MeV) 149 51.4 34.2 18.9 Decay g 5.96 3.27 3.26 0.71 ? ?? ? (???) ?? ?????? ?? ?1 ??,?1 ? ? ?????? ? ??? ?
Transverse and longitudinal disentangled ? ??,? ??
Transverse and longitudinal disentangled ?1 ??,?1 ? ?
General angular distribution 1. Phenomenological Lagrangian Initial (axial)vector meson state is a superposition of 3 different helicity states of J=1 particle. ? ?1: Respective amplitude = ?? ?? ?? ? ?1?? ? ?1? ? ?1 ? ?1
General angular distribution 2. Wigner rotation matrix Spin in Particle Physics E.~Leader, Camb. Monogr. Part. Phys. Nucl. Phys. Cosmol. 15, pp.1-500 Cambridge University Press, 2023
?? ?? ?? ?1
Method to discriminate T and L mode of ?1 Initial ?1polarization is hardly distinguished Measure the final vector meson polarization ?1 ??
?????? ?? ??(????) ? ?
Summary Polarization of the parent vector meson can be distinguished by looking at decay angle. Same method can be applied to the calculation of angular distribution of ?2 ? + ?. ? + ? Initial quantization axis=Global angular momentum.