Computational Complexity in Quantum Hamiltonians
The Bose-Hubbard model is proven to be QMA-complete, indicating the challenge in solving ground energy problems in quantum systems. Various classes of Hamiltonians, such as k-local and stoquastic, exhibit different complexities in computing ground energy. While some systems with QMA-complete ground
1 views • 40 slides
Advanced Topics in Quantum Physics
Dive deep into concepts like the Haldane Model, Chern Numbers, and Linear Response Theory in Quantum Physics. Understand the intricate relationships between magnetic fields, electron Hamiltonians, and conductivity theories. Explore the fascinating world of quantum Hall effects and gauge invariance i
1 views • 15 slides
Exploring the Role of Bi-Polarons in High Temperature Superconductivity Research
This research delves into the concept of bi-polarons and their potential involvement in high-temperature superconductivity mechanisms. Collaborators from various institutions are investigating the properties and interactions of bi-polarons, considering factors like effective mass and density. The st
1 views • 26 slides
Crystal Field Theory and Atomic Basis Sets
Explore the principles behind crystal field theory, including the concept of effective Hamiltonians to mimic covalent bonding in solids. Learn how to expand potentials on spherical harmonics and understand the selection rules and symmetry considerations in this theoretical framework.
0 views • 8 slides
Insights into Information Propagation in Long-Range Interacting Quantum Systems
Explore the dynamics of isolated quantum systems through quantum quenches, Lieb-Robinson bounds, and the Transverse Ising Model. Delve into experiments with one-dimensional quantum gases and long-range interactions in Hamiltonians, shedding light on information exchange, relaxation, and equilibrium
0 views • 20 slides
QMA(2): Hamiltonians, Provers, and Complexity Classes
Exploring the complexities of QMA(2) through discussions on separable sparse Hamiltonians, the power of Merlin in L.QMA, the impact of prover restrictions on complexity classes like IP and MIP, and the difference between Merlin.A, Merlin.B, and Arthur in L.QMA(2). Delve into short proofs for NP-Comp
0 views • 27 slides
Quantum Adiabatic Optimization vs. Quantum Monte Carlo
This content delves into the comparison between Quantum Adiabatic Optimization and Quantum Monte Carlo methods in quantum computing, discussing their approaches, algorithms, potential applications, and theoretical possibilities. It explores the concepts of adiabatic theorem, simulated annealing, sto
0 views • 19 slides
Understanding Optical Properties of Semiconductors and Insulators in Solid State Physics
Explore the exciting world of optical properties in semiconductors and insulators with a focus on excitons, interband transitions, matrix elements, and real spectra analysis. Learn about the frequency dependence of optical properties and a simple treatment of exciton effects in a two-band model. Del
1 views • 22 slides
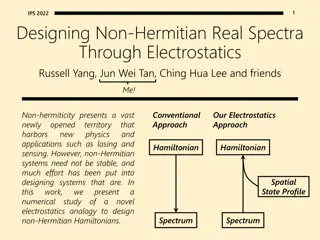
Exploring Non-Hermitian Systems Through Electrostatics and Real Spectra in IPS 2022
Discover the fascinating realm of non-Hermitian systems in IPS 2022, where innovative applications like lasing and sensing are being pursued. Dive into the design of stable non-Hermitian Hamiltonians using a novel electrostatics analogy. Explore the implications of real spectra and boundary conditio
0 views • 8 slides
Progress Towards Modeling of vFFA by Max Topp-Mugglestone
Learn about the progress in modeling the variable-field focusing accelerator (vFFA) through various Hamiltonians, closed orbits, optical components, and harmonic analysis. Understand the challenges and advantages of vFFA concept, as discussed by Max Topp-Mugglestone. Explore the fundamental Hamilton
0 views • 22 slides
Crystal Field Theory and Matrix Elements in Atomic Basis Sets
Explore the concept of crystal field theory expanded on renormalized spherical harmonics by Maurits W. Haverkort at Heidelberg University. Learn about effective Hamiltonians mimicking covalent bonding, matrix elements in the Hamiltonian, and the importance of symmetry in atomic basis sets. Discover
0 views • 8 slides
Understanding Classical Mechanics and Electromagnetism at USPAS, June 2018
Dive into a comprehensive review of classical mechanics and electromagnetism from the June 2018 USPAS program at MSU with topics covering oscillators, perturbation, special relativity, Lagrangians, Hamiltonians, phase-space, stability, canonical transformations, and accelerator Hamiltonians.
0 views • 13 slides
Understanding Atom-Photon Interactions: Methods and Results
Dive into the world of atom-photon interactions with Gilad Laredo's presentation from Spring 2017. Explore the transition probabilities, time-independent Hamiltonians, and the Floquet theorem. Learn how to solve complex equations and generalize problems in this fascinating field of study.
0 views • 18 slides
Rigorous RG Algorithms for Low-Energy Eigenstates in 1D Systems
Discover the rigorous renormalization group algorithms and area laws for low-energy eigenstates in 1D systems. Explore the algorithmic approaches and results for local Hamiltonians and ground state projections.
0 views • 13 slides