Understanding Geometric Optics: Mirrors, Light, and Image Formation
Delve into the world of geometric optics as you explore how light rays form images with optical instruments, understand the ray model of light, and discover the laws of reflection and refraction. From reflection and refraction on plane mirrors to key terms like specular reflection and virtual images, this comprehensive guide provides insights into image formation by a plane mirror through insightful diagrams and explanations.
Download Presentation

Please find below an Image/Link to download the presentation.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author. Download presentation by click this link. If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.
E N D
Presentation Transcript
Geometric Optics Mirrors, light, and image formation
Geometric Optics Understanding images and image formation, ray model of light, laws of reflection and refraction, and some simple geometry and trigonometry The study of how light rays form images with optical instruments
Reflection and refraction on plane mirrors REFLECTION AND REFRACTION AT A PLANE SURFACE
Key terms Anything from which light rays radiate Object Anything from which light rays radiate that has no physical extent Point object Real objects with length, width, and height Extended objects
Key terms Specular reflection Diffused reflection Reflection on a plane surface where reflected rays are in the same directions Relfection on a rough surface
Key terms Virtual image Real image Image formed if the outgoing rays actually pass through the image point Image formed if the outgoing rays don t actually pass through the image point
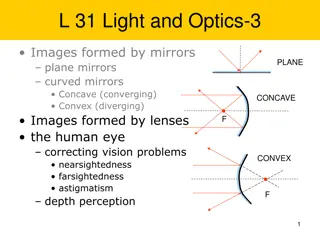
Image formation by a Plane mirror a diagram that traces the path that light takes in order for a person to view a point on the image of an object Ray Diagrams suggests that in order to view an image of an object in a mirror, a person must sight along a line at the image of the object. Line of Sight Principle
Image formation by a Plane mirror M M V s s
Image formation by a Plane mirror M is the object and M is the virtual image Ray MV is incident normally to the plane mirror and it returns along its original path s= object distance s = image distance s=-s
Image formation by a Plane mirror Sign rules For the object distance: When the object is on the same side of the reflecting or the refracting surface as the incoming light, s is positive For the image distance: When the image is on the same side of the reflecting or the refracting surface as the outgoing light, s is positive
Image of an extended object Q Q V y y M M s s V
Image of an extended object Lateral magnification Ratio of image height to object height M=y /y Image is erect m for a plane mirror is always +1 Reversed means front-back dimension is reversed
Reflection on Concave and Convex mirrors REFLECTION AT A SPHERICAL SURFACE
Reflection at a Concave Mirror V C P P
Reflection at a Concave Mirror Radius of curvature R Center of curvature The center of the sphere of which the surface is a part C Vertex The point of the mirror surface V CV Optic axis
Graphical Methods for Mirrors IMAGE FORMATION ON SPHERICAL MIRRORS
Graphical Method Consists of finding the point of intersection of a few particular rays that diverge from a point of the object and are reflected by the mirror Neglecting aberrations, all rays from this object point that strike the mirror will intersect at the same point
Graphical Method For this construction, we always choose an object point that is not on the optic axis Consists of four rays we can usually easily draw, called the principal rays
Graphical Method A ray parallel to the axis, after reflection passes through F of a concave mirror or appears to come from the (virtual) F of a convex mirror A ray through (or proceeding toward) F is reflected parallel to the axis A ray along the radius through or away from C intersects the surface normally and is reflected back along its original path A ray to V is reflected forming equal angles with the optic axis
Positions of objects for concave mirrors
Image formation by concave mirrors Position of object Position of image Character of image Real, inverted, reduced Real, inverted, same size Real, inverted, enlarged No image Virtual, upright, enlarged Virtual, upright, same size Beyond C Between F and C At C At C Between C and F Beyond C At F At infinity Between F and Vertex Beyond the vertex At V At V
Reflection at a Concave Mirror If dec, i is nearly parallel Rays nearly parallel or close to R Paraxial rays
Reflection at a Concave Mirror If inc, P is close to V Image is smeared out Spherical Aberration
Reflection at a Concave Mirror V F C s at infinity s = R/2
Reflection at a Concave Mirror All reflected rays converge on the image point Converging mirror If R is infinite, the mirror becomes plane
Reflection at a Concave Mirror The incident parallel rays converge after reflecting from the mirror They converge at a F at a distance R/2 from V f= R/2 f is the focal length, distance from the vertex to the focal point F is Focal point, where the rays are brought to focus
Reflection at a Concave Mirror V F C s at infinity s= R/2
Reflection at a Concave Mirror The object is at the focal point 1/s+ 1/s = 1/f Object image relation, spherical mirror s=f=R/2 1/s =0; s at infinity 1/s +1/s = 2/R
Image of an Extended Object Lateral magnification m= y /y Lateral m= y /y= -s /s magnification for spherical mirrors
Reflection at a Convex Mirror F C s or s= R/2 s or s at infinity
Image formation on spherical mirrors Sign rules For the object distance: When the object is on the same side of the reflecting or the refracting surface as the incoming light, s is positive; otherwise, it is negative
Image formation on spherical mirrors Sign rules For the image distance: When the image is on the same side of the reflecting or the refracting surface as the outgoing light, s is positive; otherwise, it is negative
Image formation on spherical mirrors Sign rules: For the radius of curvature of a spherical surface: When the center of curvature C is on the same side as the outgoing light, the radius of curvature is positive, otherwise negative
Reflection at a Convex Mirror The convex side of the spherical mirror faces the incident light C is at the opposite side of the outgoing rays, so R is neg. All reflected rays diverge from the same point Diverging mirror
Reflection at a Convex Mirror Incoming rays are parallel to the optic axis and are not reflected through F s is positive, s is negative Incoming rays diverge, as though they had come from point F behind the mirror F is a virtual focal point
Refraction at spherical interface REFRACTION AT A SPHERICAL SURFACE
Refraction at a Spherical Surface Object-image relation, spherical refracting surface na/s + nb/s = (nb-na)/R na/s + nb/s =0 At a plane refracting surface Lateral magnification, spherical refracting surface m=y /y= -(nas /nbs)
Biconcave and biconvex thin lenses GRAPHICAL METHOD FOR LENSES