Understanding Domain and Range of Functions
Understanding functions involves exploring concepts such as domain, range, and algebraic inputs. This content covers topics like constructing functions, common functions like quadratic and trigonometric, and solving functions based on given domain and range. It also provides practice questions to test understanding of functions and inputs, along with solutions for various scenarios.
Download Presentation

Please find below an Image/Link to download the presentation.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author. Download presentation by click this link. If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.
E N D
Presentation Transcript
Domain & Range of Functions
Overview #1: Understanding of functions #2: Domain/Range of common functions (particularly quadratic and trigonometric) #4: Constructing a function based on a given domain/range. #3: Domain/Range of other functions
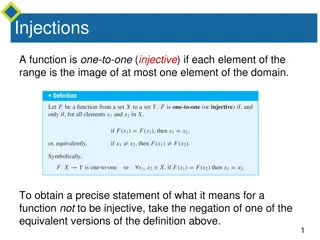
Check Your Understanding ?(?) = ?2+ 2 What does this function do? It squares the input then adds 2 to it. Q1 ? What is ?(3)? f(3) = 32 + 2 = 11 Q2 ? What is ?( 5)? f(-5) = 27 Q3 ? If ? ? = 38, what is ?? ??+ ? = ?? So ? = ? Q4 ?
Algebraic Inputs If you change the input of the function (?), just replace each occurrence of ? in the output. If ? ? = ?2 1 what is: If ? ? = ? + 1 what is: ? ? ? ? ? ? 1 = ? ?? ? = ?? ?? ? 2? = ??? ? = ??? ? ? ?2+ 1 = ??+ ? = ??+ ??? ? ? 1 = ? ? + ? = ? ? ?2= ??+ ? ? ?2= ? + ?? ? 2? = ?? + ? ? ? ? ? ? If ? ? = 2? what is: ? ? 1 = ? ? ? = ?? ? ? ?2= ??? ? ?2= ???= ??? ? ? ?
Check Your Understanding A If ? ? = 3? 1, determine: (a) ? ? 1 = ? ? ? ? = ?? ? (b) ? 2? = ? ?? ? = ?? ? (c) ? ?3 = ??? ? ? ? ? If ? ? = 2? + 1, solve ? ?2= 51 B 2?2+ 1 = 51 ? = 5 ?
[AQA Worksheet] ? ? = 2?3 250. Work out ? when ? ? = 0 [AQA Set 2] The function ?(?) is defined as 5 1 ? ? = ?2 4 0 ? < 3 3 ? 5 ??? ??? = ? 14 3? ? ? = ? (a) Work out the value of ? 1 ? ? = ?? ? = ? [AQA Worksheet] ? ? = ?2+ ?? 8. If ? 3 = 13, determine the value of ?. ? 2 ? ? ?? ? = ?? ? = ? (b) Work out the value of ? 4 = ? (b) Solve ? ? = 0 ? [AQA Worksheet] ? ? = ?2+ 3? 10 Show that ? ? + 2 = ? ? + 7 ?? ? = ? ? = ? (only 2 within domain) ? 3 ?? ?? = ? ? =?? ?= ?? ? (which is in ? ? + ? = ? + ??+ ? ? + ? ?? = ??+ ?? + ? + ?? + ? ?? = ??+ ?? = ? ? + ? ? domain) 6 If ? ? = 2? 1 determine: (a) ? 2? = ?? ? (b) ? ?2= ??? ? (c) ? 2? 1 = ? ?? ? ? = ?? ? (d) ? 1 + 2? ? 1 = ? ?? ? = ?? ?? (e) Solve ? ? + 1 + ? ? 1 = 0 ? ? ? [June 2012 Paper 2] ? ? = 3? 5 for all values of ?. Solve ? ?2= 43 ? 4 ??? ? = ?? ? = ? ? ? ? ? + ? ? + ? ? ? ? = ? ?? ? = ? ? = ?
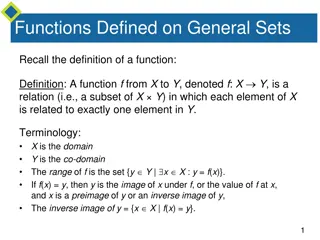
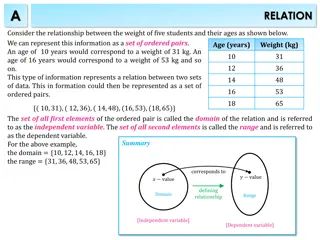
Domain & Range Inputs Outputs 1 -1 0 0 2.89 1.7 ? ? = ?? 4 2 3.1 9.61 ... ... The domain of a function is the set of possible inputs. The range of a function is the set of possible outputs.
Example ? ? ? = ?2 Sketch: ? for all ? Domain: ? The domain is the set of x-values on the graph. We can use any real number as the input here. Range: ? ? 0 ? Look at the ? values on the graph. The output has to be positive, since it s been squared. Pro Tip: Note that the domain is in terms of ? and the range in terms of ? ? .
Test Your Understanding ? Sketch: ? ? = ? ? ? ? 0 ? Domain: Presuming the output has to be a real number, we can t input negative numbers into our function. Range: ? ? 0 ? The output, again, can only be positive.
Exercise Work out the domain and range of each function. A sketch may help! ? ? =1 ? ? = 2? 1 2 3 ? ? = 2? ? For all ? For all ? except 0 For all ? Domain Domain Domain ? ? ? For all ? ? except 0 For all ?(?) Range Range Range ? ? > 0 ? ? = ?3+ 1 4 5 6 ? ? = sin? ? ? = 2cos? For all ? For all ? For all ? Domain Domain Domain 2 ? ? 2 ? 1 ? ? 1 ? ? For all ?(?) Range Range Range 1 ?2+ ?2= 9 7 8 9 ? ? = 2cos ? + 1 ? ? = ? 2+ 1 For all ? except 2 ? Domain Domain Domain ? > 1 3 ? 3 2 ? ? 2 ? ? For all ? ? except 1 Range Range Range 3 ? ? 3
Range of Quadratics A common exam question is to determine the range of a quadratic. The sketch shows the function ? = ?(?) where ? ? = ?2 4? + 7. Determine the range of ?(?). ? We need the minimum point, since from the graph we can see that ? (i.e. ?(?)) can be anything greater than this. 3 ? ? ? = ? ??+ ? ? The minimum point is (?,?) thus the range is: ? ? ? (note the rather than >) An alternative way of thinking about it, once you ve completed the square, is that anything squared is at least 0. So if ? 23 is at least 0, then clearly ? 22+ 3 is at least 3.
Test Your Understanding ? The sketch shows the function ? = ?(?) where ? ? = ? + 2 ? 4 . Determine the range of ?(?). ? + 2 ? 4 = ?2 2? 8 ? = ? 12 9 ? Therefore ? ? 9 1, 9 ? The sketch shows the function ? = ?(?) where ? ? = 21 + 4? ?2. Determine the range of ?(?). 2,25 ?2 4? 21 = ? 22 4 21 = 25 ? 22 Therefore ? ? 25 ? ?
Range for Restricted Domains Some questions are a bit jammy by restricting the domain. Look out for this, because it affects the domain! ? ? = ?2+ 4? + 3, ? 1 Determine the range of ?(?). ? Notice how the domain is ? ?. ? ? = (? + ?)(? + ?) When ? = ?,? = ??+ ? + ? = ? Sketching the graph, we see that when ? = ?, the function is increasing. Therefore when ? ?, ? ? ? ? ? 3 1 1
Test Your Understanding ? ? = ?2 3, Determine the range of ?(?). ? ? = 3? 2, Determine the range of ?(?). 0 ? < 4 ? 2 ? When ? = 0,? ? = 2 When ? = 4,? ? = 10 Range: ? ? ? < ?? ? ? ? 2 When ? = 2,? ? = 1 As ? decreases from -2, ?(?) is increasing. Therefore: ? ? 1
Range of Trigonometric Functions 180 360 90 270 Suppose we restricted the domain in different ways. Determine the range in each case (or vice versa). Ignore angles below 0 or above 360. Domain Range 1 ? ? 1 ? ? For all ? (i.e. unrestricted) 180 ? 360 ? 1 ? ? 0 0 ? 180 0 ? ? 1