Understanding Universal Gravitation and Planetary Motion
Explore the principles of Newton's Law of Universal Gravitation and Kepler's Laws of Planetary Motion. Delve into the concept of gravitational attraction between celestial bodies and the validation of these laws through the conservation of angular momentum. Learn about the measurement of the gravitational constant, G, and the nature of forces in the universe as described by the inverse square law. Gain insights into the notation and implications of these fundamental laws in explaining the motions of planets and satellites.
Uploaded on Sep 27, 2024 | 0 Views
Download Presentation

Please find below an Image/Link to download the presentation.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author. Download presentation by click this link. If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.
E N D
Presentation Transcript
Chapter 13 Universal Gravitation
Planetary Motion A large amount of data had been collected by 1687. There was no clear understanding of the forces related to these motions. Isaac Newton provided the answer. Newton s First Law A net force had to be acting on the Moon because the Moon does not move in a straight line. Newton reasoned the force was the gravitational attraction between the Earth and the Moon. Newton recognized this attraction was a special case of a general and universal attraction between objects. Introduction
Universal Gravitation This chapter emphasizes a description of planetary motion. This motion is an important test of the law s validity. Kepler s Laws of Planetary Motion These laws follow from the law of universal gravitation and the principle of conservation of angular momentum. Also derive a general expression for the gravitational potential energy of a system Look at the energy of planetary and satellite motion. Introduction
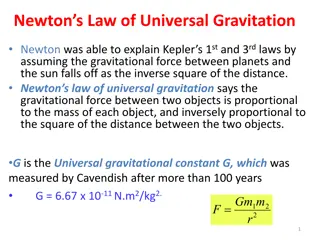
Newtons Law of Universal Gravitation Every particle in the Universe attracts every other particle with a force that is directly proportional to the product of their masses and inversely proportional to the distance between them. m m = F G 1 r 2 g 2 G is the universal gravitational constant and equals 6.673 x 10-11 N m2 / kg2. Section 13.1
Finding the Value of G In 1789 Henry Cavendish measured G. The two small spheres are fixed at the ends of a light horizontal rod. Two large masses were placed near the small ones. The angle of rotation was measured by the deflection of a light beam reflected from a mirror attached to the vertical suspension. Section 13.1
Law of Gravitation, cont This is an example of an inverse square law. The magnitude of the force varies as the inverse square of the separation of the particles. The law can also be expressed in vector form m m = 12 r G F 1 r 2 12 2 The negative sign indicates an attractive force. Section 13.1
Notation F is the force exerted by particle 1 on particle 2. 12 The negative sign in the vector form of the equation indicates that particle 2 is attracted toward particle 1. F is the force exerted by particle 2 on particle 1. 21 = F F 12 21 The forces form a Newton s Third Law action-reaction pair. Section 13.1
More About Forces Gravitation is a field force that always exists between two particles, regardless of the medium between them. The force decreases rapidly as distance increases. A consequence of the inverse square law. Section 13.1
Gravitational Force Due to a Distribution of Mass The gravitational force exerted by a finite-size, spherically symmetric mass distribution on a particle outside the distribution is the same as if the entire mass of the distribution were concentrated at the center. For example, the force exerted by the Earth on a particle of mass m near the surface of the Earth is M m R = F G E g 2 E Section 13.1
G vs. g Always distinguish between G and g. G is the universal gravitational constant. It is the same everywhere. g is the acceleration due to gravity. g = 9.80 m/s2 at the surface of the Earth. g will vary by location. Section 13.1
Finding g from G The magnitude of the force acting on an object of mass m in freefall near the Earth s surface is mg. This can be set equal to the force of universal gravitation acting on the object. M m R M R = = mg G g G E E 2 2 E E If an object is some distance habove the Earth s surface, r becomes RE + h. GM g R h + = E ( ) 2 E This shows that g decreases with increasing altitude. As r , the weight of the object approaches zero. Section 13.2
Variation of g with Height Section 13.2
Johannes Kepler 1571 1630 German astronomer Best known for developing laws of planetary motion Based on the observations of Tycho Brahe Section 13.3
Keplers Laws Kepler s First Law All planets move in elliptical orbits with the Sun at one focus. Kepler s Second Law The radius vector drawn from the Sun to a planet sweeps out equal areas in equal time intervals. Kepler s Third Law The square of the orbital period of any planet is proportional to the cube of the semimajor axis of the elliptical orbit. Section 13.3
Keplers First Law All planets move in elliptical orbits with the Sun at one focus. Any object bound to another by an inverse square law will move in an elliptical path Second focus is empty Section 7.6
Keplers Second Law A line drawn from the Sun to any planet will sweep out equal areas in equal times Area from A to B and C to D are the same The planet moves more slowly when farther from the Sun (A to B) The planet moves more quickly when closest to the Sun (C to D) Section 7.6
Keplers Third Law The square of the orbital period of any planet is proportional to cube of the average distance from the Sun to the planet. T is the period, the time required for one revolution T2 = K a3 For orbit around the Sun, K = KS = 2.97x10-19 s2/m3 K is independent of the mass of the planet Section 7.6
Keplers Third Law, cont Can be used to find the mass of the Sun or a planet When the period is measured in Earth years and the semi-major axis is in AU, Kepler s Third Law has a simpler form T2 = a3 Section 7.6
Example, Mass of the Sun Using the distance between the Earth and the Sun, and the period of the Earth s orbit, Kepler s Third Law can be used to find the mass of the Sun. 2 3 4 GT r = M Sun 2 Similarly, the mass of any object being orbited can be found if you know information about objects orbiting it. Section 13.3
Example, Geosynchronous Satellite A geosynchronous satellite appears to remain over the same point on the Earth. The gravitational force supplies a centripetal force. Consider the satellite as a particle under a net force and a particle in uniform circular motion. You can find h or v. Section 13.3