Understanding Orbital Motion and Circular Velocity in Physics
Explore the concept of orbital motion and circular velocity in physics, where we delve into the relationship between velocity and radius in circular motion with a constant centripetal force. Through Newton's 2nd Law and gravitational forces, we uncover the dependence of satellite speed on the radius and mass of the object being orbited, leading to insights into orbital velocity and the period of satellites in their orbits.
Download Presentation

Please find below an Image/Link to download the presentation.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author. Download presentation by click this link. If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.
E N D
Presentation Transcript
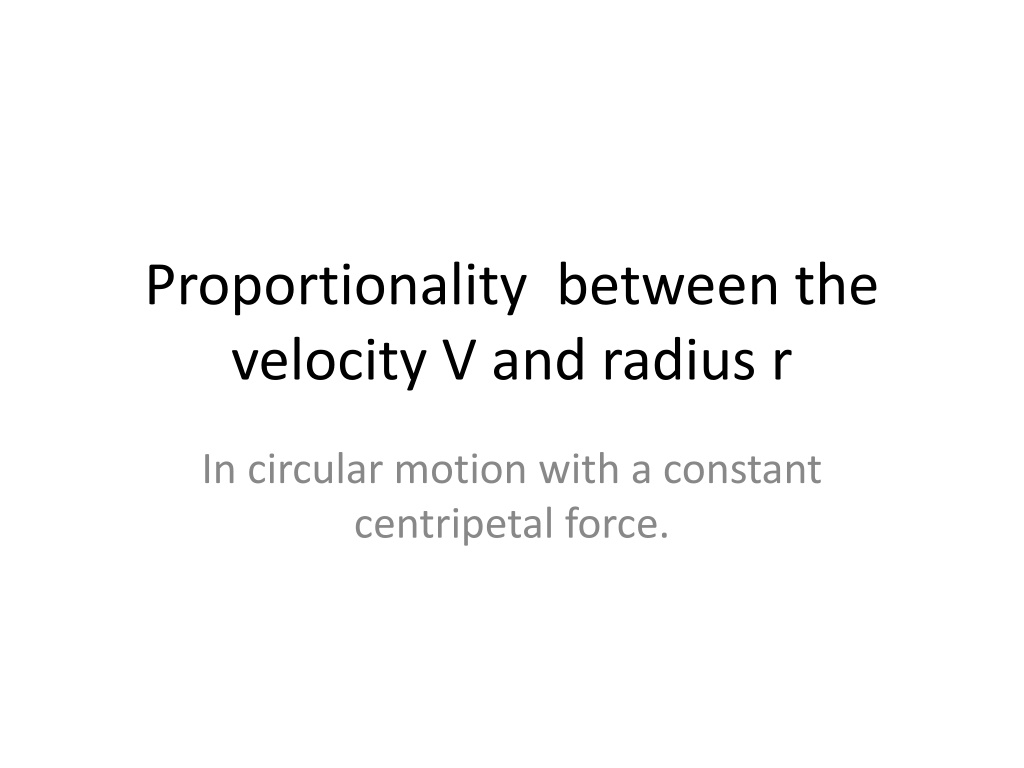
Proportionality between the velocity V and radius r In circular motion with a constant centripetal force.
Applying Newtons 2ndLaw ? = ? ? In this case the only force on the body is the force of gravity and the acceleration of the body is centripetal. ??= ??
A centripetal force is required for circular motion. This is given by the equation: 2 v Fc= ( ) m 1 r The centripetal force is supplied by the force of gravity between the two bodies which is given by the equation: Gm m FG= 1 2 2 r
Set the two forces equal to one another. F F = m1 = mass of the satellite m2 = mass of object being orbited V = velocity of the satellite G = universal gravitational constant r = radius of the circular motion C G Gm m 2 v 1 2 = m 1 2 r r Gm m 2 v 1 2 = m 1 2 r r Mass of the satellite cancels out. The speed of the satellite depends on radius r and mass m of the object being orbited.
Orbital Velocity Gm Solving for v v = r Where: v = orbital velocity (m/s) G = universal gravitational constant = 6.67 x 10-9 m = mass of the object being orbited (kg) r = radius of circular motion (m)
Period of Satellite x x We know that = = or v t t v For a circular motion, the distance x travelled by the satellite in one revolution is the circumference of the circle. That would be = 2 x r
Solving for t 2 x r t = = t or v v Gm But we also know that v = r 2 r = t This gives us Gm r
2 2 r Gm r 4 2 = t Squaring both sides We end up with orbital period t of a satellite. This is the time it takes for a satellite to complete one orbit. 3 r = 2 t Gm Again, m here is the mass of the object being orbited NOT the mass of the satellite.
Quick Check # 1: What is orbital velocity of the earth around the sun? The sun has a mass of 1.99 x 1030kg, the mean distance from the earth to the sun is 1.50 x 1011 m.
Quick Check #1 Solution 2 N.m 11 30 . 6 67 10 x (1.99 x 10 ) kg 2 kg Gm = = v 11 r 1.50 x 10 m 2 m m = = 8 4 . 8 85 10 . 2 97 x 10 v x 2 s s
Quick Check #2 A satellite is in a low earth orbit, some 250 km above the earth's surface. rearth is 6.37 x 106 m and mearth = 5.98 x 1024 kg. Find the period of the satellite in minutes.
Quick Check #2 Solution r is the distance from the center of the earth to the satellite; thus r = rearth + 250 km = 6.37 x 106 m + 250 x 103 m ( ) 3 6 6.62 10 x m 3 r = = 2 2 T 2 ( ) kg m s Gm m kg 11 24 6.67 10 5.98 10 x x kg 2 2 1min 60 = = 5357 89.3min T s s