Understanding Miller Indices in Crystallography
Miller indices, devised by British mineralogist William Hallowes Miller in 1839, provide a method to describe the orientation of planes and directions within a crystal lattice. These indices, represented by a group of three numbers, help in determining the arrangement of atoms in a crystal structure. By understanding Miller indices, one can visualize the lattice structure, planes, and atom unit cells more clearly. This comprehensive guide explains the significance, calculation, and application of Miller indices in crystallography.
Download Presentation

Please find below an Image/Link to download the presentation.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author. If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.
You are allowed to download the files provided on this website for personal or commercial use, subject to the condition that they are used lawfully. All files are the property of their respective owners.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author.
E N D
Presentation Transcript
MILLER INDICES BY : CODY, DAVID, AND SHANE
INVENTOR OF MILLER INDICES THIS SCHEME, DEVISED BY BRITISH MINERALOGIST AND CRYSTALLOGRAPHER WILLIAM HALLOWES MILLER, IN 1839, HAS THE ADVANTAGE OF ELIMINATING ALL FRACTIONS FROM THE NOTATION FOR A PLANE.
DEFINITION OF MILLER INDICES MILLER INDICES - GROUP OF THREE NUMBERS THAT INDICATES THE ORIENTATION OF A PLANE OR SET OF PARALLEL PLANES OF ATOMS IN A CRYSTAL. MILLER INDICES - (A METHOD OF DESCRIBING PLANES AND DIRECTIONS WITHIN A CRYSTAL) HTTP://WWW.YOUTUBE.COM/WATCH?V=PMTA_WIY784
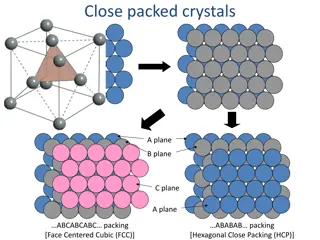
A BETTER SENSE OF THINGS IF EACH ATOM IN THE CRYSTAL IS REPRESENTED BY A POINT AND THESE POINTS ARE CONNECTED BY LINES, THE RESULTING LATTICE MAY BE DIVIDED INTO A NUMBER OF IDENTICAL BLOCKS, OR UNIT CELLS. THE INTERSECTING EDGES OF ONE OF THE UNIT CELLS DEFINES A SET OF CRYSTALLOGRAPHIC AXES, AND THE MILLER INDICES ARE DETERMINED BY THE INTERSECTION OF THE PLANE WITH THESE AXES. THE RECIPROCALS OF THESE INTERCEPTS ARE COMPUTED, AND FRACTIONS ARE CLEARED TO GIVE THE THREE MILLER INDICES (HKL).
PICTURES OF PLANES, AND DIFFERENT ATOM UNIT CELLS
CONCLUSION IF A MILLER INDEX IS ZERO, THE PLANE IS PARALLEL TO THAT AXIS. THE SMALLER A MILLER INDEX, THE MORE NEARLY PARALLEL THE PLANE IS TO THE AXIS. THE LARGER A MILLER INDEX, THE MORE NEARLY PERPENDICULAR A PLANE IS TO THAT AXIS. MULTIPLYING OR DIVIDING A MILLER INDEX BY A CONSTANT HAS NO EFFECT ON THE ORIENTATION OF THE PLANE MILLER INDICES ARE ALMOST ALWAYS SMALL.
CITED HTTP://WWW.BRITANNICA.COM/EBCHECKED/TOPIC/382843/MILLER-INDICES CHEMISTRY.BD.PSU.EDU HTTP://CNX.ORG/CONTENT/M16927/LATEST/