Understanding Joule-Thomson Coefficient in Thermodynamics
The Joule-Thomson coefficient describes the relationship between temperature and pressure changes in a substance. This coefficient impacts the direction of temperature change when pressure is altered. With equations and explanations provided by U. Nithya, M.Sc., M.Phil., this summary covers the definition, calculations, behavior of the coefficient for ideal and real gases, inversion temperature, and its relation to the van der Waals constant. The derivation of relevant equations and key concepts are explored to deepen the understanding of this critical aspect of thermodynamics.
- Joule-Thomson Coefficient
- Thermodynamics
- Pressure-Temperature Relationship
- Ideal Gas Behavior
- Real Gas Behavior
Download Presentation

Please find below an Image/Link to download the presentation.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author. Download presentation by click this link. If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.
E N D
Presentation Transcript
JOULE-THOMSON CO-EFFICIENT Name of the instructor:U.Nithya M.Sc.,M.Phil.,
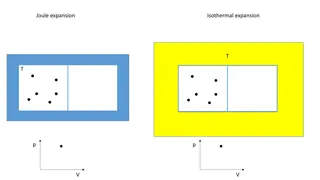
Joule Thomson co-efficient: Definition: The relationship between the fall in temperature T and fall of pressure P is called the joule thomson coefficient. It is denoted as . we know H =f(T,P) H is the state function and dH is a complete differential dH = ( H/ T)pdT + ( H/ P)Tdp --------------------- 1 ( H/ T)P= CP--------------------------------------- dH= CpdT + ( H/ P)TdP Joule Thomson expansion dH=0 2
CPdT + (H/P)TdP = 0 (or) CPdT = - ( H/ P)TdP dT/dP = - ( H/ P)T/CP ie, ( T/ P)H= - ( H/ P)T/CP ( T/ P)His called joule-Thomson coefficient and it is denoted By JT. T = -( H/ P)T/CP. P For ideal gas : ( T/ P)H= 0 For real gas : ( T/ P)H= positive or negative The pressure is decreased the temperature may decrease or increase
JTis positive decrease in pressure and decrease in temperature JTis negative decrease in pressure and increase in temperature The temperature at which JTchanges its sign is known as inversion temperature(Ti) above Tithen JT is negative below Tithen JTis Positive
Relation between JTand vander walls constant and Tiand vander wall s constant JT= ( T/ P)H= 1/Cp(2a/RT) Ti= 2a/Rb Derivation: vandeer wll s equation (P+an2/v2) (v-nb) =nRT For one of gas (P+a/v2) (v-b) =RT Expand the equation PV Pb + aV/V2-ab/V2= RT
Pv Pb + a/v ab/v2-RT =0. ---------------- 1 ab/v2is neglect a&b are small Equ 1 becomes PV Pb + a/v RT =0 (or) Pv =Pb-a/v +RT. ------------------------------ 2 We know PV =RT V=RT/P ------------------------------ 3 3 in 2 in the RHS only Pv = Pb a/RT/P +RT PV = Pb ap/RT +RT p Pv/p = Pb/P aP/RTP + RT/P V = b-a/RT +RT/P ------------------------- 4
Differentiate with respect to temperature at constant pressure ( v/ T)P= a/RT2+R/P ------------------- 5 Rearrange 4 RT/P = V-b + a/RT RT = P(v-b+a/RT) RT = Pv Pb + Pa/RT RT = P(v-b)+Pa/RT PT RT/PT = P(v-b)/PT +Pa/PTRT R/P = v-b /T + a/RT2-------------------- 6 6 in 5 ( v/ T)P= a/RT2+ v-b/T+a/RT2
(v/T)P=v-b/T+2a/RT2 ( v/ T)P= V/T b/T +2a/RT2 Rearrange ( v/ T)P-V/T = 2a/RT2-b/T xT. T( v/ T)P- vT/T = 2aT/RT2-bT/T T( v/ T)P- v= 2a/RT b----------------------- 7 (or) - v= 2a/RT- b-T( v/ T)P---------- ------ 8 We know. V= T( v/ T)P+ ( H/ P)T ------------------- 9 Rearrange 9. ( H/ P)T = V- T( v/ T)P--------------- 10 We know. JT= ( T/ P)H= - ( H/ P)Tx1/Cp------------------- 11 10 in 11. ( T/ P)H= - [V T( v/ T)Px1/Cp ( T/ P)H= [V+T( v/ T)Px1/Cp-------------- 12
12 in 8 ( T/ P)H= [ 2a/RT-b- T( v/ T)p T( v/ T)P] x 1/Cp JT=( T/ P)H= [2a/RT-b]x1/Cp---------------------- 13 Equation 13 Relationship between JTand vanderwall s constant Joule thomson effect is positive. If 2a/RT is greater than b Joule thomson effect is negative if 2a/RT is greater than b a,b and R are constants and sign of JT. The temperature at which JTchanges its sign is known as inversion temperature JT=0 T = Ti [2a/RTi-b] 1/Cp=0
2a/RTi- b =0 2a/RTi= b (or) Ti=2a/Rb. ----------------------- 14 Equation 14 relationship between inversion temperature and vander wall s constant Significance: (inversion temperature) Inversion temperature value of a gas as determined from its vander waals constant values gives us an idea about the temperature to which a gas must be cooled by a method other than subjecting the gas to joule thomson effect when we liquefy the gas. Significance of Joule Thomson experiment: joule thomson experiment helped to find out the fact that each gas possessed an inversion temerature above which the gas can never be cooled by subjecting it to joule-thomson effect. Thus joule Thomsons experiment enabled us to liquefy so called permanent gases like H2,He and air.