Understanding Isaac Newton's Mathematical Principles of Natural Philosophy
Dive into Isaac Newton's "Principia," where he explores the foundational principles of mechanics, motion, and geometry. Newton's work revolutionized our understanding of the physical world and laid the groundwork for modern physics.
Uploaded on Sep 19, 2024 | 0 Views
Download Presentation

Please find below an Image/Link to download the presentation.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author. Download presentation by click this link. If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.
E N D
Presentation Transcript
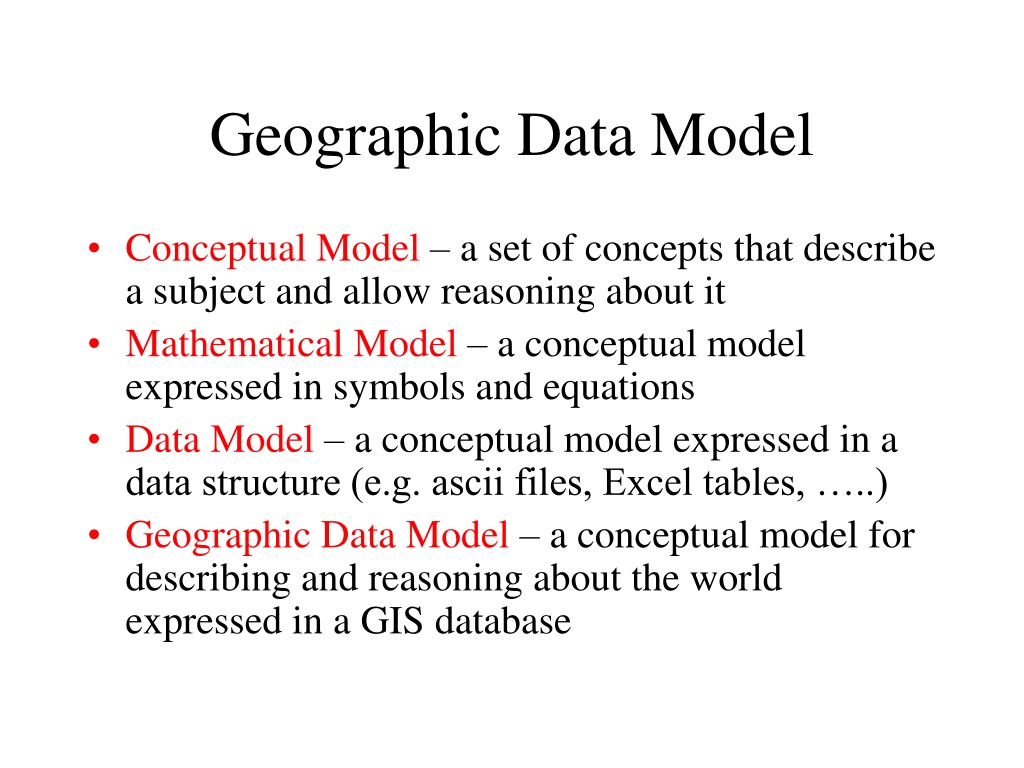
Geographic Data Model Conceptual Model a set of concepts that describe a subject and allow reasoning about it Mathematical Model a conceptual model expressed in symbols and equations Data Model a conceptual model expressed in a data structure (e.g. ascii files, Excel tables, ..) Geographic Data Model a conceptual model for describing and reasoning about the world expressed in a GIS database
Isaac Newton The Principia Mathematical Principles of Natural Philosophy Originally published in 1686, third edition 1726 Translated by I. Bernard Cohen and Anne Whitman University of California Press, 1999 Originally published as Philosophiae Naturalis Principia Mathematica In Latin
Isaac Newton Trinity College, Cambridge 5 May 1686 Introduction The ancients divided mechanics into two parts: the rational, which proceeds rigorously through demonstrations, and the practical. Practical mechanics is the subject that comprises all the manual arts, from which the subject of mechanics as a whole has adopted its name. But since those who practice the art do not generally work with a high degree of exactness, the whole subject of mechanics is distinguished from geometry by the attribution of exactness to geometry and of anything less than exactness to mechanics. Yet the errors do not come from the art but from those who practice the art.
Introduction (Cont.) Geometry postulates the solution of those problems from mechanics and teaches the use of problems thus solved. And geometry can boast that with so few principles derived from other fields it can do so much. Since the manual arts are applied especially to making bodies move, geometry is commonly used in reference to magnitude and mechanics in reference to motion In this sense rational mechanics will be the science, expressed in exact propositions and demonstrations, of the motions that result from any forces whatever, and of the forces that are required for any motions whatever
Definitions The quantity of matter is a measure of matter that arises from density and volume If the density of air is doubled in a space that is also doubled, there is four times as much air, and there is six times as much air if the space is tripled Quantity of motion is a measure of motion that arises from the velocity and the quantity of matter jointly If a body is twice as large as another and has equal velocity there is twice as much motion, and if it has twice the velocity there is four times as much motion
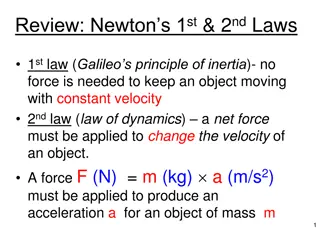
Laws Law 1 Every body perseveres in its state of being at rest or of moving uniformly straight forward except insofar as it is compelled to change its state by the forces impressed Law 2 A change in motion is proportional to the motive force impressed and takes place along the straight line in which that force is impressed Law 3 To any action there is always an opposite and equal reaction; in other words, the actions of two bodies upon each other are always equal and always opposite in direction