Understanding Forces and Mass
Forces, such as contact and non-contact forces, interact with objects to cause motion or deformation. Mass is the amount of matter in an object, measured in kilograms. Learn about applied force, normal force, frictional force, air resistance, spring force, tensile forces, compressive forces, and shearing forces. Discover how to calculate weight from mass and understand the units of force. Explore the effects of forces on objects and their surroundings.
Download Presentation

Please find below an Image/Link to download the presentation.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author. Download presentation by click this link. If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.
E N D
Presentation Transcript
Force Force
Force Force : a force forceis an interaction that causes an affected object to be pushed or pulled in a certain direction. we can not see not see the force but we can see the effect effect of force It can also change the shape or size of a body on which it acts see the Units of force: Units of force: Newtons (N) 1000 N = 1kilonewtons (kN) 1000 000 N = 1 meganewton (MN)
Mass Mass is the amount of matter contained in an object. Which can be found by using an ordinary scales. Measured in kilograms (kg) (kg) Weight Weight mass of an object in which the force of the earth exerts on its , this force is called the force of gravity. [mass (kg) x 9.81 ].Measured in Newtons (N) Example: Example: (N) A body has a mass of 100kg. What is its weight? Solution: Solution: Weight Weight = 100 x 9.81 = 981 N Therefore we need a force more than 981 N need a force more than 981 N in order to lift this 100kg body mass.
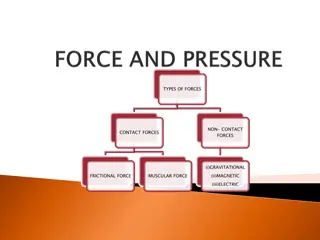
Types of force Types of force s s Basically Basically forces are of two types forces are of two types: : Contact forces Non contact forces or At a distance forces Contact Forces Contact Forces Those forces which represent the result of physical contact between two objects, where one of the object exerts force on the other. E Examples xamples of contact of contact forces: forces: Stretching of the spring of a balance pushing of a pram kicking of a football Pushing the door Hitting the ball
Types of contact Types of contact force force 1. 1. Applied force: Applied force: Force due to the action of muscles is called applied force. It is also called muscular force 2. Normal force: Normal force: It is a contact interaction force between surfaces. It always acts perpendicular to the surfaces and out of the surface 3. 3. Frictional force Frictional force Frictional force comes from interactions with a surface as an object moves or tries to move relative to the surface. 4. Air Air resistance resistance force force Force that acts in a direction opposite motion through a gas is called air resistance force. 5. Spring force Spring force Spring force comes from displacement of molecules. It is always opposite the displacement of spring. 2.
6. 6. Tensile forces Tensile forces force applied which tends to increase the length of an object 7. 7. Compressive forces Compressive forces force is applied which tends to shorten the length of an object 8. 8. Shearing forces Shearing forces force is applied which causes one layer of the material tend to slide over another layer
Non Non Contact Contact F Forces orces Those forces which do not involve physical contact between the two objects but act through the space between the two. Non Non contact force contact force examples examples 1. 1. Gravitational force Gravitational force Attractive force exerting between two objects is called gravitational force. It originates with the presence of matter. 2. Electromagnetic force 2. Electromagnetic force The electromagnetic force which includes basic electric and magnetic interactions and is responsible for the binding of atoms and the structure of solids. Weak nuclear force Strong nuclear force
Effects of forces on Engineering materials: Effects of forces on Engineering materials: Tension Tension - material will stretch Amount of stretch depends upon : Amount of stretch depends upon : the cross section area type of metal Size of force applied Compression Compression material shortens, caused buckling and breaking if material is thin and long. Bending Bending bend under the action of forces applied to them. Amount of bending depending on the size of force and the dimension of the beam. Notching Notching done to make bending easier on beams. Twisting Twisting force that caused rotation. Example: Example: A spanner used to rotate the nut.
Stress amount of force a material can withstand. The thickness or the cross sectional area of the material will mostly determined its stress contend. Stress (N/m ) = force carried by the bar (N) cross sectional area (m ) Ultimate stress - maximum stress that a material can withstand
ULTIMATE STRESSES FOR METALS IN TENSION ULTIMATE STRESSES FOR METALS IN TENSION METAL METAL ULTIMATE STRESS ULTIMATE STRESS (MN / m ) 110 400 (MN / m ) Cast Iron Mild Steel Aluminum Brass Copper 80 410 200
Example: Example: A short bar in compression has a cross-sectional area of 20 mm and it carries a force of 10 000 N Find the stress of the bar. Solution: Solution: Stress (N/m ) = force carried by the bar (N) cross sectional area (m ) Cross - Sectional Area = 20 mm = (20 mm / 1000 000 mm /m ) Stress Stress = 10 000 N (20 / 1000 000) m = (10 000 N X 1000 000 m ) 20 = 500 000 000 N/m = 500 000 000 N/m 1000 000 N/MN = 500 MN / m
Strain Strain measured the amount of distortion an object undergoes when force is applied.(h have no units) ave no units) Strain = Alteration in length / Original length Strain = Alteration in length / Original length Example: Example: A bar metal 2500 mm long has a tensile force applied to it. Under the A bar metal 2500 mm long has a tensile force applied to it. Under the action of the force its length increases by 0.75 mm. action of the force its length increases by 0.75 mm. What is the strain? What is the strain? Solution: Solution: Strain = AL / OL Strain = AL / OL = 0.75 = 0.75 mm mm / 2500 / 2500 mm mm = = 0.0003 0.0003
Example: Example: A wire 3m long and having a cross-sectional area of 0.08mm2 has a force of 80N applied to it. Under the action of this force it stretches to 3.09m. Calculate the stress stress in the wire, and also find the strain. strain. Solution: Solution: Stress Stress = fcb (N) = fcb (N) cross = 80 N (0.08 = (80N = (80N x x 1 000 000 m = 1 000 = 1 000 000 000 N = = 1000 MNm 1000 MNm2 2 Strain = Strain = AL AL / OL / OL = = 0.09 m /3m 0.09 m /3m = = 0.03 0.03 cross- -sectional (m sectional (m2 2) ) 0.08mm mm2 2/1 000 000 /1 000 000mm 1 000 000 m2 2) ) 0.08 000 000 N/m /m2 2 / / 1 1 000 000 mm2 2/m /m2 2) ) 0.08 000 000 N N/MN /MN
Force as a Vector Force as a Vector Q Quantity uantity A vector is a quantity that possessors both size size (amount of force) and direction direction (angle). Force which acts on a body in a particular direction Vertical Force Vertical Force Resultant force Resultant force Horizontal force Horizontal force Two forces acting on the object if the object is free to move thus will move in the direction of the resultant force resultant force
5N 6N 1. Resultant Force = 5 N + 6N = 11 N 10N 6N 2. Resultant Force = 10N 6N = 4N
Representation of Forces Representation of Forces representing of forces graphically Key Factors: Key Factors: Magnitude (size) Direction (angle) Point of application Sense (away /towards) the point of application Procedures in representing forces: Procedures in representing forces: Faintly draw a horizontal line and mark point of application. From the point of application draw a faint line with the direction. Chose a suitable scale. Show its sense.
Therefore: Therefore: Magnitude will be represented by a length Arrow represents the sense Angle at which the line inclined represents the direction Example: Example: A force of 15 N pushes towards point X and it acts in a direction which is 45 to the vertical. Represent this force graphically. . Solution: Solution: Scale : 1cm = 5N 45 X 3cm
Moments of force Moments of force force that have turning effect (rotation) Fulcrum Fulcrum point about which the object turns Clockwise moments Clockwise moments rotates clock wise direction distance from the fulcrum Clockwise moments = Force (N) x Distance ( Clockwise moments = Force (N) x Distance (mm,cm,m mm,cm,m) ) Units of moments Units of moments depend on the units of force and the units of distance Anti Anti- - clockwise moments clockwise moments rotates anticlockwise direction distance from the fulcrum Anti Anti- -clockwise moments = Force (N) X Distance (mm, cm, m) clockwise moments = Force (N) X Distance (mm, cm, m)
Example 1: A tommy bar is used to operate a box spanner. What turning moment do the forces produce? 60N 100mm 100mm 60N Solution: Turning Moment = Clockwise Moment = ( F X D ) + ( F X D ) = (60N X 100mm) + (60N X 100mm) = (600Nmm) + (600Nmm) = 12000Nmm
PRINCIPLE OF MOMENTS Principle of Moments the sum of the clockwise moments is equal to the sum of anti-clockwise direction Clockwise Direction = Anti-clockwise Direction (Force x Distance) = (Force X Distance)
Example 2: 1. Find the force F required to balance the lever shown: 30mm 60mm F 50N Solution: For Balance the anti-clockwise moments must equal to the clockwise Clockwise Moment = Anti-Clockwise Moment F X D = F X D F X 30mm = 50N X 60mm F30mm = 3 000 Nmm F = 3 000 Nmm / 30mm F = 100N
Example 3: Find the Force of R? R 60N 40mm 120mm Solution: Clockwise Moments = Anti-Clockwise Moments F X D = F X D 60N X 120 = R X 40mm 720Nmm = R40mm 720Nmm / 40mm = R 180N = R
Reaction at the Fulcrum Example 1: In this diagram the Fulcrum (Pin) prevents it to fall to the ground. Therefore the fulcrum pin holds the total load. Fulcrum Pin 4m 2m 1000N 2000N Solution: Total Upward Force = Total Downward Force Fulcrum Pin = 1000N + 2000N Fulcrum Pin = 3000N
Example 2: Calculate the Force on the Spring and the force on the pivot when a load of 200N is applied as shown. 200N F (spring) 20cm 2cm Pivot R Solution: F = force on the spring Clockwise moment about the Pivot = Anticlockwise moment about the Pivot F X D = F X D F X 2cm = 200N X (20cm +2cm) F2cm = 4400Ncm F = 4400N cm/2 cm F = 2200N
Cont. R =Force on the Pivot Total Upward Force = Total Downward Force 2200N = 200N + R 2200N 200N = R 2000N = R
Centre of Gravity Centre of Gravity thecentre of gravity of a body is the point at which all the mass of the body may be assumed to concentrated. Balance and Unbalance A body will only balanced if it is supported at its centre.
Example 1: A uniform bar of metal 4 meters long and weighing 180 newtons is supported at 1 metre from one end. Find the force needed to balance the bar if this force is applied at 0.5 metre from the same end as shown. Solution: F 180N will be concentrated on the centre 0.5m 1m 2m 1m 180N F 0.5m 1m
Cont. Taking the moments at the point which it was supported. Clockwise moment = Anticlockwise moment F X D = F X D F X 0.5m = 180N X 1m F0.5m = 180Nm F = 180Nm / 0.5m F = 360N