Understanding Divisibility Rules for Quick Math Solutions
Learn about various rules of divisibility such as checking if a number is divisible by 2, 3, 4, 5, 7, 8, 9, 11, and finding factors. Explore examples like forming multiples of 4 with digits 2, 4, 6, and 8, determining the number of zeros in factorials, and finding the greatest number dividing six consecutive numbers. Visual aids are provided for better understanding.
Download Presentation

Please find below an Image/Link to download the presentation.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author. Download presentation by click this link. If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.
E N D
Presentation Transcript
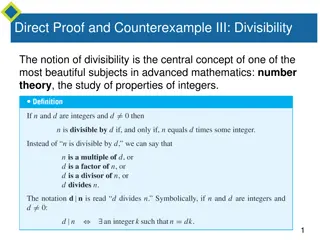
Divisibility Rules # RULE 2 The number has a units digit 0, 2, 4, 6 or 8. 3 The sum of the digits is a multiple of 3. 4 The number formed by the last two digits is divisible by 4. 5 The number has a units digit 0 or 5. 6 The number is even, and its digits sum to a multiple of 3. The result of subtracting twice the units digit from the number formed by the remaining digits is divisible by 7. 7 8 The number formed by the last three digits is divisible by 8. 9 The sum of the digits is a multiple of 9. 10 The number has a units digit 0. 11 The alternating addition and subtraction of the digits is a number divisible by 11.
Divisibility Rules Using each of the four digits 2, 4, 6 and 8 exactly once, how many four- digit multiples of 4 can be formed? 24, 28, 48, 64,68, 84 (6 two-digit numbers) 24 6824 & 8624 6 2 = 12 four-digit multiples of 4 2468 2684 4264 6248 6428 8264 2648 2864 4628 6284 6824 8624
Divisibility Rules How many zeros are there after the last nonzero digit of 125!? 125! = 125 124 123 3 2 1 125, 120, 115, 110, , 15, 10, 5 125 = 5 25 5 = 5 1 25 5s 125, 100, 75, 50, 25 25, 20, 15, 10, 5 5 5, 5 4, 5 3, 5 2, 5 1 6 5s 25 + 6 = 31 zeros
Divisibility Rules What is the greatest number that evenly divides the sum of any six consecutive whole numbers? {n, n + 1, n + 2, n + 3, n + 4, n + 5} n + n + 1 + n + 2 + n + 3 + n + 4 + n + 5 6n + 15 3(2n + 5) -1, -2, 0, 1, 2, 3 -1 + (-2) + 0 + 1 + 2 + 3 = 3