Thermal Radiation and Planck's Postulate: Fundamental Concepts of Quantum Physics
Thermal radiation is emitted by bodies due to temperature, with blackbodies absorbing all incident thermal radiation. Spectral radiancy of blackbody radiation indicates varying power with temperature and frequency. Stefan's law, Wien's displacement law, and classical theories like Rayleigh and Jeans contribute to understanding cavity radiation. Three-dimensional cavity analysis showcases the volume of allowed electromagnetic standing waves.
Download Presentation

Please find below an Image/Link to download the presentation.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author. Download presentation by click this link. If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.
E N D
Presentation Transcript
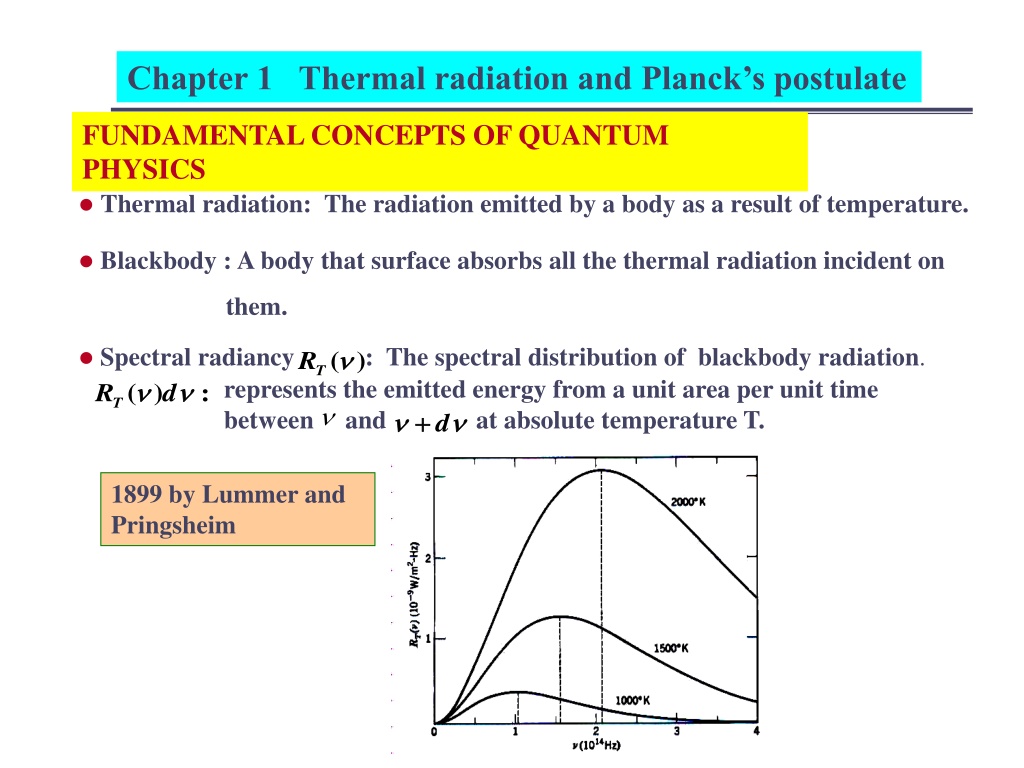
Chapter 1 Thermal radiation and Plancks postulate FUNDAMENTAL CONCEPTS OF QUANTUM PHYSICS Thermal radiation: The radiation emitted by a body as a result of temperature. Blackbody : A body that surface absorbs all the thermal radiation incident on them. Spectral radiancy : The spectral distribution of blackbody radiation. ) ( T R : ) ( d RT represents the emitted energy from a unit area per unit time between and at absolute temperature T. d + + 1899 by Lummer and Pringsheim
Chapter 1 Thermal radiation and Plancks postulate The spectral radiancy of blackbody radiation shows that: (1) little power radiation at very low frequency (2) the power radiation increases rapidly as increases from very small value. (3) the power radiation is most intense at certain for particular max temperature. (4) drops slowly, but continuously as increases ) ( , max T R , and ( T R ) . 0 (5) increases linearly with increasing temperature. max 0 = = d ( R R ) (6) the total radiation for all ( radiancy ) T T increases less rapidly than linearly with increasing temperature.
Chapter 1 Thermal radiation and Plancks postulate = = , = = o 4 8 2 4 R T W m K . 5 67 10 / Stefan s law (1879): T Stefan-Boltzmann constant T Wien s displacement (1894): max 1.3 Classical theory of cavity radiation Rayleigh and Jeans (1900): (1) standing wave with nodes at the metallic surface (2) geometrical arguments count the number of standing waves (3) average total energy depends only on the temperature one-dimensional cavity: one-dimensional electromagnetic standing wave x 2 = = E x t E t ( , ) sin( ) sin( 2 ) 0
Chapter 1 Thermal radiation and Plancks postulate for all time t, nodes at x 2 = 2 = = = = x n n / , 3 , 2 , 1 , 0 ....... = 0 = = = / 2 / / 2 x a a n a n nc a standing wave ( d N ) : the number of allowed standing wave between and +d c a dn d N / 4 ( 2 ) ( = = = = = = = = d ) n a c dn a c ( 2 / ) ( 2 / d ) two polarization states = = )( + + d d a c ( 2 / ) d = = ) c a ( 2 / 0 n
Chapter 1 Thermal radiation and Plancks postulate for three-dimensional cavity = = c ) = = d ) r a dr a c ( 2 / ( 2 / + + r r dr the volume of concentric shell a a a 2 2 2 4 = = 4 d ) = = 4 d 2 2 2 3 2 r dr v ( ) ( ( ) c 1 c c c c 3 a 3 V 3 8 8 ( d ) = = 4 = = d = = d 2 2 2 N r dr 2 8 The number of allowed electromagnetic standing wave in 3D Proof: ( x propagation direction = = ) 2 / cos / 2 /2 = = ( ) 2 / cos / 2 y = = ( E ) 2 / x cos = = / 2 z nodal planes 2 t E x t ( , ) sin( / ) sin( 2 ) x x 0 = = 2 E y t E y t ( , ) sin( / ) sin( 2 ) /2 y y 0 = = 2 E z t E z t ( , ) sin( / ) sin( 2 ) z z 0
Chapter 1 Thermal radiation and Plancks postulate for nodes: = = = = 3 , 2 , 1 = = x 2 , a x n n , 0 / , ..... x x x = = = = 3 , 2 , 1 = = y 2 , a y n n , 0 / , n ..... y y y = = = = 3 , 2 , 1 = = z 2 , a z n , 0 / , ..... z z z = = = = = = a n a n a n ( 2 / ) cos ( , x 2 / ) cos ( , y = = 2 / ) cos z + + + + + + + + 2 2 2 2 2 x 2 y 2 z a n n n ( 2 / ) (cos cos cos ) = = + + + + 2 x 2 y 2 z a n n n 2 / = = / = = + + + + = = 2 2 2 c c a n n n c a r ( / 2 ) ( / 2 ) x y z = = + + + + = = c ) = = d ) 2 2 2 r n n n a dr a c ( 2 / ( 2 / x y z = = / = = c / = = ( c / 2 2 N r dr ) r 2 dr 2 )( r ) dr N d ( 1 ( 4 ) 8 / = = / 2 ( ) ( considering two polarization state d ) ( d = = 4 d 3 2 3 2 N a a ) ( ( = = d ) ) = = 4 : d 3) 2 N N V c / 2 / 1 ( / 2c 3 8 Density of states per unit volume per unit frequency
Chapter 1 Thermal radiation and Plancks postulate the law of equipartition energy: For a system of gas molecules in thermal equilibrium at temperature T, the average kinetic energy of a molecules per degree of freedom is kT/2, is Boltzmann constant. K joule k 10 38 . 1 = = o/ 23 average total energy of each standing wave : = = = = KT KT 2 / 2 the energy density between and +d : kTd c 2 8 Rayleigh-Jeans blackbody radiation = = d ( ) T 3 ultraviolet catastrophe
Chapter 1 Thermal radiation and Plancks postulate 1.4 Planck s theory of cavity radiation , kT 0 = = , Planck s assumption: and T ( ) 0 the origin of equipartition of energy: ( = = kT/ / Boltzmann distribution d P probability of finding a system with energy between and +d ) ( 0 P e kT ) ( ) : ( d ) P = = 0 P d kT / e 1 | 0 ( d ) = = d = = ( = = kT / P kT e ) 1 kT kT 0 0 kT / e ( d ) = = d P kT 0 0 1 | 0 = = [ ( ( = = ] kT kT / / kT e kT e kT ) ) kT 0 = = kT
Chapter 1 Thermal radiation and Plancks postulate = = .......... Planck s assumption: , 0 2 , 3 , 4 , .... , kT kT (1) small kT 0 0 (2) large large . 6 10 = = h , kT kT 34 = = h joul s 63 Planck constant Using Planck s discrete energy to find = = = = nh = = n , 3 , 2 , 1 , 0 = = ...... , kT kT nh = = ( nh kT n / p e n e ) kT 1 = = = = = = n n = = n 0 0 0 kT = = = = ( nh kT n / P e e ) kT n n n 0 0 0 = = h kT /
Chapter 1 Thermal radiation and Plancks postulate d d = = n = = n = = n n n n e e n e d d d = = n = = = = = = n 0 0 0 e ln d = = n = = n = = n n n n 0 e e e 0 = = ] 0 0 d d = = n = = n = = [ n n kT e h e ln ln d d 0 0 n n 2 3 = = + + + + + + + + e e e e 1 ..... = = 0 = = = = X e + + + + + + + + = = d = = 2 3 1 1 X X X X e 1 ....... 1 ( ) 1 ( ) d = = = = [ 1 h e h e ln( 1 ) ( ) ln( 1 )] d d h h 1 e = = = = = = h e ( ) h kT / e e 1 1 1 h h + + h kT / kT e kT kT 1 h / h h kT / kT e 1 0
Chapter 1 Thermal radiation and Plancks postulate 2 h 8 ( = = ) energy density between and +d : T h kT 3 / c e 1 ( d = = ( d ) ) T T d 8 c hc 1 kT ( = = ( = = ( = = ) ) ) T T T d hc 2 5 / e 1 dV ( = = ( c R ) 4 ( / ) ) Ex: Show T T r d A dA cos r = = = = solid angle expanded by dA is 4 4 2 2 r r spectral radiancy: ) ( T R = = dA cos r ( dV dA t ) ( ) /( ) T 4 2 dA cos r 2 c t / 2 = = d d ( 2 2 r dr ) sin T 4 2 t 0 0 0 c = = ( ) T 4
Chapter 1 Thermal radiation and Plancks postulate Ex: Use the relation between spectral radiancy c d R T / 4 ( ) ( = = ) ( d ) T and energy density, together with Planck s radiation law, to derive Stefan s law = = , = = 2 4 5 4 2 3 RT T k c h / 15 3 c h 2 c = = ( d = = ( d = = d R R ) ) T T T h kT 2 / e 4 x x 1 0 0 0 4 3 kT 2 c ( ) = = h x kT / = = dx 2 3 h e 1 0 4 4 = = x 3 4 kT 2 c ( ) x e dx /( ) 1 / 15 = = = = 4 T 0 2 3 h 15 2 5 4 k = = 2 3 c h 15
Chapter 1 Thermal radiation and Plancks postulate = = x 3 1 4 x e dx ( ) 1 / 15 Ex: Show that 0 0 = = = = x x x 3 1 3 1 I x e dx x e e dx ( ) 1 1 ( ) 0 = = + + + + + + = = x x x nx 1 2 e e e e 1 ( ) 1 ..... = = n 0 1 x 4 + + = = = = = = nx n x y 3 3 ( 1 ) 3 I x e e dx x e dx y e dy + + + + n n ( ) 1 ) 1 0 0 0 = = = = = = y n + + n = = n 0 ) 1 0 0 + + = = + + = = = = n x y 3 3 ( 1 ) y n x dx dy n x e e ( /( ) 1 /( , Set 0 = = y 3 y e dy 6 by consecutive partial integration 1 n 1 1 n = = n = = n = = n 4= = ? = = = = I 6 6 + + 4 4 n ( ) 1 1 0 1 2 1 n = = x = = n = = 2 F x ( ) Fourier series expansion F : 2 6 1 4 4 1 n 1 n 1 n = = x 2 = = n = = n = = n = = + + 8 = = 4 4 F x ( ) 48 2 4 4 5 90 1 1 1
Chapter 1 Thermal radiation and Plancks postulate Ex: Derive the Wien displacement law ( ), T = = T hc k 2014 . 0 / . max max hc 8 ( = = ) T T hc kT 5 / e 1 max d ( hc hc kT / hc e ) 5 = = + + = = T d 0 0 kT hc kT / / 2 e kT e ( ) 1 x = = / x hc kT x e + + = = 1 5 Solve by plotting: find the intersection point for two functions x 5 = = = = x y y e 1 , Y 1 2 = = y x 1 / 5 intersection points: , 0 = = = = x x 1 . 4 965 X = = x y e 2 = = T hc k 2014 . 0 / max 5
Chapter 1 Thermal radiation and Plancks postulate 1.5 The use of Planck s radiation law in thermometry optical pyrometer (1) For monochromatic radiation of wave length the ratio of the spectral o o intensities emitted by sources at and is given by T K T K 1 2 T : = = o hc kT / T C 1068 e 1 standard temperature ( Au ) 1 1 melting hc kT / T : e 1 2 unknown temperature 2 o (2) blackbody radiation supports the big-bang theory. K 3
Chapter 1 Thermal radiation and Plancks postulate 1.6 Planck s Postulate and its implication Planck s postulate: Any physical entity with one degree of freedom whose coordinate is a sinusoidal function of time (i.e., simple harmonic oscillation can posses = = only total energy nh Ex: Find the discrete energy for a pendulum of mass 0.01 Kg suspended by a string 0.01 m in length and extreme position at an angle 0.1 rad. g 1 1 8 . 9 = = = = = = / 1 ( 6 . 1 sec) 2 l 2 = = 1 . 0 = = 8 . 9 1 . 0 = = 5 mgh mg J 1 ( cos ) . 0 01 1 ( cos E ) 1 . 0 5 10 ( ) 33 E 10 = = = = 6 . 1 = = = = = = 34 33 29 E h J . 6 63 10 10 ( ) 2 10 5 5 10 The discreteness in the energy is not so valid.