Rationalizing in Mathematics
There is an agreement
in mathematics
that we don’t leave a radical
in the denominator
of a fraction.
So how do we change the
denominator of a fraction?
(Without changing the value of
the fraction, of course.)
The same way we change the
denominator of any fraction!
(Without changing the value of
the fraction, of course.)
We multiply the denominator
by the same number.
and the numerator
By what number
to a rational number?
to change it
can we multiply
The answer is . . .
. . . by itself!
Remember,
is the number we square
to get
n
.
we’d better get
n
.
So when we square it,
Because we are changing
the denominator
we call this process
rationalizing
.
to a rational number,
Rationalize the denominator:
(Don’t forget to simplify)
Rationalize the denominator:
(Don’t forget to simplify)
(Don’t forget to simplify)
When there is a binomial with a
radical in the denominator of a
fraction, you find the conjugate and
multiply. This gives a rational
denominator.
Multiply by
the conjugate.
FOIL numerator
and denominator.
Next
S
i
m
p
l
i
f
y
·
=
Combine like terms
Try this on your own:
This powerpoint was kindly donated to
www.worldofteaching.com
http://www.worldofteaching.com
is home to over a
thousand powerpoints submitted by teachers. This is a
completely free site and requires no registration. Please
visit and I hope it will help in your teaching.
Discover the essential concept of rationalizing in mathematics, focusing on not leaving radicals in the denominator of a fraction. Learn how to change the denominator of a fraction without altering its value by multiplying the numerator and denominator by the same number. Explore the process of rationalizing to convert the denominator into a rational number, illustrated step-by-step with examples.
Download Presentation
Please find below an Image/Link to download the presentation.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author.If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.
You are allowed to download the files provided on this website for personal or commercial use, subject to the condition that they are used lawfully. All files are the property of their respective owners.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author.
E N D
Presentation Transcript
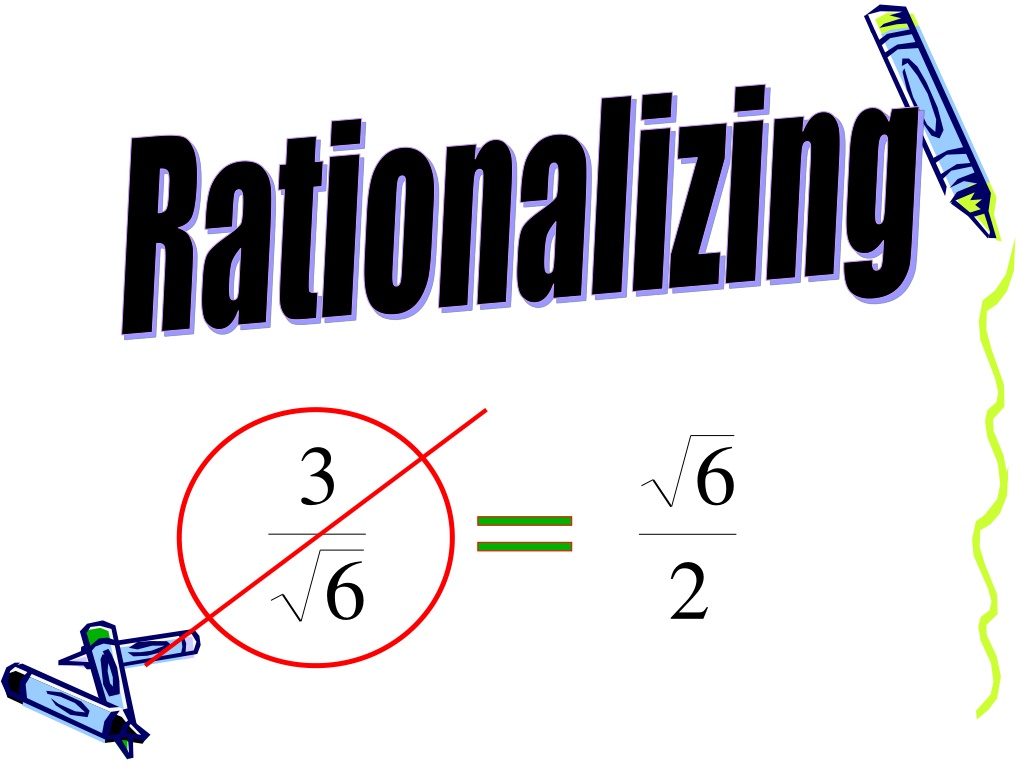
Rationalizing 3 6 6 2
There is an agreement in mathematics that we don t leave a radical in the denominator of a fraction. 1 3
So how do we change the denominator of a fraction? (Without changing the value of the fraction, of course.) 1 3
The same way we change the denominator of any fraction! (Without changing the value of the fraction, of course.) 1 3 3 1 = = 4 3 12 4
We multiply the denominator and the numerator by the same number. 1 3 3 1 = = 4 3 12 4
By what number can we multiply 3 to change it to a rational number? 1 3
The answer is . . . . . . by itself! 2 3 ( ) = = 3 3 3 1 3
Remember, ( )2 = n n n is the number we square to get n. So when we square it, we d better get n.
In our fraction, to get the radical out of the denominator, we can multiply numerator and denominator by . 3 1 3
In our fraction, to get the radical out of the denominator, we can multiply numerator and denominator by . 3 1 1 3 3 = = 3 3 3 3
Because we are changing the denominator to a rational number, we call this process rationalizing. 3 1 = 3 3
Rationalize the denominator: 2 4 2 4 2 4 = = = 2 2 2 2 2 2
Rationalize the denominator: 8 96 8 12 = = = 12 12 12 12 6 4 6 = 3 12 3
When there is a binomial with a radical in the denominator of a fraction, you find the conjugate and multiply. This gives a rational denominator. Ex: 5 +6 Conjugate: 5 6 3 2 2 Conjugate: 3+2 2 What is conjugate of 2 7+3? Answer: 2 7 3
5 + 6 5 3 Simplify: 5 +6 5 3= 5 +6 5 3 5 +3 5 +3 Multiply by the conjugate. 5+3 5 +6 5 +18 5 9 FOIL numerator and denominator. Next
2 Simplify 2 5 2 + 5 + 2 5 2 5 2 2(5) 2 2 2 5 ( 2) 2 10 2 2 23 10 2 2 25 2 =
23 +9 5 4 Combine like terms Try this on your own: 6 Answer: 3 6 2 3 3+ 2 7
This powerpoint was kindly donated to www.worldofteaching.com http://www.worldofteaching.com is home to over a thousand powerpoints submitted by teachers. This is a completely free site and requires no registration. Please visit and I hope it will help in your teaching.
