Rational Numbers and Decimals
The concept of rational numbers, decimals, and their relationship. Learn how to convert fractions to decimals, identify terminating and repeating decimals, and represent decimals as fractions. Practice various exercises to enhance your understanding of rational numbers. Dive into modeling and problem-solving scenarios to apply your knowledge effectively.
Uploaded on Mar 10, 2025 | 0 Views
Download Presentation

Please find below an Image/Link to download the presentation.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author.If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.
You are allowed to download the files provided on this website for personal or commercial use, subject to the condition that they are used lawfully. All files are the property of their respective owners.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author.
E N D
Presentation Transcript
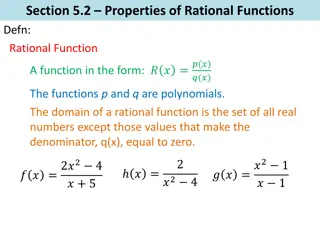
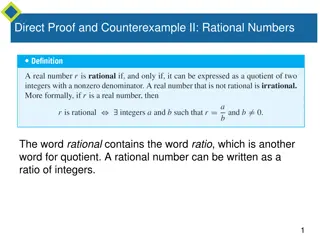
A rational number is a number that can be written as ?/? where a and b are integers and b 0.
Because you can divide any integer by any nonzero integer, you can use long division to write fractions and mixed numbers as decimals. These decimals are also rational numbers and will either terminate or repeat.
A terminating decimal is a decimal that ends. For example: 1.5, 0.25, 10.625 A repeating decimal is a decimal that has a pattern that repeats. For example: 1.333 . . . = 1. 3 0.151515 . . . = 0. 15
Modeling Write -21 4as a decimal. Write 5 11as a decimal.
Practice 1. 6 5 2. 73 8 3 11 5 27 3. 4. 1
Modeling Write 0.26 as a fraction in simplest form.
Practice Write the decimal as a fraction or a mixed number in simplest form. 5. 0.7 6. 0.125 7. 3.1 8. 10.25
Modeling A box turtle hibernates in sand at 15 spotted turtle hibernates at 116 turtle is deeper? 8feet. A 25feet. Which
Practice You lose one quarter, two dimes, and two nickels. a. Write the amount as a decimal. b. Write the amount as a fraction in simplest form.
Lesson Beginning Copy and complete the statement. a. -0.85 ___ 3 4 b. 1.25 ____11 5 2 3____. 6 c.
Commutative Property of Addition Words: In a sum, you can add terms in any order. Numbers: 5 + (-6) -6 + 5 Algebra: a + b b + a
Commutative Property of Multiplication Words: In a product, you can multiply factors in any order. Numbers: 4(-7) -7(4) Algebra: ab ba
Associative Property of Addition Words: Changing the grouping of terms will not change the sum. Numbers: (9 + 8) + 6 9 + (8 +6) Algebra: (a + b) + c a +(b + c)
Associative Property of Multiplication Words: Changing the grouping of factors will not change the product. Numbers: (2 x 3) x 4 2 x (3 x 4) Algebra: (ab)c a(bc)
Inverse Property of Addition Words: The sum of a number and its additive inverse, or opposite, is 0. Numbers: 5 + (-5) 0 Algebra: a + (-a) 0
Inverse Property of Multiplication Words: The product of a non zero number and its multiplicative inverse ,or reciprocal, is 1. Numbers: 3 4x 4 Algebra: For nonzero integers a and b, ? 3= 1 ? ?= 1 ?
Identity Property of Addition Words: The sum of a number and the additive identity, 0, is the number. Numbers: -7 + 0 = -7 Algebra: a + 0 = a
Identity Property of Multiplication Words: The product of a number and the multiplicative identity , 1, is the number. Numbers: 9 x 1 = 9 Algebra: a(1) = a
Modeling Identify the properties. a. 4 5 5 4= 1 b. -3.5 + 3.5 = 0 c. -4 + 8 = 8 + (-4) d. (-7.9)(1) = -7.9
Practice Tell which property is being illustrated. a. -3 + 0 = -3 b. 15 x 8 = 8 x 15 c. (5 + 4) + 6 = 5 + (4 + 6)
Modeling Evaluate the expression. Justify each step. -10.6 + 3 + (-4.4)
Modeling Evaluate the expression. Justify each step you take. 2 3+ 10+ ( 2 7 3)
Practice Evaluate the expression. Justify each step you take. a. -25 (7) (-4) b. 5 3 7 7 4 4
Homework Pg 304 # 1, 2, 3 37 odd