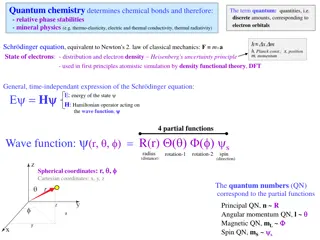
Quantum Statistics in Physical Systems

In the realm of quantum statistics, various ensembles such as the grand canonical ensemble play a crucial role in describing the behavior of systems like gases and biological molecules. Understanding concepts such as Gibbs factor, chemical potential, and the probabilities of states being occupied sheds light on phenomena like hemoglobin binding to oxygen and the dangers of carbon monoxide poisoning. The statistics of ideal gases, Boltzmann statistics, Bose statistics, and Fermi statistics further enrich our comprehension of how particles behave in different scenarios.

• Quantum Statistics
• Grand Canonical Ensemble
• Chemical Potential
• Probability
• Ideal Gas

rael Follow

Uploaded on Oct 07, 2024 | 0 Views

Download Presentation

Please find below an Image/Link to download the presentation.

The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author. Download presentation by click this link. If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.

Presentation Transcript

1. Week 11 Quantum Statistics

2. Gibbs factor/grand canonical ensemble ? ?2 ? ?1= ??2 ???= ???1 = 1 ?(?1)= ????2 ??(?1) ? 1 ????+ ???? ???? ???2 ?? ?2 ? ?1 ?? ?2 + ??(?1) ? ?1=? ? ?2 ??(?2) ? ? ?1 ??(?1) ?? ? ?2 ?? Reservoir ??, ??, ?, ? System ?, ? 1 ?? ? ? ?? ? ? ? = ?? ? = ?? ? ? ?? ? ?? ??+ ? = const, ??+ ? = const

3. Hemoglobin with O2 ? = 1 ? = 0 ? = 1 + ? (? ?)/??, ? = 0.7 eV ??int ??? 0.6 eV at 310 K 0.2 atm. ? = ??ln ? (? ?)/?? ?(0.1 ??)/?? 40 Probability of any given site being occupied by oxygen: ? =? (? ?)/?? ? 40 1+40= 98%

4. Carbon monoxide poisoning CO 1/100th of CO2 ? = 1 + ? (? ?)/??+ ? (? ? )/??, ? = 0.85 eV ? = ? ??ln100 = 0.72 eV. ? (? ? )/?? ?(0.13 ??)/?? 120 Probability of any given site being occupied by oxygen: ? =? (? ?)/?? ? 40 1+40+120= 25%

5. Chemical potential of ideal gas ??int ??3, ? = 2???? , ?? = ??? ? = ??ln ? ? ? ?,? = ? ? + ??ln Partial pressure of O2 1/5 atm Partial pressure of CO 1/5 1/100 atm ? = 0.175 10 11m ??ln ?int= ?rot=?? 0.00018 eV) from problem 6.24 ? ??3= 0.466 eV 2?= 74.2 (? =

6. Boltzmann statistics 1 ?!?1 ? ? = Putting ? identical balls without labels into ?1 (= ?) different states (with same energy) Boxes have labels they are different states. ? =1 Balls are the same, indistinguishable. 2!52= 12.5 1 2 3 4 5

7. 11000 01010 20000 10100 01001 02000 10010 00110 00200 10001 00101 00020 01100 00011 00002 Bose statistics ? = ? Putting ? identical balls without labels into ? different states, each state can be occupied with 0, 1, 2, , balls. Boxes having labels they are different states. Balls are the same, indistinguishable. ? ? + ? 1 ? = 15 = 1 2 3 4 5 ?: degeneracy

8. 11000 01010 20000 10100 01001 02000 10010 00110 00200 10001 00101 00020 01100 00011 00002 Fermi statistics ? = ? Putting ? identical balls without labels into ? different states, not more than 1 ball in each state (Pauli exclusion). Boxes having labels they are different states. Balls are the same, indistinguishable. Cannot put more than one ball is a box. ? ? ? = =10 1 2 3 4 5

9. Spin and statistics Bose particle (like photon, phonon, Higgs, 4He) wave functions must be symmetric with respect to particle permutation, the spin is 0 or integer (in units of Planck constant ) Fermi particle (like electron, p, n, 3He), wave functions must be antisymmetric with respect to particle permutation, the spin is 1/2 or half integers

10. When Boltzmann counting ? = ?!?1 1 ? is valid? When we have much more states than balls, each state has very low probability of occupation. ?1 ?. ? ??3 1 ?3= ?? ?~ ??? /? Normal gas, ? Quantum gas, ? ? ?3 ? ?3

11. Particle number distribution The probability of a particular state with energy ? is occupied by ? particles, ? = 0, 1, State ?, no particles ? ? =1 ?? ? ? ? State ?, one particle ?? grand partition function ? ? ? ? ? = etc ?? ?=0,1,

12. Fermi-Dirac distribution ? = 0, and 1 only ? 0 =1 ?(1) =1 ?? ? ? State ?, no particles ?, ?? ? = 1 + ? ? ? State ?, one particle ?? ? = 0 ? 0 + 1 ? 1 = ? ? ? ?? 1+? ? ? ?? Not allowed 1 = ?(? ?)/??+1

13. Fermi-Dirac distribution for fermions 1 ?FD= ? ? ?? + 1 ? 1 Low ? ?FD= occupancy High ? 0 ? ?

14. Bose-Einstein distribution ? = 0, 1, 2, 3, ?(?) =1 ?? ? ? ? State ?, no particles ?? ? = 1 + ? ? ? ?? + ? 2 ? ? State ?, one particle + ?? ?BE= 0 ? 0 + 1 ? 1 + 2 ? 2 + = ?(? ?)/?? 1 etc 1

15. Compute the average ? Let ? = ? ? ? ? = 1 + ? + ?2+ ?3+ , multiplying ? to ? and subtract, we get ? ?? = 1 or ? = 1 1 ?. ??, then ? = ?=0 ?? ln ? 1 ? ?=0 1 1 ???=? 1 ? ? ?? 1 ? ??= ?? ?=0 ?? ? = ? ? = 1 ?= ? 1= ?? ?

16. Boltzmann distribution 1 ?1? ? ? ? = ?? Average occupation number ? = ?? ? = ? = ??ln?1 ?Boltzmann= ? ? ? ? ?1? ? ??, ? ??

17. Tutorial problems

18. Tutorial problem 6.44