Ordinary Differential Equations at Ishik University
Fundamentals of Ordinary Differential Equations in the context of engineering systems at Ishik University, covering topics such as rates of change, general solutions, and unique solutions with appropriate conditions. Gain insights into incorporating derivatives into mathematical models and understanding the importance of conditions in obtaining unique solutions.
Download Presentation

Please find below an Image/Link to download the presentation.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author.If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.
You are allowed to download the files provided on this website for personal or commercial use, subject to the condition that they are used lawfully. All files are the property of their respective owners.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author.
E N D
Presentation Transcript
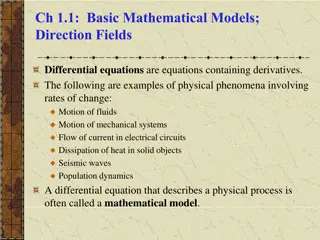
Differential Equations Differential Equations Lecture 2 Ordinary Differential Equations fall semester Instructor: A. S. Brwa / MSc. In Structural Engineering College of Engineering / Ishik University
Ishik University Ordinary Differential Equations Ordinary Differential Equation (ODE): is a differential equation containing one or more functions of one independent variable and its derivativeses 2 Faculty of Engineering Differential Equations Lecture 2 Ordinary Differential Equations
Ishik University Learning Outcomes Understand the use of differential equations in modelling engineering systems Identify the order and type of a differential equation recognize the nature of a general solution Determine the nature of the appropriate additional conditions which will give a unique solution to the equation 3 Faculty of Engineering Differential Equations Lecture 2 Ordinary Differential Equations
Ishik University Ordinary Differential Equations Rate of Change: A rate of change is a rate that describes how one quantity changes in relation to another quantity. If x is the independent variable and y is the dependent variable, then ???? ?? ? ???? =? ???? ?? ? ? ???? ?? ?=?? ?? Rates of change can be positive or negative. This corresponds to an increase or decrease in the y value between the two data points. When a quantity does not change over time, it is called zero rate of change 4 Faculty of Engineering Differential Equations Lecture 2 Ordinary Differential Equations
Ishik University Ordinary Differential Equations Many models of engineering systems involve the rate of change of a quantity. There is thus a need to incorporate derivatives into the mathematical model. These mathematical models are examples of differential equations. 5 Faculty of Engineering Differential Equations Lecture 2 Ordinary Differential Equations
Ishik University Ordinary Differential Equations Accompanying the differential equation will be one or more conditions that let us obtain a unique solution to a particular problem. Often we solve the differential equation first to obtain a general solution; then we apply the conditions to obtain the unique solution. It is important to know which conditions must be specified in order to obtain a unique solution. 6 Faculty of Engineering Differential Equations Lecture 2 Ordinary Differential Equations
Ishik University Case Study Newton s law of cooling as an example When a hot liquid is placed in a cooler environment, experimental observation shows that its temperature decreases to approximately that of its surroundings. A typical graph of the temperature of the liquid plotted against time is shown in Figure 1. 7 Faculty of Engineering Differential Equations Lecture 2 Ordinary Differential Equations
Ishik University 8 Faculty of Engineering Differential Equations Lecture 2 1st Order ODE
Ishik University Case Study After an initially rapid decrease the temperature changes progressively less rapidly and eventually the curve appears to flattenout . Newton s law of cooling states that the rate of cooling of liquid is proportional to the difference between its temperature and the temperature of its environment (the ambient temperature). 9 Faculty of Engineering Differential Equations Lecture 2 Ordinary Differential Equations
Ishik University Case Study To convert this into mathematics, let t be the time elapsed (in seconds, s), y the temperature of the liquid ( ), and ?? the temperature of the liquid at the start (t = 0). The temperature of the surroundings is denoted by?? . Now, we can create a mathematical equation which is equivalent to Newton s law of cooling and state the accompanying condition. 10 Faculty of Engineering Differential Equations Lecture 2 Ordinary Differential Equations
Ishik University Case Study First, find an expression for the rate of cooling, and an expression for the difference between the liquid s temperature and that of the environment: 11 Faculty of Engineering Differential Equations Lecture 2 Ordinary Differential Equations
Ishik University Case Study The rate of cooling is the rate of change of temperature with time: ?? ?? The temperature difference is ? ?? 12 Faculty of Engineering Differential Equations Lecture 2 Ordinary Differential Equations
Ishik University Case Study Now formulate Newton s law of cooling: You should obtain equivalently: d dt = k( s). ?? ?? ? ?? Which is equivalent to ?? ??= ? ? ?? ? is a positive constant of proportion 13 Faculty of Engineering Differential Equations Lecture 2 Ordinary Differential Equations
Ishik University Case Study The negative sign ok ( ?) is present because (? ??) is positive, whereas?? ??must be negative, since ? decreases with time. The units of ? are? 1. The accompanying condition is at t = 0 ? = ?? which simply states the temperature of the liquid when the cooling begins. 14 Faculty of Engineering Differential Equations Lecture 2 Ordinary Differential Equations
Ishik University Case Study In the above Task we call t the independent variable and ? the dependent variable. Since the condition is given at t = 0. so, this is considered as an initial condition. For future reference, the solution of the above differential equation which satisfies the initial condition is. ? = ??+ ?? ??? ?? 15 Faculty of Engineering Differential Equations Lecture 2 Ordinary Differential Equations
Ishik University General Solution of a ODE The general solution of a ODE Consider the equation ? = ??2?where A is an arbitrary constant. If we differentiate it we obtain An arbitrary constant is a constant whose value could be assumed to be anything, it doesn't depend on the other variables in an equation or expression. A constant that's not arbitrary can usually just take one value, such as 1, 2, or 10 ?? ??= 2??2? and so, since ? = ??2?we obtain ?? ??= 2? 16 Faculty of Engineering Differential Equations Lecture 2 Ordinary Differential Equations
Ishik University General Solution of a ODE Thus a differential equation satisfied by y is ?? ??= 2? Note that we have eliminated the arbitrary constant. 17 Faculty of Engineering Differential Equations Lecture 2 Ordinary Differential Equations
Ishik University General Solution of a ODE Now consider the equation ? = ????3? + ????3? Where, A and B are arbitrary constants. Differentiate once , we obtain ?? ??= 3????3? + 3????3? 18 Faculty of Engineering Differential Equations Lecture 2 Ordinary Differential Equations
Ishik University General Solution of a ODE Differentiating a second time gives ?2? ??2= 9????3? 9????3? The right hand side is simply ( 9) times the expression for y. Hence y satisfies the differential equation ?2? ??2= 9? 19 Faculty of Engineering Differential Equations Lecture 2 Ordinary Differential Equations
Ishik University General Solution of a ODE Find a differential equation satisfied by ? = ?cosh2? + ?sinh2? Where, A and B are arbitrary constants. Differentiating once we obtain ?? ??= 2????h2? + 2????h2? 20 Faculty of Engineering Differential Equations Lecture 2 Ordinary Differential Equations
Ishik University General Solution of a ODE Differentiating a second time we obtain ?2? ??2= 4?cosh2? + 4?sinh2? ?2? ??2= 4? 21 Faculty of Engineering Differential Equations Lecture 2 Ordinary Differential Equations
Ishik University General Solution of a ODE We have seen that an expression including one arbitrary constant required one differentiation to obtain a differential equation which eliminated the arbitrary constant. Where two constants were presented, two differentiations were required. 22 Faculty of Engineering Differential Equations Lecture 2 Ordinary Differential Equations
Ishik University General Solution of a ODE Integrate twice the differential equation ?2? ??2=? ?? ?2 2 Where, w and L are constants, to find a general solution for y. where A is an arbitrary constant (of integration) Integrating once: ??2 2 ?3 ?? ??=? + A 2 3 23 Faculty of Engineering Differential Equations Lecture 2 Ordinary Differential Equations
Ishik University General Solution of a ODE Integrating again: ??3 6 ?4 12 ? =? + Ax + B 2 Where, B is second arbitrary constant (of integration) 24 Faculty of Engineering Differential Equations Lecture 2 Ordinary Differential Equations
Ishik University General Solution of a ODE Consider the simple differential equation ?? ??= 2? On integrating, we obtain the general solution ? = ?2+ ? Where, C is an arbitrary constant. As C varies we get different solutions, each of which belongs to the family of solutions 25 Faculty of Engineering Differential Equations Lecture 2 Ordinary Differential Equations
Ishik University General Solution of a ODE Consider the simple differential equation ?? ??= 2? On integrating, we obtain the general solution ? = ?2+ ? Where, C is an arbitrary constant. As C varies we get different solutions, each of which belongs to the family of solutions Figure-2 Shows different values of C 26 Faculty of Engineering Differential Equations Lecture 2 Ordinary Differential Equations
Ishik University Home Work Find differential equations satisfied by 1. ? = ? cos4? + ? sin4? 2. ? = ? sin? + ? sinh? + ? cos? + ? cosh? Solve the differential equation ?2? ??2= 6? 27 Faculty of Engineering Differential Equations Lecture 2 Ordinary Differential Equations