Moment of Inertia Calculations in Engineering Mechanics - Problems and Solutions
The content covers problems related to calculating moments of inertia in engineering mechanics, specifically focusing on triangular areas, shaded areas, and circles. Detailed step-by-step solutions are provided for each problem, including determining moments of inertia about different axes and finding radii of gyration. Diagrams and formulas are utilized to explain the concepts thoroughly.
Download Presentation

Please find below an Image/Link to download the presentation.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author. Download presentation by click this link. If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.
E N D
Presentation Transcript
STATICS (ENGINEERING MECHANICS-I) Area moment of Inertia-Problems October 7, 2024 1
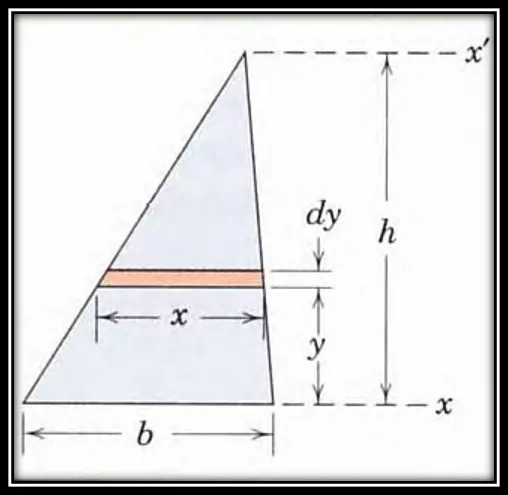
Problem-1 Determine the moments of inertia of the triangular area about: (i) The base, (ii) The parallel axis passing through its centroid, (iii) The parallel axis passing through its vertex, 10/7/2024 2
Solution The i. moment of inertia about the base i.e. - axis : x h h 3 4 1 h y y y 2 = = = = 2 3 bdy I y dA y b bh x 3 4 12 h h 0 0 ii. The moment of inertia x about the centroidal axis I (a distance above h/3 the - axis) : 2 2 1 1 1 h h = = = 3 3 I I A bh bh bh x 3 12 2 3 36 iii . transfer A from the centroiuda axis l to the x'-axis through th vertex e gives : 2 2 2 1 1 2 1 h h = + = + = 3 3 I I A bh bh bh 3 36 2 3 4 10/7/2024 3
Problem-2 For the shaded area and the strip shown in the two figures, calculate the moment of Inertia and radius of gyration about the x-axis (i.e. Ix and rx) y y 3 m 3 m y = x2 / 12 y = x2 / 12 x x 6 m 6 m October 7, 2024 4
Solution y 3 m 3 3 0 0 = = = 1 2 / 6 ( ) 6 ( 2 y 3 dy ) A dA -x dy - y = x2 / 12 3 3 2 / y = = 2 6 2 3 6 m y - 3 2 / x 6 m 0 3 3 = = = 2 2 2 (6 - ) (6 - 12 ) I y dA y x dy y y dy x 0 0 3 3 3 7 2 / y y = = = 2 5/2 4 (6 - 2 3 ) 6 2 3 . 7 714 m I y y dy - x 3 7 2 / 0 0 Radius of gyration about the - axis : x . 7 714 I = = = . 1 339 m r x x 6 A October 7, 2024 5
Solution (Contd.) y 3 m y = x2 / 12 x 6 m Moment of inertia of the strip about its base is, 1 1 dIx= 3 3 ( ) (Fomula : ) dx y bd 3 3 6 6 6 6 7 1 1 1 1 x x dx = = = = = 3 4 7 714 . m I dI y dx x x 3 3 3 3 12 3 12 7 0 0 0 . 7 714 I = = = . 1 339 m r x x 6 A October 7, 2024 6
Problem-3 Calculate the moments of inertia of the area of a circle about a diametral axis and about the polar axis through the center. Specify the radii of gyration. 10/7/2024 7
Solution r 4 r 2 2 = = = 2 ( ) I r dA r r dr 0 0 0 0 z 2 0 Radius of gyration is 4 I r r = = = k z z 2 2 A r 2 = = + symmetry By and we know I I I I I x y z x y 4 4 1 1 r r = + = = = = 2 I I I I I I z x x x x z 2 2 2 4 Radius of gyration is 4 I r r = = = k x x 2 4 2 A r 10/7/2024 8
Moment of Inertia of Composite Areas Important Formulas October 7, 2024 9
Problem-4 Determine the moment of inertia of the shaded area about the x-axis. y 100 mm 20 mm 50 mm 20 mm 170 mm 20 mm x O October 7, 2024 10
Solution y 1 100 mm = = 3 6 4 20 mm 20 240 92 16 . 10 mm I 1 x 3 3 50 mm 2 1 = + = 3 2 6 4 60 20 ( 60 20 ) 230 63 52 . 10 mm I 20 mm 2 x 12 1 1 = + = 3 2 6 4 170 mm 20 70 ( 70 20 ) 205 59 41 . 10 mm I 3 x 12 20 mm = + + = 6 4 215 09 . 10 mm I I I I Ans. x 1 2 3 x x x x O October 7, 2024 11