Linear Modulation Performance in Fading Channels: Overview & Announcements
Explore the latest updates and announcements from Lecture 9, covering topics such as outage probability, average error probability, and linear modulation in fading channels. Stay informed about project proposal deadlines and upcoming midterm details.
Download Presentation

Please find below an Image/Link to download the presentation.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author. If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.
You are allowed to download the files provided on this website for personal or commercial use, subject to the condition that they are used lawfully. All files are the property of their respective owners.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author.
E N D
Presentation Transcript
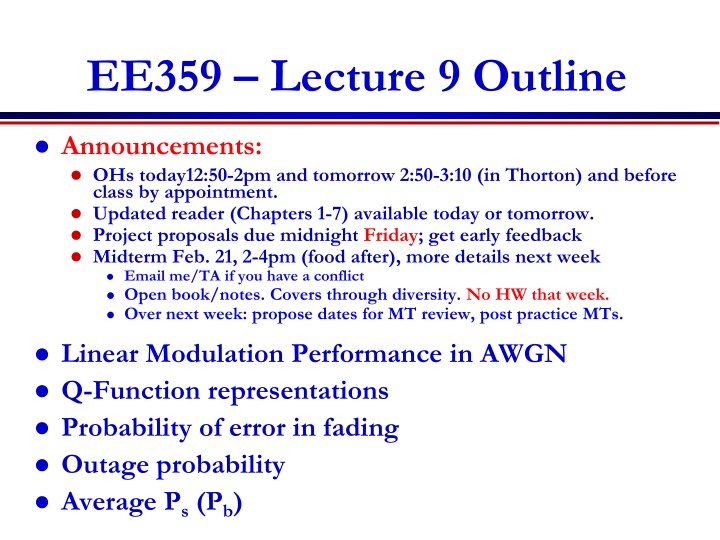
EE359 Lecture 9 Outline Announcements: OHs today12:50-2pm and tomorrow 2:50-3:10 (in Thorton) and before class by appointment. Updated reader (Chapters 1-7) available today or tomorrow. Project proposals due midnight Friday; get early feedback Midterm Feb. 21, 2-4pm (food after), more details next week Email me/TA if you have a conflict Open book/notes. Covers through diversity. No HW that week. Over next week: propose dates for MT review, post practice MTs. Linear Modulation Performance in AWGN Q-Function representations Probability of error in fading Outage probability Average Ps(Pb)
Review of Last Lecture Capacity in Flat-Fading: known at TX/RX Optimal Adaptation: Power: / 0-1/ Rate: Blog2( / 0); Depend on p( ) only through 0 Channel Inversion and Truncated Inversion Received SNR constant; Capacity is Blog2(1+ ) above an outage level associated with truncation Capacity of ISI channels Divide wideband channel into narrowband flat-fading subchannels of bandwidth B approximately equal to Bc Each subchannel has NB fading approx. independent from others Water-filling of power over freq; or time and freq. 1/|H(f)|2 Total Power 1/ 0 P(fi) Bc f 1/|H(fi)|2 fi
Linear Digital Modulation Signal over ith symbol period: 2 cos( ) ( ) ( 1 = t g s t s i + + ) ( ) sin( 2 ) f t s g t f t 0 2 0 c i c Pulse g(t) typically Nyquist, assumed square Signal constellation defined by (si1,si2) pairs M values for (si1,si2) log2 M bits per symbol We focus on MPSK and MQAM MPSK can be differentially encoded For MQAM and MPSK, Ps depends on Minimum distance dmin (depends on s) # of nearest neighbors M Approximate expression: Standard/alternate Q function ( ) P Q s M M s
Linear Modulation in Fading In fading s and therefore Ps random Performance metrics: Outage probability: p(Ps>Ptarget)=p( < target) Average Ps , Ps: = 0 ( ( ) ) P P p d s s Combined outage and average Ps
Outage Probability Ts Outage Ps Ps(target) t or d Probability that Ps is above target Equivalently, probability s below target Used when Tc>>Ts
Average Ps = ( ( ) ) P P p d s s s s s Ts Ps Ps t or d Expected value of random variable Ps Used when Tc~Ts Error probability much higher than in AWGN alone Rarely obtain average error probability in closed form Probability in AWGN is Q-function, double infinite integral
Average Probability of Error (MQAM) Fading severely degrades performance
Alternate Q Function Analysis Traditional Q function representation ) ( ) ( z x p z Q z 1 2 = = x / 2 e dx x ) 1 , 0 ( N , ~ 2 Infinite integrand, argument in integral limits Average Pe entails infinite integral over Q(z) Craig s representation: 1 / 2 2 2 = d z /(sin ) Q z e ( ) 0 Very useful in fading and diversity analysis AWGN formula: Fading formula: 0 sin M s is MGF of fading pdf of s; , depend on modulation* 5 . = M M P M dx s 2 x s *current reader has -.5 =g notation
Main Points ( ) P Q Ps approximation in AWGN: For MPSK, MQAM s M M s In fading Ps is a random variable, characterized by average value, outage, or combined outage/average Fading greatly increases average Ps or required power for a given target Ps with some outage Outage probability based on target SNR in AWGN. Need to combat flat fading or waste lots of power
