Insights into Non-equilibrium Kinetic Theory: Inhomogeneous Systems
Statistical averages in kinetic theory connect distribution functions to macroscopic properties like pressure and particle flux. When systems are inhomogeneous or away from equilibrium, local equilibrium breaks down, leading to slow relaxation processes towards global equilibrium. The evolution of particle distribution functions can be described through the kinetic equation, offering insights into quantities like thermal conductivity and viscosity in non-equilibrium scenarios.
- Kinetic theory
- Inhomogeneous systems
- Non-equilibrium dynamics
- Statistical mechanics
- Particle distribution
Download Presentation

Please find below an Image/Link to download the presentation.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author. Download presentation by click this link. If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.
E N D
Presentation Transcript
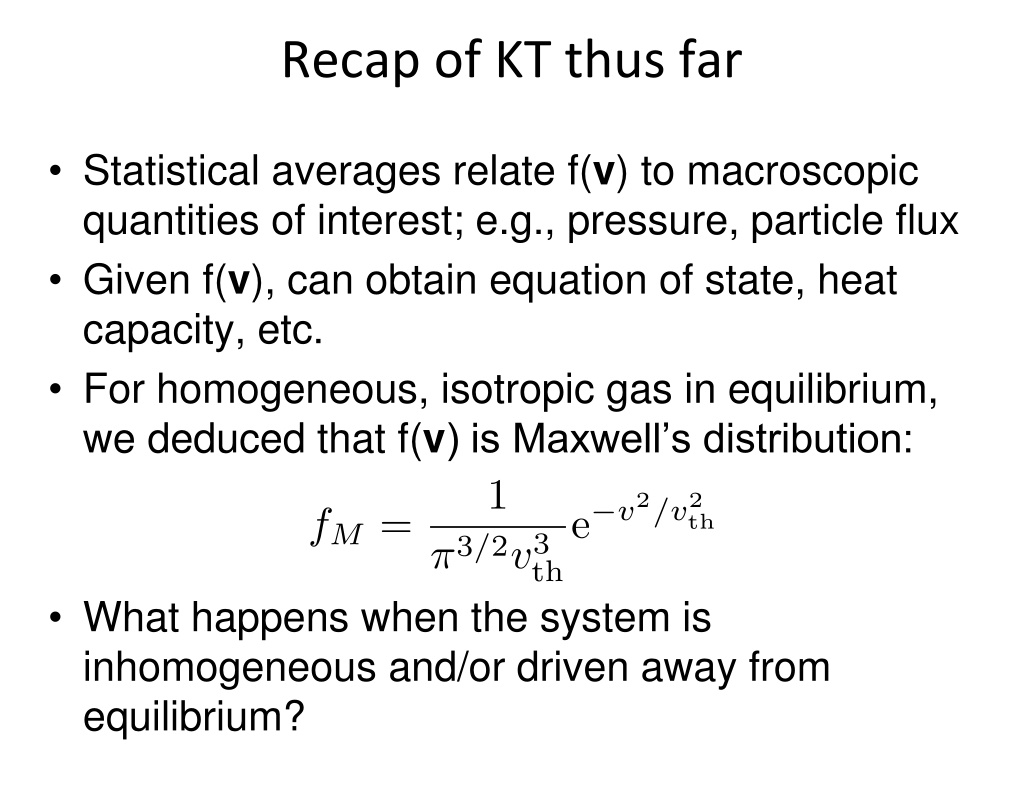
Recap of KT thus far Statistical averages relate f(v) to macroscopic quantities of interest; e.g., pressure, particle flux Given f(v), can obtain equation of state, heat capacity, etc. For homogeneous, isotropic gas in equilibrium, we deduced that f(v) is Maxwell s distribution: What happens when the system is inhomogeneous and/or driven away from equilibrium?
KT4: key ideas Random walk model indicates time scale separation if inhomogeneity length scale >> collisional mean-free-path: Local Maxwellian equilibrium at all space-time points Slow relaxation to global equilibrium via diffusive process Estimates for thermal conductivity and viscosity The kinetic equation describes evolution of the particle distribution function = F(r,v,t) Velocity-space moments of F produce particle, momentum, and energy density, etc.
Initial state T global equilibrium z L ~ (d ln T / dx)-1
T z L ~ (d ln T / dx)-1
KT4: key ideas Random walk model indicates time scale separation if inhomogeneity length scale >> collisional mean-free-path: Local Maxwellian equilibrium at all space-time points Slow relaxation to global equilibrium via diffusive process Estimates for thermal conductivity and viscosity The kinetic equation describes evolution of the particle distribution function = F(r,v,t) Velocity-space moments of F produce particle, momentum, and energy density, etc.
T z L ~ (d ln T / dx)-1
KT4: key ideas Random walk model indicates time scale separation if inhomogeneity length scale >> collisional mean-free-path: Local Maxwellian equilibrium at all space-time points Slow relaxation to global equilibrium via diffusive process Estimates for thermal conductivity and viscosity The kinetic equation describes evolution of the particle distribution function = F(r,v,t) Velocity-space moments of F produce particle, momentum, and energy density, etc.
How might one modify the kinetic equation to account for an external force, e.g., gravity?
KT4: key ideas Random walk model indicates time scale separation if inhomogeneity length scale >> collisional mean-free-path: Local Maxwellian equilibrium at all space-time points Slow relaxation to global equilibrium via diffusive process Estimates for thermal conductivity and viscosity The kinetic equation describes evolution of the particle distribution function = F(r,v,t) Velocity-space moments of F produce particle, momentum, and energy density, etc.
KT4: key ideas Random walk model indicates time scale separation if inhomogeneity length scale >> collisional mean-free-path: Local Maxwellian equilibrium at all space-time points Slow relaxation to global equilibrium via diffusive process Estimates for thermal conductivity and viscosity The kinetic equation describes evolution of the particle distribution function = F(r,v,t) Velocity-space moments of F produce particle, momentum, and energy density, etc.