Injective, Surjective, and Bijective Functions
Learn about the concepts of injective, surjective, and bijective functions through detailed explanations and examples. Explore the relationships between inputs and outputs in functions to understand their distinct characteristics. Gain insights into crucial elements such as domain, codomain, and range to identify different types of functions and their properties.
Download Presentation

Please find below an Image/Link to download the presentation.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author.If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.
You are allowed to download the files provided on this website for personal or commercial use, subject to the condition that they are used lawfully. All files are the property of their respective owners.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author.
E N D
Presentation Transcript
LCHL Strand 5 Functions: Injective, Surjective and Bijective Functions Culan O Meara Ballinrobe Community School
Relations and Functions Relations Injective Bijective Surjective Functions Author: Culan O'Meara
Relations and Functions If we have the set of relations, within that set we have relations that are not functions and ones that are Within the set of functions, we have some that are functions but neither injective, surjective nor bijective We then have functions that are both injective and surjective which are called bijective Author: Culan O'Meara
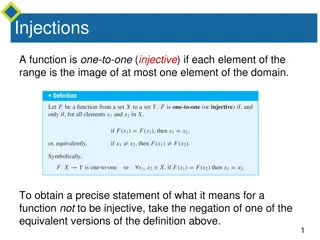
Function f(x)=2x Injective Functions These are functions who have one-to-one relationships between inputs and outputs 1 2 1 4 2 All unique inputs must be mapped to a unique output In the diagram, we have f(x)=2x with Domain {1,2,3,4}, Codomain {1,2,4,6,8,19} and Range {2,4,6,8}. All inputs map onto one and only one output and vice versa 6 3 8 4 For injectivity, It s not relevant that 1 and 19 are unmapped 19 Codomain Range Domain Codomain Author: Culan O'Meara
Function f(x)=2x Surjective Functions 1 2 1 These are functions who have an onto relationship between inputs and outputs All elements of the Codomain must be mapped onto . If Surjective then Codomain = Range In the diagram from last slide, we can see that 1 and 19 are NOT mapped onto so therefore this example is not surjective 4 2 6 3 8 4 19 Domain Codomain Author: Culan O'Meara
Surjective Functions When determining whether a function is surjective or not, it is very important you are aware of what the Domain and Codomain are These are given in the more formal description of the function Basic Function: ? ? = ?? More detailed: ?: ?,?,?,? ?,?,?,?,?,?? :? ?? Function name: ? Domain: 1,2,3,4 Codomain: 1,2,4,6,8,19 Mapping: ? 2? Author: Culan O'Meara
Function f(x)=2x ?: 1,2,3,4 1,2,4,6,8,19 :? 2? Surjective Functions 1 2 1 For our example shown, the function ?: 1,2,3,4 1,2,4,6,8,19 :? 2? is NOT surjective as the Codomain is {1,2,4,6,8,19} whilst the Range is {2,4,6,8} 4 2 6 3 8 4 19 Domain Codomain Author: Culan O'Meara
Function f(x)=2x ?: 1,2,3,4 2,4,6,8 :? 2? Surjective Functions 2 1 For this example, the function ?: 1,2,3,4 2,4,6,8 :? 2?IS surjective as the Codomain = Range = {2,4,6,8} All elements of the Codomain have been mapped onto 4 2 6 3 8 4 Domain Codomain Author: Culan O'Meara
Function f(x)=2x ?: 1,2,3,4 2,4,6,8 :? 2? Bijective Functions 2 1 Bijective functions are those which are BOTH injective AND surjective. The example shown IS bijective Injective? YES Surjective? YES Therefore it must also be bijective 4 2 6 3 8 4 Domain Codomain Author: Culan O'Meara