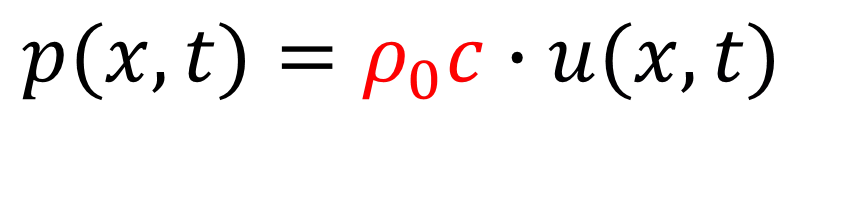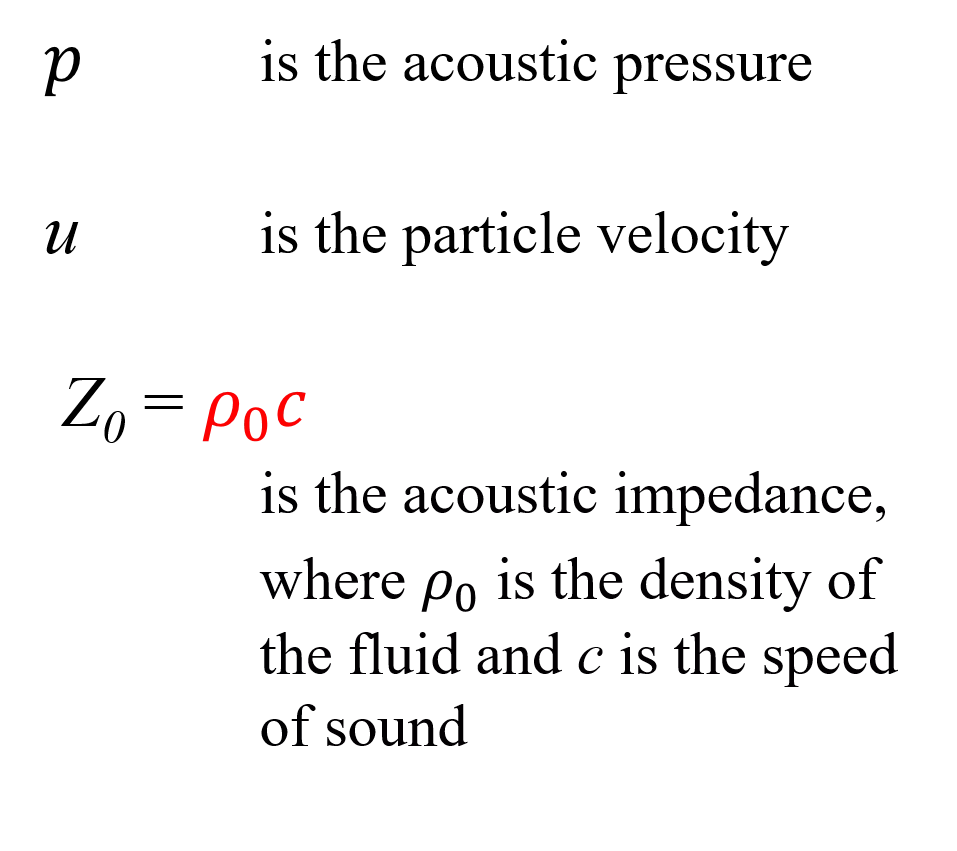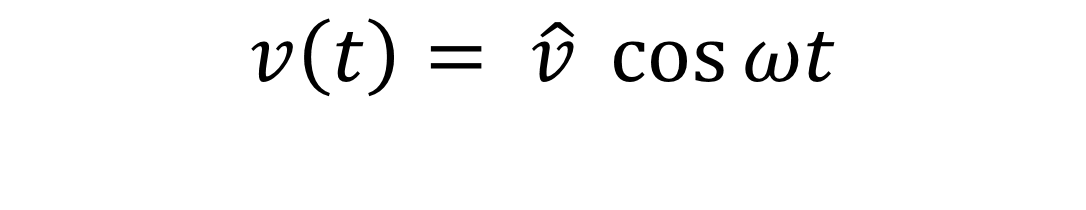Influence of Environmental Factors on Sound Pressure Levels

Slide Note
Embed
Share

In this study, the relationship between sound pressure, particle velocity, and the acoustic impedance is explored in the context of varying humidity, temperature, and barometric pressure. The ideal gas law is used to determine the density of both dry and humid air, considering parameters such as partial pressure of water vapor and relative humidity. The results present the density of humid air under different humidity levels, barometric pressure set at 1013.25 hPa, and temperature values in Celsius. This research provides valuable insights into how environmental conditions impact sound pressure levels.

teigan
teigan Follow

Uploaded on Jul 17, 2024 | 1 Views

Download Presentation

Please find below an Image/Link to download the presentation.

The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author. Download presentation by click this link. If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.

E N D

Presentation Transcript

  1. IWGMU-11-06 Derivation of the Influence of Humidity, Temperature and Barometric pressure on sound pressure level Submitted by Shervin Solhkonan on behalf of the HCV Sub-group IWG MU IWGMU-11-06, 2021-05-25 GRBP IWG MU 1

  2. Relation between sound pressure and particle velocity ? is the acoustic pressure u is the particle velocity ?(?,?) = ?0? ?(?,?) Z0= ?0? is the acoustic impedance, where ?0 is the density of the fluid and c is the speed of sound ? ? = ? cos?? How is the acoustic impedance Z dependent on temperaure, barometic pressure and humidity? IWGMU-11-06, 2021-05-25 GRBP IWG MU 2

  3. Ideal gas law density of air The Ideal gas law is described as ? ? = ?? ? where is the densitiy [kg/m3] P is the barometric pressure, [Pa] Rdis the specific gas constant for dry air, with value 287,058 J/(kg K) T is the temperature in Kelvin IWGMU-11-06, 2021-05-25 GRBP IWG MU 3

  4. Ideal gas law density of humid air For humid air, the density of air can be described as (1) where pd is the partial pressure of dry air Rdis the specific gas constant for dry air, 287,058 J/(kgK) pvis the pressure of water vapor (Pa) Md = Molar mass of dry air, 0,0289654 kg/mol Mv = Molar mass of water vapor, 0,018016 kg/mol R = Universal gas constant 8,314 J/(K mol) IWGMU-11-06, 2021-05-25 GRBP IWG MU 4

  5. Density of humid air The pressure of water vapor pvcan be calculated from the saturation vapor pressure and the relative humidity pv = ? psat where ?is the relative humidity psatis the saturation vapor pressure the saturation vapor pressure is given by Tetens equation [2] 7,5? ?+237,3 ????= 610,78 10 The partial pressure of dry air pd is simply described as pd = p - pv where p is the absolut pressure IWGMU-11-06, 2021-05-25 GRBP IWG MU 5

  6. Result - Density of humid air Barometric pressure set to 1013,25 hPa Density of humid air kg/m^3 Temperature C Humidity % 5 10 15 20 25 30 35 40 100 90 80 70 60 50 1,265 1,265 1,266 1,266 1,267 1,267 1,241 1,242 1,242 1,243 1,243 1,244 1,217 1,218 1,219 1,220 1,220 1,221 1,194 1,195 1,196 1,197 1,198 1,199 1,170 1,171 1,173 1,174 1,176 1,177 1,146 1,148 1,150 1,152 1,153 1,155 1,122 1,124 1,126 1,129 1,131 1,134 1,096 1,099 1,102 1,106 1,109 1,112 40 1,267 1,244 1,222 1,200 1,178 1,157 1,136 1,115 30 1,268 1,245 1,223 1,201 1,180 1,159 1,138 1,118 20 1,268 1,246 1,224 1,202 1,181 1,161 1,141 1,121 ? = ?0? 10 1,269 1,246 1,224 1,203 1,183 1,163 1,143 1,124 0 1,269 1,247 1,225 1,204 1,184 1,164 1,146 1,127 IWGMU-11-06, 2021-05-25 GRBP IWG MU 6

  7. Result - Density of humid air - normalized Barometric pressure set to 1013,25 hPa Density of humid air, normalized to 5 C and 0% humidity Temperature C 5 0,997 0,978 0,997 0,979 0,998 0,979 0,998 0,979 0,998 0,980 0,998 0,980 0,999 0,981 0,999 0,981 0,999 0,982 1,000 0,982 1,000 0,982 Humidity % 10 15 20 25 30 35 40 100 90 80 70 60 50 40 30 20 10 0,960 0,960 0,961 0,962 0,962 0,963 0,963 0,964 0,964 0,965 0,965 0,942 0,943 0,943 0,944 0,945 0,945 0,946 0,947 0,947 0,948 0,949 0,924 0,925 0,926 0,927 0,927 0,928 0,929 0,930 0,931 0,932 0,933 0,906 0,907 0,908 0,909 0,911 0,912 0,913 0,914 0,915 0,916 0,918 0,888 0,889 0,891 0,892 0,894 0,895 0,897 0,898 0,900 0,901 0,903 0,870 0,872 0,874 0,875 0,877 0,879 0,881 0,883 0,885 0,886 0,888 0 ? = ?0? Density of humid air, normalized to discrete temperatures and 0 % humidity Temperature C 5 10 0,997 0,996 0,997 0,996 0,998 0,997 0,998 0,997 0,998 0,997 0,998 0,998 0,999 0,998 0,999 0,999 0,999 0,999 1,000 1,000 1,000 1,000 IWGMU-11-06, 2021-05-25 Humidity % 15 20 25 30 35 40 100 90 80 70 60 50 40 30 20 10 0,994 0,995 0,996 0,996 0,997 0,997 0,998 0,998 0,999 0,999 1,000 0,993 0,993 0,994 0,995 0,996 0,996 0,997 0,998 0,999 0,999 1,000 0,990 0,991 0,992 0,993 0,994 0,995 0,996 0,997 0,998 0,999 1,000 GRBP IWG MU 0,987 0,989 0,990 0,991 0,992 0,994 0,995 0,996 0,997 0,999 1,000 0,984 0,985 0,987 0,989 0,990 0,992 0,994 0,995 0,997 0,998 1,000 0,979 0,981 0,983 0,986 0,988 0,990 0,992 0,994 0,996 0,998 1,000 0 7

  8. Results speed of sound ? = ? ?0/?0 ? = 1,40 (??? ???) Speed of sound Temperature C Humidity % 5 10 15 20 25 30 35 40 100 90 80 70 60 50 40 30 20 10 334,9 334,8 334,8 334,7 334,7 334,6 334,5 334,5 334,4 334,4 334,3 338,1 338,0 337,9 337,9 337,8 337,7 337,6 337,5 337,5 337,4 337,3 341,4 341,3 341,2 341,0 340,9 340,8 340,7 340,6 340,5 340,4 340,3 344,7 344,6 344,4 344,3 344,1 344,0 343,8 343,7 343,5 343,4 343,2 348,2 348,0 347,8 347,6 347,4 347,2 347,0 346,8 346,5 346,3 346,1 351,8 351,5 351,3 351,0 350,7 350,4 350,1 349,9 349,6 349,3 349,0 355,6 355,3 354,9 354,5 354,1 353,8 353,4 353,0 352,6 352,3 351,9 359,7 359,2 358,7 358,2 357,7 357,2 356,7 356,2 355,7 355,2 354,7 ? = ?0? 0 The adiabatic inde? ? is 1,398 for humid air. The difference to ? for dry air is so small that 1,4 can be used in our calculations. IWGMU-11-06, 2021-05-25 GRBP IWG MU 8

  9. Results Acoustic Impedance Acoustic impedance * c Temperature C Humidity % 5 10 15 20 25 30 35 40 100 90 80 70 60 50 40 30 20 10 423,6 423,7 423,8 423,8 423,9 424,0 424,0 424,1 424,2 424,2 424,3 419,6 419,7 419,8 419,9 420,0 420,1 420,2 420,3 420,3 420,4 420,5 415,5 415,7 415,8 415,9 416,1 416,2 416,3 416,5 416,6 416,7 416,9 411,5 411,7 411,9 412,0 412,2 412,4 412,6 412,8 412,9 413,1 413,3 407,4 407,6 407,9 408,1 408,4 408,6 408,9 409,1 409,3 409,6 409,8 403,2 403,5 403,8 404,2 404,5 404,8 405,1 405,5 405,8 406,1 406,4 398,9 399,3 399,7 400,1 400,6 401,0 401,4 401,8 402,3 402,7 403,1 394,3 394,9 395,5 396,0 396,6 397,1 397,7 398,2 398,8 399,3 399,9 ? = ?0? 0 Acoustic impedance * c, normalized to 5 C and 0 % humidity Temperature C 5 10 0,998 0,989 0,999 0,989 0,999 0,989 0,999 0,990 0,999 0,990 0,999 0,990 0,999 0,990 1,000 0,990 1,000 0,991 1,000 0,991 1,000 0,991 IWGMU-11-06, 2021-05-25 Humidity % 15 20 25 30 35 40 100 90 80 70 60 50 40 30 20 10 0,979 0,980 0,980 0,980 0,981 0,981 0,981 0,982 0,982 0,982 0,982 0,970 0,970 0,971 0,971 0,972 0,972 0,972 0,973 0,973 0,974 0,974 0,960 0,961 0,961 0,962 0,962 0,963 0,964 0,964 0,965 0,965 0,966 GRBP IWG MU 0,950 0,951 0,952 0,953 0,953 0,954 0,955 0,956 0,956 0,957 0,958 0,940 0,941 0,942 0,943 0,944 0,945 0,946 0,947 0,948 0,949 0,950 0,929 0,931 0,932 0,933 0,935 0,936 0,937 0,939 0,940 0,941 0,942 9 0

  10. Result difference in sound pressure level Influence of humidity and temperature on sound pressure level, normalized to 5C and 0% relative humidity Temperature C 5 C 10 C 15 C 20 C 25 C -0,01 -0,10 -0,18 -0,27 -0,35 -0,01 -0,10 -0,18 -0,26 -0,35 -0,01 -0,09 -0,18 -0,26 -0,34 -0,01 -0,09 -0,17 -0,25 -0,34 -0,01 -0,09 -0,17 -0,25 -0,33 -0,01 -0,09 -0,17 -0,25 -0,33 -0,01 -0,09 -0,16 -0,24 -0,32 0,00 -0,08 -0,16 -0,24 -0,32 0,00 -0,08 -0,16 -0,24 -0,31 0,00 -0,08 -0,16 -0,23 -0,31 0,00 -0,08 -0,15 -0,23 -0,30 Humidity % 30 C -0,44 -0,44 -0,43 -0,42 -0,42 -0,41 -0,40 -0,39 -0,39 -0,38 -0,37 35 C -0,54 -0,53 -0,52 -0,51 -0,50 -0,49 -0,48 -0,47 -0,46 -0,45 -0,44 40 C -0,64 -0,62 -0,61 -0,60 -0,59 -0,57 -0,56 -0,55 -0,54 -0,53 -0,51 100 90 80 70 60 50 40 30 20 10 ? ??= 20log ???? 0 Peak-to-peak, SPL due to temperatur: 0,63 dB Peak-to-peak, SPL due to humidity: 0,13 dB IWGMU-11-06, 2021-05-25 GRBP IWG MU 10

  11. Relation between barometic pressure and density Again, the ideal gas law to calculate the air density P = R T Let the temperature T be constant (here put to 5 C) Sound pressure difference ? = ?0? Barometic pressure (hPa) Air density (kg/m3) Normalized to 1013,25 hPa Acoustic imedance 950 960 970 980 990 1000 1010 1020 1030 1040 1050 1,19 1,20 1,22 1,23 1,24 1,25 1,27 1,28 1,29 1,30 1,32 398,0 402,2 406,4 410,6 414,8 418,9 423,1 427,3 431,5 435,7 439,9 0,94 0,95 0,96 0,97 0,98 0,99 1,00 1,01 1,02 1,03 1,04 -0,5 -0,4 -0,3 -0,2 -0,1 -0,1 0,0 0,1 0,2 0,3 0,4 Peak-to-peak, SPL difference due to atmospheric pressure: 0,9 dB IWGMU-11-06, 2021-05-25 GRBP IWG MU 11

  12. Inserted in the HVC Excelsheet Type B or/and A: Deviations of the meas. result (peak- peak) Type B Conbine d standard uncertai nty Type B: Type B & A Standard uncertain ty Type A Standard uncertain ty theoretic al derivatio ns Deviations of the meas. result (peak-peak) standard deviatio n contribu tion [%] Type B & A variance Type A Variance statistica l methods Input Quantity probability distribution Same as M1,N1 situation variance Status Test {Uncertainty of vehicle sound emission} Lwot Lwot Day to day Acoustic effects of sound transmission in air related to ambient temperature Acoustic effects of sound transmission in air related to barometric pressure Acoustic effects of sound transmission in air related to humidity Ambient air temperature effect on vehicle sound due to air density influence on engine power Ambient air temperature effect on ICE vehicles due to tyre noise between 5-40 C; Barometric pressure air density effect on engine power (powertrain behavior based on R85) 0,6 rectangular 0,030 0,173 5,6% 0,30 X 0,9 rectangular 0,068 0,260 12,5% 0,00 X 1,9 0,1 rectangular 0,001 0,029 0,2% 0,00 0,46 0,56 0,00 1,0 rectangular 0,083 0,289 15,5% 0,00 x 0,4 rectangular 0,013 0,115 2,5% 0,00 X 0,4 0,4 rectangular 0,013 0,115 2,5% 0,01 x IWGMU-11-06, 2021-05-25 GRBP IWG MU 12

  13. Assumptions in our calculations ? = ? ? Real situation different temp and impedance Situation in calculations same temp and impedance What are the implications of our simplifications? Will the engine compartment be the same regardless of ambient temperature? ?1 ?1 ?1 ?1 ?2 ?1 ?1 ?2 ?1= ambient temperature ?2=Engine compartment temperature IWGMU-11-06, 2021-05-25 GRBP IWG MU 13

  14. References [1] Shelquist, R (2009) Equations - Air Density and Density Altitude [2] Shelquist, R (2009) Algorithms - Schlatter and Baker IWGMU-11-06, 2021-05-25 GRBP IWG MU 14

Related


Understanding Fluid Pressure in Oil Field Hydraulics

Understanding Fluid Pressure in Oil Field Hydraulics

christabel
Understanding Sound Waves and How They Travel

Understanding Sound Waves and How They Travel

delyth
Measurement Methods for Pressure Evaluation

Measurement Methods for Pressure Evaluation

merrick
Understanding Horizontal Distribution of Air Pressure and Pressure Belts on the Globe

Understanding Horizontal Distribution of Air Pressure and Pressure Belts on the Globe

effie
Understanding Pressure Measurement and Hydrostatic Forces on Surfaces

Understanding Pressure Measurement and Hydrostatic Forces on Surfaces

lucan
Understanding Audio Basics in Television Operations

Understanding Audio Basics in Television Operations

erasmus
Understanding Sound Waves and Vibration in Music

Understanding Sound Waves and Vibration in Music

machabee
Understanding Pressure in Physics and Daily Life

Understanding Pressure in Physics and Daily Life

deveraux
Colligative Properties in Solutions: Vapor Pressure, Freezing Point Depression, and Osmotic Pressure

Colligative Properties in Solutions: Vapor Pressure, Freezing Point Depression, and Osmotic Pressure

yisroel
Understanding Pressure and Designing Objects to Alter It

Understanding Pressure and Designing Objects to Alter It

kinley
Understanding Sound Waves and Their Effects

Understanding Sound Waves and Their Effects

deen
Sound Energy by Ethan, Mrs. Mann's Class

Sound Energy by Ethan, Mrs. Mann's Class

grab_el
Understanding Hydrostatic Pressure in Fluid Statics

Understanding Hydrostatic Pressure in Fluid Statics

lwien
Understanding Sound: Energy, Pitch, Transmission, and Speed

Understanding Sound: Energy, Pitch, Transmission, and Speed

zune923
Understanding Different Types of Sound in Media Production

Understanding Different Types of Sound in Media Production

brinnle
Exploring Essential Questions About Sound Waves

Exploring Essential Questions About Sound Waves

gramajo_b
Evolution of Sound Technology in Cinema: A Historical Perspective

Evolution of Sound Technology in Cinema: A Historical Perspective

dem_roz
Understanding the Science of Sound and Its Effects

Understanding the Science of Sound and Its Effects

pau_bas
Understanding Digital Audio and Sound Characteristics in Multimedia

Understanding Digital Audio and Sound Characteristics in Multimedia

kaed_7
Optimal Blood Pressure Levels to Prevent Cognitive Decline in Older Adults

Optimal Blood Pressure Levels to Prevent Cognitive Decline in Older Adults

brily
Understanding the Influence of Words on Sound Recognition in Interactive and Non-interactive Models

Understanding the Influence of Words on Sound Recognition in Interactive and Non-interactive Models

mohl_abe
Understanding Pressure Sores and Preventive Interventions for Bedridden Patients

Understanding Pressure Sores and Preventive Interventions for Bedridden Patients

vlytt

More Related Content