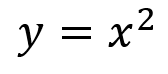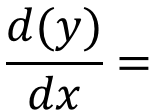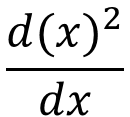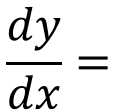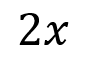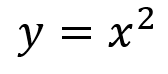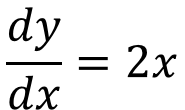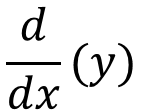Implicit Differentiation Explained: Techniques and Examples
Learn about implicit differentiation, a technique used to differentiate functions without rearranging them. This process involves differentiating both sides of an equation with respect to a variable, allowing you to find derivatives of functions where equations are not explicitly expressed in terms of a single variable. The explanation covers the notation, steps, and methods involved in implicit differentiation, emphasizing the importance of understanding the process clearly. Examples and visual aids illustrate how to apply implicit differentiation effectively.
Download Presentation

Please find below an Image/Link to download the presentation.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author. Download presentation by click this link. If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.
E N D
Presentation Transcript
Teachings for Exercise 9H Teachings for Exercise 9H
Differentiation ? = ?2 You need to be able to differentiate functions implicitly, without needing to rearrange them Follow the usual pattern for differentiation ?? ??= 2? In reality, there is an extra step in this process We are going to start by revisiting and clarifying some of the notation that is used for differentiation, as well as seeing the way things might be written in this section It is important that you understand this step as it will help you understand the process of implicit differentiation more clearly ? = ?2 A small change in ? divided by a small change in ?, will be the same as a small change in ?2 divided by a small change in ? =?(?)2 ?? ?(?) ?? ? ??(?) We can work out the right side (essentially, we can only follow the pattern if the term we differentiate is the same as the term we are differentiating with respect to ?? ??= 2? Note that the left side can also be written as The left side we leave as it is So really, if we are differentiating with respect to ?, we can only work out the ?-terms 9H
Differentiation You need to be able to differentiate functions implicitly, without needing to rearrange them ?3= 3?2 Differentiate both sides with respect to ? ? ??(?3) = ? ??(3?2) The right side we can work out since the terms are in ?, and we differentiated with respect to ? Differentiate the following equation implicitly: ? ??(?3) = 6? On the left side, we can rewrite (effectively using the chain rule) ? ??(?3) ?? ?3= 3?2 ??= 6? Now since the differential is with respect to ?, we can follow the standard pattern 3?2?? Using implicit differentiation, you do not need to rearrange anything first ??= 6? Divide both sides by 3?2 ?? ??= 6? 3?2 So we have a formula for the gradient of the curve, but in this case it is in terms of both ? and ? Sometimes a single coordinate is not enough to determine the gradient 9H
Differentiation You need to be able to differentiate functions implicitly, without needing to rearrange them ? ???(?) Differentiate the ? term Multiply by ?? = ? (?)?? ?? As a general rule, to differentiate a ? term with respect to ?, you should differentiate ? as normal, and multiply it by ?? ?? ?? 9H
? ???(?) = ? (?)?? Differentiation ?? You need to be able to differentiate functions implicitly, without needing to rearrange them Below is a sketch of the circle with equation ?2+ ?2= 25, 5 ? 5, 5 ? 5. Find the gradient of the curve where ? = 4 ? 5 Notice that when ? = 4, there are two possible gradients 3 These points have different ?- coordinates, so that means that the gradient function must also contain a term in ? ? -5 4 5 -3 -5 9H
? ???(?) = ? (?)?? Differentiation ?? ?2+ ?2= 25 You need to be able to differentiate functions implicitly, without needing to rearrange them Differentiate each term with respect to ? 2? + 2??? ??= 0 Subtract 2? 2??? Below is a sketch of the circle with equation ?2+ ?2= 25, 5 ? 5, 5 ? 5. Find the gradient of the curve where ? = 4 ? ??= 2? Divide by 2? ?? ??= 2? 2? Simplify ?? ??= ? 5 ? 3 ? -5 4 5 -3 -5 9H
? ???(?) = ? (?)?? Differentiation ?? ?? ??= ? You need to be able to differentiate functions implicitly, without needing to rearrange them ? The higher coordinate is (4,3) The lower coordinate is (4,-3) ?? ??= ?? ??=4 4 ?? ??= 4 Below is a sketch of the circle with equation ?2+ ?2= 25, 5 ? 5, 5 ? 5. Find the gradient of the curve where ? = 4 ? 3 3 3 5 ???????? = 4 3 3 ? -5 4 5 ???????? =4 -3 3 -5 9H
? ???(?) = ? (?)?? Differentiation ?? ?3+ ? + ?3+ 3? = 6 You need to be able to differentiate functions implicitly, without needing to rearrange them Differentiate each term with respect to ? 3?2+ 1 + 3?2?? ??+ 3?? ??= 0 Subtract 1 and subtract 3?2 3?2?? ??+ 3?? ??= 1 3?2 Find ?? ?? in terms of ? and ? when: Factorise left side ?? ?? 3?2+ 3 = 1 3?2 ?3+ ? + ?3+ 3? = 6 Divide by 3?2+ 3 ?? ??= 1 3?2 3?2+ 3 9H
? ???(?) = ? (?)?? If:? = ?? ?? ??= ??? ??+ ??? ?? Differentiation If: ? =? ??? ?? ??? ?2 ?? ??= ? ?? ?? 4??2+6?2 You need to be able to differentiate functions implicitly, without needing to rearrange them = 10 ? Use the product rule for this term ? = ?2 Given that 4??2+6?2 value of ?? Differentiate (with respect to ?) Differentiate (with respect to ?) ? = 4? ?= 10, find the ?? at the point (1,1) ?? ??= 2??? ?? ??= 4 ?? Substitute these expressions into the product rule In this question you have two functions multiplied in the first term, and a division of functions in the second term ?? ??= ??? ??+ ??? Sub in expressions ?? This means that you will need to apply your knowledge of the product and quotient rules as well! ?? ??= (4?) 2??? + (?2)(4) ?? Simplify ?? ??= 8???? ??+ 4?2 8???? ??+ 4?2 9H
? ???(?) = ? (?)?? If:? = ?? ?? ??= ??? ??+ ??? ?? Differentiation If: ? =? ??? ?? ??? ?2 ?? ??= ? ?? ?? 4??2+6?2 You need to be able to differentiate functions implicitly, without needing to rearrange them = 10 ? Use the quotient rule for this term ? = ? ? = 6?2 Differentiate (with respect to ?) Differentiate (with respect to ?) Given that 4??2+6?2 value of ?? ?= 10, find the ?? at the point (1,1) ?? ??= 1?? ?? ??= 12? ?? Substitute these expressions into the quotient rule In this question you have two functions multiplied in the first term, and a division of functions in the second term ??? ?? ??? ?2 ?? ??= ?? Sub in expressions (?)(12?) (6?2) 1?? This means that you will need to apply your knowledge of the product and quotient rules as well! ?? ??= ?? ?2 Simplify terms 12?? 6?2?? 12?? 6?2?? ?? ??= ?? 8???? ?? ??+ 4?2 ?2 ?2 9H
? ???(?) = ? (?)?? If:? = ?? ?? ??= ??? ??+ ??? ?? Differentiation If: ? =? ??? ?? ??? ?2 ?? ??= ? ?? ?? 4??2+6?2 You need to be able to differentiate functions implicitly, without needing to rearrange them = 10 ? Differentiate with respect to ?, using the results we just obtained 12?? 6?2?? 8???? ?? ??+ 4?2+ = 0 Given that 4??2+6?2 value of ?? ?2 ?= 10, find the ?? at the point (1,1) Rather than rearranging, you can actually sub in the ? and ? values now! 12(1)(1) 6(1)2?? 8(1)(1)?? ?? ??+ 4(1)2+ = 0 (1)2 Simplify 8?? ??+ 4 + 12 6?? ??= 0 Collect like terms 2?? ??+ 16 = 0 Subtract 16, divide by 2 ?? ??= 8 9H
? ???(?) = ? (?)?? If:? = ?? ?? ??= ??? ??+ ??? ?? Differentiation If: ? =? ??? ?? ??? ?2 ?? ??= ? ?? ?? ? = ?2? Differentiate (with respect to ?) ? = ??? Differentiate (with respect to ?) You need to be able to differentiate functions implicitly, without needing to rearrange them ?? ??= 2?2? ?? ??=1 ?? ?? ? Substitute these expressions into the product rule ?? ??= ??? ??+ ??? Find the value of ?? ?? at the point (1,1), when: Sub in expressions ?? ?? ??= (?2?) 1 ? ?? ?? + (???)(2?2?) ?2???? = ? + ? + 2 Simplify ?? ??=?2? ?? ??+ 2?2???? Use the product rule to differentiate the left side ? ?2? ? ?? ??+ 2?2???? 9H
? ???(?) = ? (?)?? If:? = ?? ?? ??= ??? ??+ ??? ?? Differentiation If: ? =? ??? ?? ??? ?2 ?? ??= ? ?? ?? You need to be able to differentiate functions implicitly, without needing to rearrange them ?2???? = ? + ? + 2 Differentiate each term with respect to ?, using the expression we found ?2? ? ?? ??+ 2?2???? = 1 +?? ?? Sub in values now, rather than rearranging first ?2(1) (1) ?? ??+ 2?2(1)??(1) = 1 +?? Find the value of ?? ?? at the point (1,1), when: ?? Calculate/simplify terms (??1 = 0) ?2?? ??= 1 +?? ?? ?2???? = ? + ? + 2 Subtract ?? ?? ?2?? ?? ?? ??= 1 Use the product rule to differentiate the left side Factorise left side ?? ?? ?2 1 = 1 Divide by the bracket ?2? ? ?? ??+ 2?2???? ?? ??= 1 ?2 1 9H