Game Theory and Oligopoly Interdependence

Game theory explores strategic behavior in oligopolistic markets, highlighting interdependence among firms. Topics include prisoners dilemma, advantages of first mover, and topical applications like tacit collusion. Types of games such as simultaneous and sequential are analyzed, showcasing real-world scenarios like patent races and market entry decisions.

• Game Theory
• Oligopoly
• Interdependence
• First Mover
• Strategic Behavior

shael Follow

Uploaded on Mar 01, 2025 | 0 Views

Download Presentation

Please find below an Image/Link to download the presentation.

The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author.If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.

You are allowed to download the files provided on this website for personal or commercial use, subject to the condition that they are used lawfully. All files are the property of their respective owners.

The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author.

Presentation Transcript

1. Oligopoly and Game Theory Oligopoly and Game Theory Topic 3.3.9 Students should be able to: Use simple game theory to illustrate the interdependence that exists in oligopolistic markets Understanding the prisoners dilemma and a simple two firm/two outcome model. Students should analyse the advantages/disadvantages of being a first mover Students will not be expected to have an understanding of the Nash Equilibrium

2. Oligopoly and Game Theory Oligopoly and Game Theory Topic 3.3.9

3. Game Theory and Oligopoly Game theory is the study of how people and businesses behave in strategic situations (i.e. when they must consider the effect of other people s responses to their own actions). A game consists of: 1. Players 2. Strategies 3. Payoffs 4. Might also involve some form of pre-commitment Oligopoly theory often makes heavy use of game theory to model the actual behaviour of businesses in concentrated markets

4. Topical Applications of Game Theory Tacit Collusion between Firms in an Oligopoly Patent Races / Innovation Effort / Product Testing Central Bank Currency Policy - Intervention Business reactions to the new UK Living Wage Market Entry / Exit Decisions Auction Tactics e.g. bidding for a franchise

5. Different Types of Games Simultaneous Games When players effectively make their decisions at the same time They don t know the choices of other players when making their decisions Examples: The Prisoners Dilemma Rock, Paper, Scissors Split or Steal in Golden Balls Individual plays in a game of American Football Penalty shoot-outs in cup matches Serve and receive in Tennis Speed dating? Closed bid auctions Athletes choosing to dope or not to dope

6. Different Types of Games Sequential games A sequential game is one in which the players take alternate turns to make their choices. Classic examples: A game of chess Open-outcry auctions with sequential bidding Salary negotiations Haggling to buy a second hand car Making offers on a property It is important to know who is going to move first in a sequential game as their may be a first mover advantage, or even a first mover disadvantage

7. Repeated versus One-Shot Games One-Shot Games Game played only once Pay-off may be such that a game might be impossible to play twice E.g. mutually assured nuclear destruction Slightly different with tactical / conventional warfare In one-shot interaction, individual's often have an incentive to behave opportunistically / selfishly Consider a one shot Prisoners Dilemma game where an individual wants to minimise their own sentence

8. Repeated versus One-Shot Games Repeated games the game played more than once A feature of applied business e.g. decisions on: Pricing (Established supermarkets versus the Deep Discounters) Marketing budgets (Coca Cola v Pepsi) Research and development spend (Samsung v Apple v Huawei) Capital investment and supply capacity forecasts (Cruise ships) More scope for co-operative strategies to emerge Credible threat power history of past behaviours Agents learn by doing if someone continually serves to your backhand in tennis, your backhand improves Repeated games crucial point is the reaction to a defective strategy by another player Tit for Tat Strategy if you defect, I defect in the next round Grim Strategy if you defect, I will defect in all future rounds

9. Valuing the future in repeated games If you get punished tomorrow for bad behaviour today and you value the future sufficiently highly, it is probably in your own self-interest to behave well today! Application: Fines applied to price fixing and other anti-competitive cartels After a cartel fine how likely is the cartel to reform? Are students who have taken an Economics degree less likely to behave cooperatively in their adult lives?

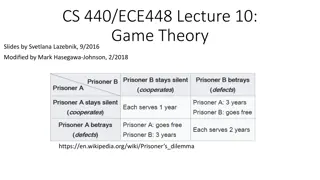
10. Game Theory Prisoners Dilemma Prisoner B Silent Betray Silent (6M,6M) (10Y,0) Prisoner A Betray (0,10Y) (5Y,5Y) Comment on the best strategies for each player and the likely outcome in this game

11. Game Theory Prisoners Dilemma The prisoners' dilemma is a particular game that illustrated why it is difficult to cooperate, even when it is in the best interest of both parties. Both players are assumed to select their own dominant strategies for short-sighted personal gain / self-interest. Eventually, they reach an equilibrium in which they are both worse off than they would have been, if they could both agree to select an alternative (non- dominant) strategy. Prisoner B Silent Betray Silent (6M,6M) (10Y,0) Prisoner A Betray (0,10Y) (5Y,5Y) Comment on the best strategies for each player and the likely outcome in this game

12. Prisoners Dilemma Decision Trees Prisoner B Silent Betray Silent (6M,6M) (10Y,0) Prisoner A Betray (0,10Y) (5Y,5Y)

13. Nash Equilibrium Nash Equilibrium is an important idea in game theory it describes any situation where all of the participants in a game are pursuing their best possible strategy given the strategies of all of the other participants. In a Nash Equilibrium, the outcome of a game that occurs is when player A takes the best possible action given the action of player B, and player B takes the best possible action given the action of player A

14. Game Theory: A Simple Pricing Game In this two firm game, they have to decide whether to set high or low prices The table shows the profits (pay-offs) that results from each set of choices Firm B (right hand figures below) Expected Profit ($bn) High Prices Low Prices High Prices $3bn; $3bn $0bn, $5bn Firm A Low Prices $5bn; $0bn $1bn, $1bn The grid above shows a pay-off matrix it shows a simple pricing game between firm A and firm B. They are assumed to choose their prices at the same time

15. A Simple Pricing Game To understand the game we isolate one firm and assume that Firm B makes the first decision. Assume that each firm is a profit maximiser. Firm B (right hand figures below) Expected Profit ($bn) High Prices Low Prices High Prices $3bn; $3bn $0bn, $5bn Firm A Low Prices $5bn; $0bn $1bn, $1bn The grid above shows a pay-off matrix it shows a simple pricing game between firm A and firm B. They are assumed to choose their prices at the same time

16. A Simple Pricing Game In this game, regardless of what the other firm decides to do, the best response of the other firm is to charge a lower price they may settle at this low price B Profit $bn High Prices Low Prices High Prices $3bn; $3bn $0 bn, $5bn A Low Prices $5bn; $0bn $1bn, $1bn The grid above shows a pay-off matrix it shows a simple pricing game between firm A and firm B. They are assumed to choose their prices at the same time

17. Pricing Game Incentives to Collude If these firms got together and decided to collude by both setting a high price, then both of them would earn higher total profits this would be pareto optimal B Profit $bn High Prices Low Prices High Prices $3bn; $3bn $0 bn, $5bn A Low Prices $5bn; $0bn $1bn, $1bn The grid above shows a pay-off matrix it shows a simple pricing game between firm A and firm B. They are assumed to choose their prices at the same time

18. Non-Price Competition Marketing Spend In this case, the dominant strategy is to advertise, and this would be a Nash equilibrium. Firm B Advertise Don t Advertise Firm A Advertise ( 6m, 6m) ( 8m, 3m) Don t Advertise ( 3m, 8m) ( 5m, 5m) The grid above shows a simple game between two firms competing for the same customers who must decide whether to launch an expensive advertising campaign

19. Highest UK Advertising Spending in 2014 Spending in million in 2014 0 50 100 150 200 250 British Sky Broadcasting 221 Procter & Gamble 172 BT 146 Unilever UK 140 Asda Stores 107 Tesco 102 Virgin Media 86 Wim Morrison Supermarkets 79 Reckitt Benckiser UK 77 DFS Furniture Co 75 Sainsburys Supermarkets 74 TalkTalk Group 71 McDonald's Restrs 68 Aldi 68 Jd Williams & Co 63

20. First Mover Advantage Two players A and B take turns choosing a number between 1 and 10 (inclusive) A goes first The cumulative number of ALL of the numbers chosen is calculated as the game progresses The winner is the player whose choice of number TAKES THE TOTAL to 100 or more Does this game have first mover advantage?

21. First Mover Advantage 0 to 100 Game For Player A to win, he/she must a number that takes the total to 100 or more The only way this can happen is Player B to leave me a number of 90 or more Player A needs to ensure Player B is left with 89 Player B knows this too Player A needs to leave Player B with 78 Use backward-induction to work towards the solution 100 89 78 67 56 45 34 23 12 Player A can ensure he/she wins by going first and choosing 1

22. Examples of First Mover Advantage? Just Eat Golden Leaf Holdings Oculus Rift Spotify

23. Evaluating First Mover Advantage Advantages of being the first mover A business first into the market can develop a significant competitive advantage through learning by doing - making it difficult and costly for new firms/rivals to enter They can exploit internal economies of scale (leading to lower LRAC) and also build brand loyalty/ repeat demand Consumer behaviour can become habitual hard to eat into! Possible first mover disadvantage? Employees from first mover may leave to set up challenge brands taking some of the intellectual capital with them First movers are often unprofitable, the failure rate can be high Second-movers can learn much from first mover mistakes First-scale advantage may be more important than first mover

24. Game Theory: The Stag Hunt Two hunters Within range is one stag and two hares Both hunters must chase the stag to catch it and share the meat The two hares can be caught individually The meat from one stag > the meat from two hares

25. The Stag Hunt Player 2 Pay-offs from hunting in terms of units of meat Stag Hare Stag (3 , 3) (0, 2) Player 1 Hare (2, 0) (1, 1)

26. The Ultimatum Game 1. The first player (the proposer) receives a sum of money and proposes how to divide the sum between the proposer and the other player 2. The second player (the responder) chooses to either accept or reject this proposal 3. If the second player accepts, the money is split according to the proposal 4. If the second player rejects, neither player receives any money 5. The ultimatum game is often played once what is the equilibrium choice for Player 1? In the basic ultimatum game:

27. The Ultimatum Game - Tweaked The first player (the proposer) receives a sum of money and proposes how to divide the sum between the proposer and the other player The second player (the responder) chooses to either accept or reject this proposal If the second player accepts, the money is split according to the proposal The second player can Reject the offer and end the game Propose a counter-offer

28. Discussing the Ultimatum Game People value things other than money! They care about fairness (equity) The size of the bargaining pie will shape people s willingness to accept a seemingly inequitable offer Repeated games / options to make counter-offers may change the behaviour of players Are players maximisers or satisficers? Professionally trained economists nearly always offer less than normal people!

29. Rock Paper Scissors Nash Equilibrium? In the famous Rock, Paper, Scissors game: Rock > Scissors, Paper > Rock and Scissors > Paper. Is there an optimum strategy when playing this game? Rock Paper Scissors Rock ( 0, 0) (-1 , 1) (1, -1) Paper (1, -1) (0, 0) (-1, 1) Scissors (-1, 1) (1, -1) (0, 0)

30. Rock Paper Scissors Random Strategy Rock Paper Scissors Rock ( 0, 0) (-1 , 1) (1, -1) Paper (1, -1) (0, 0) (-1, 1) Scissors (-1, 1) (1, -1) (0, 0) In this game matter what both players choose, at least one of them can always improve their payoff by switching to a different choice. If one of them wins the game, the loser can improve their payoff by switching. If it's a tie, either player can improve their payoff by switching to a different choice.

31. Evaluating Relevance of Game Theory Game theory becomes relevant to analysing business decision making when there are relatively few firms Standard game theory assumes rational agents are looking to maximise their own self-interest More complex game theory reveals that people / businesses can develop co-operative / collaborative behaviours e.g. the rise of joint ventures / altruism Repeated games are different from one shot games Key evaluation point for the exam: Game theory can over-simplify complex decisions, and when there are more than two rival firms in a market the degree of complexity increases. Many firms fall back on heuristics

32. Key Concepts Game Theory An equilibrium in a game where the players agree to cooperate Cooperative outcome A dominant strategy is one where a single strategy is best for a player regardless of what strategy other players in the game decide to use Dominant strategy Any situation where all participants in a game are pursuing their best possible strategy given the strategies of all of the other participants Nash equilibrium Where firms undertake actions that are likely to minimize a competitive response, e.g. avoiding price-cutting or not attacking each other s market Tacit collusion When one or more agents in a collusive agreement report it to the authorities Whistle blowing An economic transaction in which whatever is gained by one party must be lost by the other. Zero sum game