Factoring Trinomials and Difference of Two Perfect Squares

Explore the concept of factoring trinomials and difference of two perfect squares through step-by-step examples and visual aids. Understand the sign rules for factoring trinomials with positive and negative last terms. Learn how to factor out a greatest common factor and practice factoring various quadratic expressions effectively.

• Factoring
• Trinomials
• Perfect Squares
• Sign Rules
• Quadratic Expressions

fenster_a Follow

Uploaded on Sep 25, 2024 | 0 Views

Download Presentation

Please find below an Image/Link to download the presentation.

The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author. Download presentation by click this link. If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.

Presentation Transcript

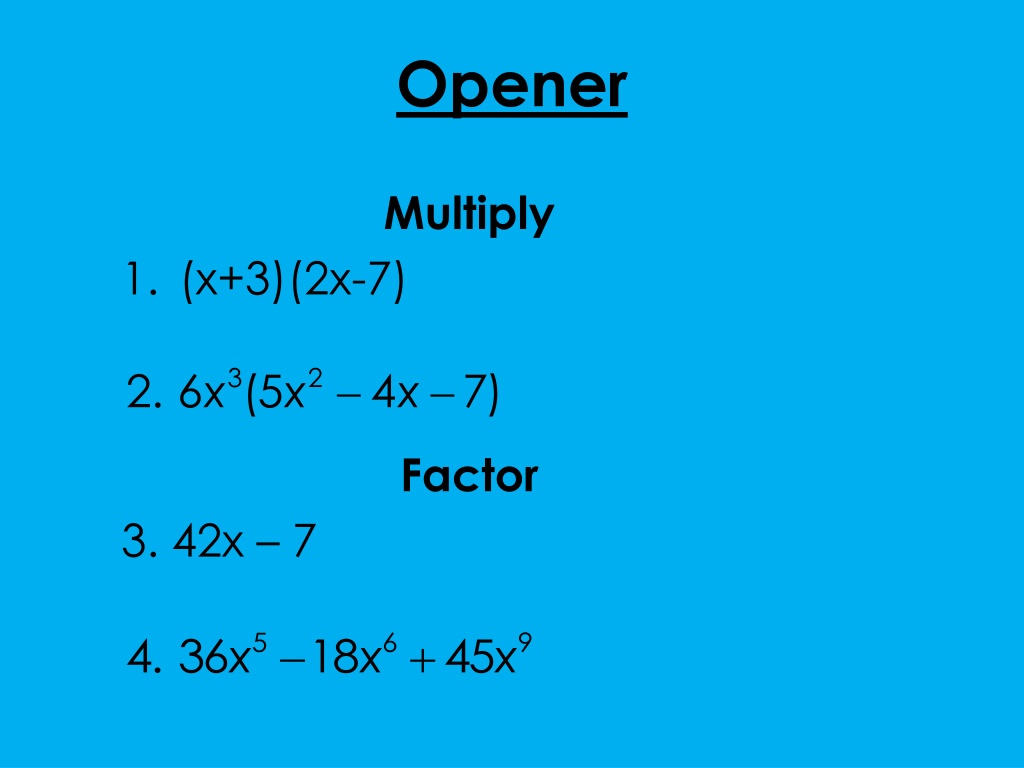
1. Opener Multiply 1. (x+3)(2x-7) 3 2 2. 6 (5 x x 4 x 7) Factor 3. 42x 7 5 6 9 4. 36 x 18 x 45 x +

2. Homework Check

3. Skills Check

4. Factoring Trinomials and Difference of Two Perfect Squares

5. Sign Rule for Factoring Trinomials: When the last term is POSITIVE The signs inside the parenthesis will be the SAME Look at b for the sign Front are factors of a Last factors of c that will add to get b.

6. x2 +7x + 6 ( )( ) x + 6 x + 1

7. x2 + 9x + 14 ( )( ) x + 7 x + 2

8. x2 6x + 8 ( )( ) x 4 x 2

9. Sometimes you can factor out a GCF 1st!

10. x2 10x + 16 ( )( ) x 8 x 2

11. 2x2 16x + 24 2(x2 8x +12) 2( )( ) x 6 x 2

12. Opener.. 3y2 + 36y + 60 3(y +10)(y +2) 4x2 +24x + 32 4(x + 2)(x + 4)

13. Sign Rule for Factoring Trinomials: When the last term is NEGATIVE The parenthesis will have DIFFERENT SIGNS. The larger factor will have the SAME sign as the middle number

14. n2 + 2n 48 ( )( ) n + 8 n 6

15. x2 + 8x 20 ( )( ) x + 10 x 2

16. x2 4x 21 ( )( ) x + 3 x 7

17. x2 9x 36 ( )( ) x + 3 x 12

18. c4 + 2c3 80c2 ( ) 8 + 2 2 2 80 c c c )( ( ) + 2 10 c c c

19. 3x2 + 6x 24 ( ) + 2 3 2 8 x x ( )( ) + 3 4 2 x x

20. Opener Match the problems with the signs of the factors. A.x2 - 6x + 8 i. Both signs are positive. B.x2 + 6x + 8 ii. Both signs are negative. C. x2 - 7x - 8 iii. They have different signs.

21. 2x3 + 18x2 + 28x ( ) + + 2 2 9 14 x x x ( )( ) + + 2 7 2 x x x

22. 5x2 + 5x 10 ( ) + )( 2 2 5 ( 2 x x ) + 5 1 x x

23. 3x3 6x2 45x ( ( ) 2 3 2 )( 15 ) 3 x x x + 3 5 x x x

24. 3x3 39x2 + 120x ( ( ) ) + 2 3 13 )( 5 40 x x x 3 8 x x x

25. Difference of Two Perfect Squares

26. Factoring Difference of Two Squares 1. Both terms must be Perfect Squares and have a MINUS between them 2. Check the binomial for GCF 3. Use two sets of parenthesis (one s a plus, one s a minus) 4. Split up what it takes to make the 1st a perfect square and what it takes the 2nd to be a perfect square

27. Difference of Two Squares Factor 2 1. 25 n ( n = )( ) + 5 5 n 2 ( 2 2. 4 121 x + x y )( ) = 2 11 2 11 y x y

28. Difference of Two Squares Factor 2 3. 196r 1 ( 14 = )( ) + 1 14 1 r r x ( 10 2 4. 100 49 + )( ) = 7 10 7 x x

29. 2x3 162x ( ) x x 2 2 81 ( )( ) + 2 9 9 x x x

30. 16x2 36 ( ) x 2 4 4 9 ( )( ) + 4 2 3 2 3 x x

31. Classwork Worksheet