Exploring Numbers with Eratosthenes' Sieve and Prime Factorization
Eratosthenes, a Greek scholar, developed the Sieve of Eratosthenes to find prime numbers and made significant contributions to mathematics and astronomy. The model shown highlights factors, prime numbers, and square numbers, providing insights into number properties and their relationships. It demonstrates how to identify primes and generate prime factorizations using circles and crossings. The Fundamental Theorem of Arithmetic is also explained, illustrating how any integer can be represented as the product of prime numbers.
Download Presentation

Please find below an Image/Link to download the presentation.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author. Download presentation by click this link. If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.
E N D
Presentation Transcript
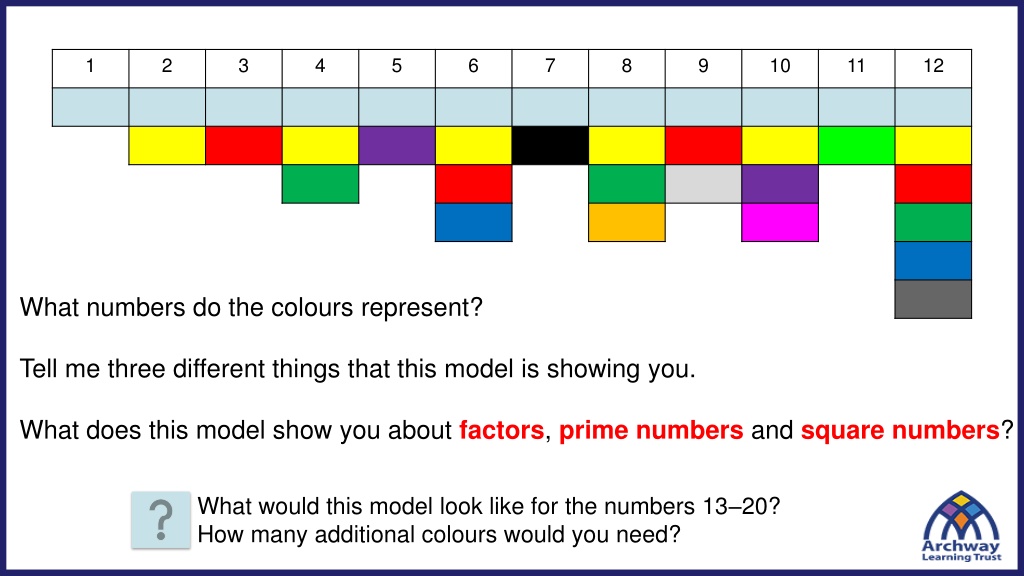
1 2 3 4 5 6 7 8 9 10 11 12 What numbers do the colours represent? Tell me three different things that this model is showing you. What does this model show you about factors, prime numbers and square numbers? What would this model look like for the numbers 13 20? How many additional colours would you need?
Eratosthenes of Cyrene (275-194 B.C) Eratosthenes was a prominent Greek scholar who spent his early life in Athens. He was a friend and contemporary of Archimedes and excelled in many areas, notably mathematics, astronomy, geography, history, poetry and athletics. He was a universal genius who was known to his friends as Beta, because he was regarded as the second best in almost all the fields he studied. He eventually went to Alexandria (Egypt) where he became the 3rd librarian at the great university as well as private tutor to the son of Ptolemy III. It was Eratosthenes who suggested a calendar (later adopted by the Romans) of 365 days with an additional day every 4th year. During old age he went blind and ended his life by drinking poison. 1 2 3 4 5 6 7 8 9 10 11 12 13 14 15 16 17 18 19 20 21 22 23 24 25 26 27 28 29 30 31 32 33 34 35 36 37 38 39 40 41 42 43 44 45 46 47 48 49 50 51 52 53 54 55 56 57 58 59 60 61 62 63 64 65 66 67 68 69 70 71 72 73 74 75 76 77 78 79 80 81 82 83 84 85 86 87 88 89 90 91 92 93 94 95 96 97 98 99 100 He is best remembered today for two notable achievements: The use of his Sieve to isolate prime numbers His ingenious method for determining the distance around the Earth with a high degree of accuracy.
1 2 3 4 5 6 7 8 9 10 Cross out 1 11 12 13 14 15 16 17 18 19 20 21 22 23 24 25 26 27 28 29 30 31 32 33 34 35 36 37 38 39 40 41 42 43 44 45 46 47 48 49 50 51 52 53 54 55 56 57 58 59 60 61 62 63 64 65 66 67 68 69 70 71 72 73 74 75 76 77 78 79 80 81 82 83 84 85 86 87 88 89 90 91 92 93 94 95 96 97 98 99 100 Circle 2 and then cross out all multiples of 2 Circle 3 and then cross out all multiples of 3 Circle the next available number (5) and then cross out all multiples of this number Repeat until all numbers are either circled or crossed out Eratosthenes Sieve
1 2 3 4 5 6 7 8 9 10 25 primes Below 100 11 12 13 14 15 16 17 18 19 20 21 22 23 24 25 26 27 28 29 30 31 32 33 34 35 36 37 38 39 40 41 42 43 44 45 46 47 48 49 50 51 52 53 54 55 56 57 58 59 60 61 62 63 64 65 66 67 68 69 70 71 72 73 74 75 76 77 78 79 80 81 82 83 84 85 86 87 88 89 90 91 92 93 94 95 96 97 98 99 100
The Fundamental Theorem of Arithmetic The Basic Idea The Basic Idea is that any integer above 1 is either a Prime Number, or can be made by multiplying Prime Numbers together, also known as Prime Number Decomposition or Prime Factorisation. So: 2 is prime 3 is prime 4 = 2 x 2 5 is prime 6 is 2 x 3
On your mini whiteboard. Which prime numbers multiply to make 15? ? ?
On your mini whiteboard. Which prime numbers multiply to make 21? ? ?
On your mini whiteboard. Which prime numbers multiply to make 45? ? ? ?
A prime factor tree can help work out the prime factor decomposition of a number
On your mini whiteboard. Complete the prime factor tree
On your mini whiteboard. Express 20 as a productof its s prime factors ? ? ?
On your mini whiteboard. Express 24 as a productof its s prime factors ? ? ? ?
Title: Prime Factorisation Express each of the following as a product of prime factors If you have the CASIO calculator that we recommend, you can actually check your answers on your calculator using the FACT button. a) 300 b) 600 c) 150 d) 450 e) 4500 f) 45 g) 90 h) 270 i) 135 Can you find it? Press your number, then the = button. Now press SHIFT then FACT. Use it to check your answers for question 3.
To finish n was written as the product of its prime factors. ? = 2 2 3 5 7 Is n divisible by 10? 6? 8? How many other numbers can you find that n is divisible by?