Evolution of Communication Systems: From Bandwidth Division to Spread Spectrum
Third-generation communication systems utilize Pseudo-Noise (PN) codes to share bandwidth without interference, while first and second-generation systems divide bandwidth into smaller channels. PN codes are vectors with 1s and -1s, orthogonal to each other. Users transmit data using PN coding, combining user data with respective PN codes. Spread spectrum systems employ carrier modulation and PN modulation stages, increasing system bandwidth. Frequency Hopping Spread Spectrum (FHSS) originated during World War II for naval ships to evade detection by enemy submarines through frequency hopping with PN codes.
Download Presentation

Please find below an Image/Link to download the presentation.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author. Download presentation by click this link. If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.
E N D
Presentation Transcript
First and second generation communication systems divide into smaller bandwidth channels divide the total available bandwidth However, third generation systems share entire bandwidth without mutual interference share the This is possible through the use of Pseudo Noise ( Pseudo- - Noise (PN) codes PN) codes
PN codes are vectors with a combination of 1s and -1s: Example: [1 1 -1 -1] an [1 -1 1 -1] PN codes are orthogonal different codes is zero orthogonal to each other, i.e. dot product of two Example: [1 1 -1 -1] . [1 -1 1 -1] = (1)(1) + (1)(-1) + (-1)(1) + (-1)(-1) = 0 Dot product of two identical codes is equal to the length code length of the Example: [1 1 -1 -1] . [1 1 -1 -1] = (1)(1) + (1)(1) + (-1)(-1) + (-1)(-1) = 4
User Data User A data: 12 User B data: 7 User C data: -10 PN codes A: B: C: [1 1 1 1] [1 -1 1 -1] [1 1 -1 -1] Transmission coding User 1 : User 1 Data x User 1 code = 12 x [1 1 1 1] = [12 12 12 12] User 2 : User 2 Data x User 2 code = 7 x [1 -1 1 -1] = [7 -7 7 -7] User 3 : User 3 Data x User 3 code = -10 x [1 1 -1 -1] = [-10 -10 10 10] Transmitted data = Sum of user data = [12 12 12 12] + [7 -7 7 -7] + [-10 -10 10 10] = [9 -5 29 15]
Received User 1 data = Combined data . . User 1 code Code Length = [9 -5 29 15] . . [1 1 1 1] /4 = 48/4 = 12 Received User 2 data = Combined data . . User 2 code Code Length = [9 -5 29 15] . . [1 -1 1 -1]/4 = 28/4 =7 Received User 3 data = Combined data . . User 3 code Code Length = [9 -5 29 15] . . [1 1 -1 -1]/4 = -40/4 =-10
Spread spectrum systems have two modulation (at transmitter) and demodulation (at receiver) two stages of First stage is carrier modulation /demodulation as in 1G and 2G systems Second stage is PN modulation/demodulation which increases increases the bandwidth of the system
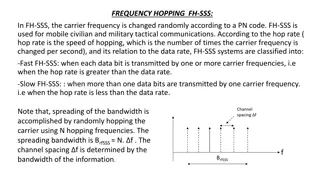
FHSS evolved during World War II, primarily as a technique for naval ships to avoid detection by enemy submarines Ships would keep changing the radio signal frequency or hopping frequencies hopping through a set of PN Codes would help design orthogonal frequency sets to avoid interference
Processing Gain PG= System bandwidth/Channel bandwidth = W/B FHSS bandwidth BWFHSS = PGBFSK FHSS BER for M users
DSSS is simpler type of Spread Spectrum using PN coding Developed by Qualcomm in 1995 as CDMA (Code Division Multiple Access) CDMA (Code Division Multiple Access) Initial system was called IS-95 which led on to CDMA2000 and W-CDMA
DSSS coding multiplies the data data with the PN code PN code Data is random (bits sequence (chips bits) but PN code is repeating chips) Each user has a distinct distinct PN code
Processing Gain PG = Data period/Chip period = T/Tc DSSS bandwidth BWDSSS = PG BPSK DSSS BER for M users
Spread spectrum systems have higher noise immunity and fading due to large bandwidth noise immunity Pseudo-random nature of the PN sequence provides increased signal signal security security Lower jamming power density Lower jamming effects on system due to lower High bandwidth requires wideband circuitry wideband system models wideband circuitry and wideband system models Spread Spectrum systems are more complex 2-stage modulation systems; complex due to