Binomial Distribution in Statistics

Exploring the concepts of binomial distribution in statistics through practical examples and analyses of combined test scores, probabilities, and experimental properties. Dive into the fundamentals of binary outcomes, independence, fixed trials, and constant probabilities. Challenge your understanding with scenarios involving student performance, gaming, and random events.

• Statistics
• Binomial Distribution
• Probability
• Experiments

mysterma Follow

Uploaded on Sep 14, 2024 | 1 Views

Download Presentation

Please find below an Image/Link to download the presentation.

The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author.If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.

You are allowed to download the files provided on this website for personal or commercial use, subject to the condition that they are used lawfully. All files are the property of their respective owners.

The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author.

Presentation Transcript

1. Homework Work on project Agenda Warm Up Check-up Check, copies Lecture, binomial Mean, s.d. of binomial Casino game Exit Pass 10 min 10 min 45 min 20 min 10 min 5 min

2. Warm Up (AP) Mr. Colligan s students score an average of 79.3% on tests, with =8.2%, while Ms. Tan s class scores an average of 73.5% on tests, with =9.4%. Several students are enrolled in both Mr. Colligan sand Ms. Tan s class. tinyurl.com/Colligan-may-2020 1. What is the mean and standard deviation of these students combined test scores? 2. Assuming the test scores are Normal, what is the probability that one of these students has a combined test score of 180% or more? 3. Challenge. What is the probability that a student gets a higher score in Mr. Colligan s class than Ms. Tan s class?

3. Warm Up (AP) ANSWERS Mr. Colligan s students score an average of 79.3% on tests, with =8.2%, while Ms. Tan s class scores an average of 73.5% on tests, with =9.4%. Several students are enrolled in both Mr. Colligan s and Ms. Tan s class. 1. What is the mean and standard deviation of these students combined test scores? 3 . 79 + = 73 5 . 152 + 8 . = 2 2 2 . 8 4 . 9 12 47 . 2. Assuming Normality, what is the probability that one of these students has a combined test score of 180% or more? 0146 . 0 180 152 8 . = . 2 18 12 47 . 3. Challenge. What is the probability that a student gets a higher score in Mr. Colligan s class than Ms. Tan s class? . 0 47 . 12 8 . 5 0 6772 . 0 = 46

4. Checkup time

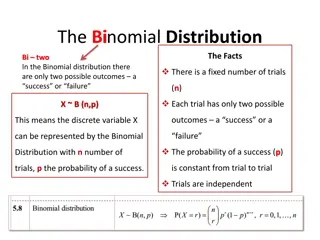
5. Notes Binomial 1 of 4 Properties of a binomial experiment: 1. B inary? Only 2 outcomes for each trial: success or failure. 2. I ndependent? Trials are independent. 3. N umber? Fixed number (n) of trials. 4. S uccess? Probability of success (p) is constant. 10 N n Assume yes when the population (N) is big enough. Is it binomial? a. The numberof children out of 5 children who inherit a particular blood type from their parents. b. Deal 10 cards from a fair deck and count the number of red cards. c. An engineer chooses a SRS of 10 switches from a shipment of 10,000 switches, where 10% of the total population of switches are bad. The engineer counts the number of bad switches in the sample.

6. Window/Door Is it binomial? If not, explain why not ..which of the four conditions does it not meet? 1. The number of heads in 20 flips of a weighted coin, where P(head) = 1/3. 2. The number of females births at Kaiser Permanente in Sacramento next week. 3. The number of odd numbers rolled in 10 rolls of a six-sided die and 10 rolls of a four-sided die. 4. The number of spades in five cards selected from a standard deck. 5. The number of students who pass a final exam. 6. The number of red cards out of 10 cards dealt from a standard deck.

7. Window/Door Is it binomial? If not, explain why not ..which of the four conditions does it not meet? 1. The number of heads in 20 flips of a weighted coin, where P(head) = 1/3. Yes. 2. The number of females births at Kaiser Permanente in Sacramento next week. No. Not fixed number of trials. 3. The number of odd numbers rolled in 10 rolls of a six-sided die and 10 rolls of a four-sided die. 4. The number of spades in five cards selected from a standard deck. Yes. N>10n. 5. The number of students who pass a final exam. No. Not fixed number of trials. 6. The number of red cards out of 10 cards dealt from a standard deck. No. Probability not constant (or independent). Yes.

8. Notes 2 of 4 Binomial on Calculator x is number of successesin n trials, with probability of success p. 2nd, Distr .. binompdf (n,p,x) OR binomcdf (n,p,x) At most x successes in n trials Exactly x successes in n trials Examples: Roll 7 dice, get exactly three 3 s. Roll 7 dice, get at most three 3 s. binompdf (7, 1/6, 3) = 0.078 binomcdf (7, 1/6, 3) = 0.982 binomcdf (7, 1/6, 2) = 0.904 1 0.904 = 0.095 Roll 7 dice, get at least three 3 s.

9. Notes 2 of 4 Binomial on Calculator x is number of successesin n trials, with probability of success p. 2nd, Distr .. binompdf (n,p,x) OR binomcdf (n,p,x) At most x successes in n trials Exactly x successes in n trials Examples: Roll 7 dice, get exactly three 3 s. Roll 7 dice, get at most three 3 s. binompdf (7, 1/6, 3) = 0.078 binomcdf (7, 1/6, 3) = 0.982 binomcdf (7, 1/6, 2) = 0.904 1 0.904 = 0.095 Roll 7 dice, get at least three 3 s.

10. Window/Door Find the probability of the following: 1. Flip a coin 50 times, getting 30 Heads. 2. Roll a die 15 times, getting five 6 s. 3. Roll a die 15 times, getting any 6 s. (Hint: any = at least one ) 4. Flip a coin 50 times, getting at least 30 Heads.

11. Window/Door ANSWERS (calculator) 1. Flip a coin 50 times, getting 30 Heads. 1 , 50 ( = binompdf 30 , ) . 0 042 2 2. Roll a die 15 times, getting five 6 s. 1 = 15 ( , ) 5 , 6 . 0 062 binompdf 3. Roll a die 15 times, getting any 6 s. (Hint: any = at least one ) 1 , 15 ( 1 binomcdf = ) 0 , 6 . 0 935 4. Flip a coin 50 times, getting at least 30 Heads. 1 binomcdf = 1 50 ( , , 29 ) . 0 101 2

12. Binomial Formula n x n x 1 ( ) p p k number of successes n trials p probability of success x Examples: Roll 7 dice, get exactly three 3 s. 7 1 1 3 7 3 ( ) 1 ( ) 3 6 6 Roll 7 dice, get at most three 3 s. 1 1 ( ) 6 7 1 7 1 1 7 1 1 7 1 1 3 7 3 ( ) + + 1 7 1 2 7 2 ( ) 1 ( ) ( ) 1 ( ) + 0 7 0 ( ) 1 ( ) 3 6 1 6 6 2 6 6 0 6 6

13. Example: FRQ 2011 #3 An airline claims that there is a 0.10 probability that a coach-class ticket holder who flies frequently will be upgraded to first class on any flight. This outcome is independent from flight to flight. Sam is a frequent flier who always purchases coach-class tickets. a. What is the probability that Sam s first upgrade will occur after the third flight? b. What is the probability that Sam will be upgraded exactly 2 times in his next 20 flights? ) 2 , 10 . 0 , 20 ( = binompdf 20 trials (n) 0.10 probability (p) 2 successes (x) . 0 285 c. Sam will take 104 flights next year. Would you be surprised if Sam receives more than 20 upgrades to first class during the year? Justify your answer.

14. Notes Mean and s.d. of Binomial 3 of 4 If you roll a die 900 times, how many three s do you expect? How much variability? np = = np 1 ( ) p Example: If you roll a die 900 times, and get 175 three s, do you conclude that the die is weighted? 150 ) 6 / 1 )( 900 ( = = = = 900 ( / 1 )( / 5 )( 6 ) 6 11 18 . 175 150 1 = . 2 24 = 1 binomcdf 900 ( , 175 , ) 01247 . 0 11 18 . 6 = 1 9875 . 0 0125 . 0

15. Example: FRQ 2011 #3 An airline claims that there is a 0.10 probability that a coach-class ticket holder who flies frequently will be upgraded to first class on any flight. This outcome is independent from flight to flight. Sam is a frequent flier who always purchases coach-class tickets. c. if Sam receives more than 20 upgrades to first class during the year? Justify your answer. np = 4 . 10 ) 1 . 0 )( 104 ( = = 4 . 10 20 = Sam will take 104 flights next year. Would you be surprised = = np 1 ( 104 ( ) p = 1 . 0 )( ) 9 . 0 )( . 3 059 . 3 14 = 1 binomcdf 104 ( , 1 . 0 , 20 ) . 3 059 0014 . 0 = 1 9991 . 0 0009 . 0

16. Notes Z-scores & Binomials 4 of 4 Assume Normality when sample size (n) is big enough. 10 np p 1 ( n ) 10 Assume Independence when population size (N) is big enough. N 10 n

17. Next slide I ve got three problems on the next slide, ordered by difficulty. Choose one of them.

18. Good, bad, ugly 1. Offspring of a particular genetic cross have an undesirable trait with probability 1/8. Inheritance of this trait by separate offspring is independent. You examine 100 offspring from this cross and count the number X who have the undesirable trait. What is the probability that 10 or fewer of the offspring have this trait? 2. Are attitudes towards shopping changing? Sample surveys show that fewer people enjoy shopping than in the past. A survey asked a nationwide random sample of 2500 adults if they agreed or disagreed that I like buying new clothes, but shopping is often frustrating and time-consuming. Suppose that in fact 60% of all adult U.S. residents would say Agree if asked the same question. What is the probability that 1520 or more of the sample agree? 3. Is the coin fair? A coin is flipped 25 times. Let X represent the number of heads. The coin is judged to be fair if X is between 8 and 17, inclusive, and biased if X is outside this interval. What is the probability that the coin is judged to be biased when it is, in fact, fair ( false positive )?

19. Good, bad, ugly 1. Offspring of a particular genetic cross have an undesirable trait with probability 1/8. Inheritance of this trait by separate offspring is independent. You examine 100 offspring from this cross and count the number X who have the undesirable trait. What is the probability that 10 or fewer of the offspring have this trait? Independence is assumed, we assume N>1,000 .that the population of offspring with this trait more than 10 times the sample size. Normality is met. np=12.5 and n(1-p)=87.5, both greater than 10. 1 = = 100 ( )( ) 12 5 . np 8 1 7 p = = 1 ( ) 100 ( )( )( ) . 3 31 np 8 8 2236 . 0 10 12 5 . = . 0 76 . 3 31

20. Good, bad, ugly 1. Offspring of a particular genetic cross have an undesirable trait with probability 1/8. Inheritance of this trait by separate offspring is independent. You examine 100 offspring from this cross and count the number X who have the undesirable trait. What is the probability that 10 or fewer of the offspring have this trait? 1 , 100 ( binomcdf = 10 , ) . 0 281 8 100 1 7 100 1 7 + + 10 90 0 100 ) ( ) ( ) .... ( ) ( 10 8 8 0 8 8

21. 2. Are attitudes towards shopping changing? Sample surveys show that fewer people enjoy shopping than in the past. A survey asked a nationwide random sample of 2500 adults if they agreed or disagreed that I like buying new clothes, but shopping is often frustrating and time-consuming. Suppose that in fact 60% of all adult U.S. residents would say Agree if asked the same question. What is the probability that 1520 or more of the sample agree? Independence is met. N>25,000 .the population of American adults is more than 10 times the sample size. Normality is met. np=1500 and n(1-p)=1000, both greater than 10. = = ( 2500 . 0 )( 60 ) 1500 np p = = 1 ( ) ( 2500 . 0 )( 60 . 0 )( 40 ) 24 5 . np 1520 1500 7939 . 0 = = 1 2061 . 0 . 0 82 24 5 .

22. Good, bad, ugly 2. Are attitudes towards shopping changing? Sample surveys show that fewer people enjoy shopping than in the past. A survey asked a nationwide random sample of 2500 adults if they agreed or disagreed that I like buying new clothes, but shopping is often frustrating and time-consuming. Suppose that in fact 60% of all adult U.S. residents would say Agree if asked the same question. What is the probability that 1520 or more of the sample agree? 1519 , 60 . 0 , 2500 ( binomcdf = ) 7868 . 0 7868 . 0 = 1 2132 . 0 2500 2500 + + 1520 980 2500 0 ) 6 (. ) 4 (. .... ) 6 (. ) 4 (. 1520 2500

23. 3. Is the coin fair? A coin is flipped 25 times. Let X represent the number of heads. The coin is judged to be fair if X is between 8 and 17, inclusive, and biased if X is outside this interval. What is the probability that the coin is judged to be biased when it is, in fact, fair ( false positive )? Independence is met. Contextually, coin flips are independent. Also, N>250. Normality is met. np=12.5 and n(1-p)=12.5, both greater than 10. 1 1 1 = = p = = ( 25 )( ) 12 5 . np 1 ( ) ( 25 )( )( ) 5 . 2 np 2 2 2 8 12 5 . 17 12 5 . = 8 . 1 8 . 1 = 5 . 2 5 . 2 0359 . 0 9641 . 0 0359 . 0 = 9641 . 0 9282 . 0 9282 . 0 = 1 0718 . 0

24. Good, bad, ugly 3. Is the coin fair? A coin is flipped 25 times. Let X represent the number of heads. The coin is judged to be fair if X is between 8 and 17, inclusive, and biased if X is outside this interval. What is the probability that the coin is judged to be biased when it is, in fact, fair ( false positive )? 1 , 25 ( = binomcdf 1 binomcdf ) 7 , 2 0216 . 0 1 = ( 25 , 17 , ) 0217 . 0 2 + = 0216 . 0 0217 . + 0433 . 0 25 25 + 7 18 0 25 (. 5 ) (. 5 ) .... (. 5 ) (. 5 ) + .... + 7 0 25 25 + 17 8 25 0 (. 5 ) (. 5 ) (. 5 ) (. 5 ) 17 25

25. Casino Project (AP) Create, mathematically analyze, and run a simple gambling game which is significantly (but not obviously) in favor of you, The House . 1-2 people (2 recommended) Up to +5% extra credit for binomial probabilities and/or confidence intervals. Monday March 9th, due end of class: 1. List three possible ideas for your project. For each game, state how to play and how to win. Wednesday March 18th (or earlier), due end of class: 2. Choose one game and give it a catchy name. Describe your game (including the title), and calculate its probabilities and expected value. Tuesday March 24th: 3. Bring an attractive poster that explains your game, including prices and prizes. Bring all components needed for your game. Thursday March 26th: CASINO DAY! 7. Run your game, attract gamblers, and help them lose their money! You must profit at least $500 ($1000 total).

26. Homework Work on project Exit Pass Each child born to a particular set of parents has probability 0.25 of having blood type O. The parents have 5 children. What is the probability that . 1. Exactly 2 of their children have type O blood? 2. None of their children have type O blood? 3. At least 1 of their children has type O blood? tinyurl.com/Colligan-may-2020