Analysis and Comparison of Wave Equation Prediction for Propagating Waves

Initial analysis and comparison of the wave equation and asymptotic prediction of a receiver experiment at depth for one-way propagating waves. The study examines the amplitude and information derived from a wave equation migration algorithm and its asymptotic form. The focus is on the prediction of the receiver experiment at depth, including Green's theorem/FK prediction, the exact Cagniard-deHoop solution, and the asymptotic output. The theory, numerical tests, and a summary are outlined for wave equation prediction and its corresponding asymptotic form for one-way up-going waves. Various equations and formulas are discussed in detail, shedding light on the mathematical aspects of wave propagation in heterogeneous media.

• Wave Equation
• Propagating Waves
• Asymptotic Prediction
• Receiver Experiment
• Depth

chr_plu Follow

Uploaded on Nov 19, 2024 | 0 Views

Download Presentation

Please find below an Image/Link to download the presentation.

The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author. Download presentation by click this link. If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.

Presentation Transcript

1. Initial analysis and comparison of the wave equation and asymptotic prediction of a receiver experiment at depth for one- way propagating waves Chao Ma*, Jing Wu and Arthur B. Weglein 1 M-OSRP 2014 Annual Meeting, May, 2014 Austin, TX

2. Motivation Qiang et al (2014) examine the amplitude information at the image that derives from a wave equation migration algorithm and its corresponding asymptotic form. The asymptotic form follows from a stationary phase approximation performed on the operator that predicts the receiver and source experiment at depth. We examine the prediction of the receiver experiment at depth: Green s theorem/FK prediction The exact Cagniard-deHoop (CdH) solution The asymptotic output. 2

3. Outline Theory Wave equation prediction and its corresponding asymptotic form for one way waves Numerical test Summary 3

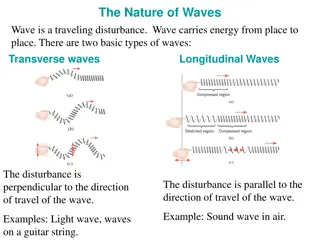
4. Wave equation prediction for one way up-going waves Stolt (1978), Stolt and Benson (1986), Stolt and Weglein (2012) Assuming an up-going wave, and homogeneous medium ( , | , ; ) D x z x z t g g s s ( ) ik x i t , e dx e dt g g g , ; ) s x z ( , | D k z g g s 2 g 2 k c z z = ( ) ik 1 ; ) = ; ) k ( , | , ( , | , D k z x z D k z x z e z g z 2 g s s g g s s c 1 ik x ; ) = ; )e W ( , | x z x z , ( , | , D D k z x z dk g s s g s s g 2 4 1 i t = ; ) W W ( , | x z x z t , ; ) ( , | x z x z , D D e d s s s s 2

5. Asymptotic form of the wave equation prediction for one way up-going waves 1 z z ( ) ik x ik ik x = W ( , | x z x z , ; ) s ( , | , ; ) s x z D D x z e dx e e dk g g z g g s g g s g g 2 1 z z + x x [ ( ) ( )] i k k = ( , | , ; ) s x z D x z dx e dk z g g g g g s g g 2 5

6. Asymptotic form of the wave equation prediction for one way up-going waves 1 z z ( ) ik x ik ik x = W ( , | x z x z , ; ) s ( , | , ; ) s x z D D x z e dx e e dk g g z g g s g g s g g 2 1 z z + x x [ ( ) ( )] i k k = ( , | , ; ) s x z D x z dx e dk z g g g g g s g g 2 2 2 ( cr ) i z z 1 gr c ( , | x z x z / A g , ; ) D ( , | , ; ) s x z D x z dx e s s g g s g 3 g 2 ) ( 6 = + 2 2 ( ) ( ) r z z x x g g g

7. Asymptotic form of the wave equation prediction for one way up-going waves 1 z z ( ) ik x ik ik x = W ( , | x z x z , ; ) s ( , | , ; ) s x z D D x z e dx e e dk g g z g g s g g s g g 2 1 z z + x x [ ( ) ( )] i k k = ( , | , ; ) s x z D x z dx e dk z g g g g g s g g 2 2 g 2 k c = 1 k z 2 c 2 2 ( cr ) i z z 1 gr c ( , | x z x z / A g , ; ) D ( , | , ; ) s x z D x z dx e s s g g s g 3 g 2 ) ( ( ) ( ) x x z z 7 g g = = = + 2 2 , , ( ) ( ) k k r z z x x g z g g g c r c r g g

8. Asymptotic form of the wave equation prediction for one way up-going waves 1 z z ( ) ik x ik ik x = W ( , | x z x z , ; ) s ( , | , ; ) s x z D D x z e dx e e dk g g z g g s g g s g g 2 1 z z + x x [ ( ) ( )] i k k = ( , | , ; ) s x z D x z dx e dk z g g g g g s g g 2 2 g 2 k c Non-linear = 1 k z 2 c 2 2 ( cr ) i z z 1 gr c ( , | x z x z / A g , ; ) D ( , | , ; ) s x z D x z dx e s s g g s g 3 g 2 ) ( ( ) ( ) x x z z 8 g g = = = + 2 2 , , ( ) ( ) k k r z z x x Linear g z g g g c r c r g g

9. Outline Theory Wave equation prediction and its corresponding asymptotic form for one way waves; Numerical test Summary 9

10. Numerical test comparing a wave equation prediction of a receiver experiment at depth and its asymptotic form Model: -20,000 m to 20,000 m (dx=4m) Input data at depth 400 m sz = (0, 0) x z = ( , 400) = CdH ( , 400|0, , ) D x z z t g g g g s = 2000 / c m s 0 Reflector depth 2000 m = 1000 / c m s 1 10 Tmax=5 s, dt=1 ms;

11. Numerical test comparing a wave equation prediction of a receiver experiment at depth and its asymptotic form sz = (0, 0) Input data at depth 400 m Predicted depth 600 m x z = ( , 400) g g = CdH ( , 400|0, , ) D x z z t g g s = 2000 / c m s 0 Reflector depth 2000 m = 1000 / c m s 1 Input Calculate = = z W W ( , x z 600|0, , ) ( , x z 600|0, , ) D z t D 11 s s = CdH ( , 400|0, , ) D x z z t g g s = = z A A ( , x z 600|0, , ) ( , x z 600|0, , ) D z t D s s

12. Numerical test comparing a wave equation prediction of a receiver experiment at depth and its asymptotic form Comparison (0m offset and 2000m offset) = = z CdH CdH ( , x z 600|0, , ) ( , x z 600|0, , ) D z t D s s = = z W W ( , x z 600|0, , ) ( , x z 600|0, , ) D z t D s s = = z A A ( , x z 600|0, , ) ( , x z 600|0, , ) D z t D s s 12

13. Compare (0 m) Zero-offset space-time = CdH ( , x z 600|0, , ) D z t s = W ( , x z 600|0, , ) D z t s = A ( , x z 600|0, , ) D z t s 13

14. Compare (0 m) Zero-offset space-time (zoom-in of the plot in the last slide) = CdH ( , x z 600|0, , ) D z t s = W ( , x z 600|0, , ) D z t s = A ( , x z 600|0, , ) D z t s 14

15. Compare (0 m) Zero-offset space-frequency = z CdH ( , x z 600|0, , ) D s = z W ( , x z 600|0, , ) D s = z A ( , x z 600|0, , ) D s 0.04 CdH accurate data Asymptotic prediction Wave prediction 0.035 0.03 0.025 Amplitude 0.02 0.015 0.01 0.005 15 0 0 10 20 30 40 50 60 Frequency/Hz

16. Compare (0 m) Zero-offset space-frequency (zoom-in of the plot in the last slide) = z CdH ( , x z 600|0, , ) D s = z W ( , x z 600|0, , ) D s = z A ( , x z 600|0, , ) D s 0.04 CdH accurate data Asymptotic prediction Wave prediction 0.035 0.03 0.025 Amplitude 0.02 0.015 0.01 0.005 16 0 0 2 4 6 8 10 12 14 16 18 20 Frequency/Hz

17. Compare (2000 m) 2000 m-offset space-time = CdH ( , x z 600|0, , ) D z t s = W ( , x z 600|0, , ) D z t s = A ( , x z 600|0, , ) D z t s 17

18. Compare (2000 m) 2000 m-offset space-time (zoom-in of the plot in the last slide) = CdH ( , x z 600|0, , ) D z t s = W ( , x z 600|0, , ) D z t s = A ( , x z 600|0, , ) D z t s 18

19. Compare (2000 m) 2000 m-offset space-frequency (zoom-in of the plot in the last slide) = z CdH ( , x z 600|0, , ) D s = z W ( , x z 600|0, , ) D s = z A ( , x z 600|0, , ) D s 0.04 CdH accurate data Asymptotic prediction Wave prediction 0.035 0.03 0.025 Amplitude 0.02 0.015 0.01 0.005 19 0 0 10 20 30 40 50 60 Frequency/Hz

20. Compare (2000 m) 2000 m-offset space-frequency (zoom-in of the plot in the last slide) = z CdH ( , x z 600|0, , ) D s = z W ( , x z 600|0, , ) D s = z A ( , x z 600|0, , ) D s 0.04 CdH accurate data Asymptotic prediction Wave prediction 0.035 0.03 0.025 Amplitude 0.02 0.015 0.01 0.005 20 0 0 2 4 6 8 10 12 14 16 18 20 Frequency/Hz

21. Outline Theory Wave equation prediction and its corresponding asymptotic form for one way waves; Numerical test Summary 21

22. Summary The difference in the spectrum at the low end has a dramatic impact on subsequent imaging step, and makes the asymptotic migration method NOT an approximate source and receiver coincident experiment at time equals zero. 22

23. 23