Advanced Techniques for Mathematical Integration

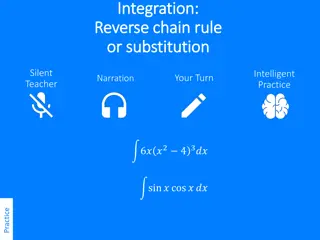
Teachings for Exercise 11D

If: ? = ??? Integration Then: ?? 1 ??= ? When integrating some equations, you might need to use the chain rule, but in reverse Let: ? = ??? Inverse Logarithm ? = ?? In the differentiation chapter, you learnt how to differentiate ? = ??? Differentiate ?? ??= ?? ?? ?? = 1 ?? ?? ?? ??= 1 ?? Earlier we said that ? = ?? ?? ??=1 ? 11D

If: ? = ??? Integration Then: ?? 1 ??= ? If: ? = ???(?) ??=? (?) Then: ?? ?(?) But what about if the logarithm was a function of x? Let: ? = ???(?) Let ? = ?(?) ? = ?(?) ? = ??? Differentiate (we know this result from before) Differentiate ?? ??= ? (?) ?? ??=1 ? ?? ??=?? ?? ?? ?? Sub in values ?? ??=1 ? (?) ? ? = ?(?) ?? ??= ?? ??=? (?) 1 ?(?)? (?) ?(?) 11D

If: ? = ??? Integration Then: ?? 1 ??= ? If: ? = ???(?) ??=? (?) Then: ?? ?(?) When integrating some equations, you might need to use the chain rule, but in reverse You can use this pattern to help integrate functions of the form: ?? (?) ?(?) You should be checking if the denominator would differentiate to give the numerator It will still work if the differential would then have to be multiplied by the constant 11D

If: ? = ??? Integration Then: ?? 1 ??= ? If: ? = ???(?) ??=? (?) Then: ?? ?(?) When integrating some equations, you might need to use the chain rule, but in reverse ? = ?? ?2+ 1 Differentiate using the relationship above ?? ??= 2? ?2+ 1 Find: Since this gives us exactly what we are trying to integrate, it must follow that: 2? ?? ?2+ 1 2? ?? ?2+ 1 Integrate (remember you should include the modulus sign!) Notice that the denominator will differentiate to give the numerator = ?? ?2+ 1 + ? So start by checking if ? = ??(???????????)will work 11D

If: ? = ??? Integration Then: ?? 1 ??= ? If: ? = ???(?) ??=? (?) Then: ?? ?(?) When integrating some equations, you might need to use the chain rule, but in reverse ? = ?? 3 + 2???? Differentiate using the relationship above ?? ??= 2???? 3 + 2???? Find: Since this gives us double what we are looking for, we need to halve the original guess ???? ?? 3 + 2???? Therefore ???? Notice that the denominator will differentiate to give the numerator (albeit also being multiplied by 2 ) ?? 3 + 2???? Integrate, including the modulus sign =1 2?? 3 + 2???? + ? So start by checking if ? = ??(???????????)will work 11D

Integration When integrating some equations, you might need to use the chain rule, but in reverse You can use a similar method when integrating functions of the form: ? = ?? (?) ?(?)? Function raised to a power A constant The derivative of that function So you should look for two functions multiplied together, where one is the derivative of the other (usually one will be raised to a power as well) 11D

Integration When integrating some equations, you might need to use the chain rule, but in reverse Find: 3???????2? ?? So notice here that sine differentiates to cosine, and they are multiplied together In most cases, try starting with a power one higher than in the function you are integrating You should start by differentiating ? = ???3?, and see what happens 11D

Integration ? = ???3? When integrating some equations, you might need to use the chain rule, but in reverse Rewrite for differentiation ? = ????3 Differentiate using the chain rule ?? ??= 3 ????2(????) Find: Simplify ?? ??= 3???2????? 3???????2? ?? Notice that this has given us the exact expression we are trying to integrate So notice here that sine differentiates to cosine, and they are multiplied together Therefore: 3???????2? ?? Integrate You should start by differentiating ? = ???3?, and see what happens = ???3? + ? 11D

Integration When integrating some equations, you might need to use the chain rule, but in reverse Find: ? ?2+ 53 ?? In most cases, try starting with a power one higher than in the function you are integrating Notice that if we differentiate the inner bracket, we will get the term outside (multiplied by 2) The terms are also multiplied together Start by differentiating ? = ?2+ 54 and see what happens 11D

Integration ? = ?2+ 54 When integrating some equations, you might need to use the chain rule, but in reverse Differentiate using the chain rule ?? ??= 4 ?2+ 53(2?) Simplify ?? ??= 8? ?2+ 53 Find: ? ?2+ 53 ?? Notice that this has given us the expression we were trying to integrate, but has been multiplied by 8 Notice that if we differentiate the inner bracket, we will get the term outside (multiplied by 2) Therefore, when integrating we need to divide the original guess by 8 ? ?2+ 53 ?? The terms are also multiplied together Integrate =1 8?2+ 54+ ? Start by differentiating ? = ?2+ 54 and see what happens 11D

Integration When integrating some equations, you might need to use the chain rule, but in reverse Use integration to find: ?????2? 2 + ????3 ?? Be careful with this one. It looks like we should use the rule involving ln, but actually the denominator will not differentiate to give the numerator We need to rewrite this as solve it as with the previous question 11D

Integration When integrating some equations, you might need to use the chain rule, but in reverse ? = 2 + ???? 2 Differentiate using the chain rule ?? ??= 2 2 + ???? 3 ?????2? The negatives cancel out ?? ??= 2 ?????2? 2 + ???? 3 Use integration to find: Rewrite as a fraction 2?????2? 2 + ????3 ?? ??= ?????2? 2 + ????3 ?? Notice that this has given us the expression we were trying to integrate, but has been multiplied by 2 Try rewriting it Therefore, when integrating we need to divide the original guess by 2 = ?????2? 2 + ???? 3 ?? ?????2? 2 + ????3 ?? Integrate Now try differentiating ? = 2 + ???? 2 and see what happens =1 22 + ???? 2+ ? 11D

Integration ? = ???4? When integrating some equations, you might need to use the chain rule, but in reverse Write for differentiation ? = ????4 Differentiate using the chain rule ?? ??= 4 ????3???????? Given that: 5???????4? ?? =15 Simplify ? ?? ??= 4???????4? 4 0 This has given us the expression we are looking for, but only 4 Where 0 < ? <? 2, find the exact value of ?. 5 of it We therefore need to multiply the original guess by 5 4 Notice above, that when you differentiate ????, you get ???? in the answer This means that for ????, you should try differentiating it with the same power as in the answer 5??????4? ?? Integrate =5 4???4? + ? 11D

Integration ? When integrating some equations, you might need to use the chain rule, but in reverse 5???????4? Integrate as we showed previously (we do not need to include the +c for definite integration) 0 ? 5 4???4? = 0 Write as a subtraction Given that: 5???????4? ?? =15 5 4???4? 5 4???4(0) ? = Remember that ???? = 4 1 0 5 5 ???? = 4 ????4 4 ???04 Calculate using ???0 Where 0 < ? <? 2, find the exact value of ?. 5 5 4 = 4 ????4 Notice above, that when you differentiate ????, you get ???? in the answer This means that for ????, you should try differentiating it with the same power as in the answer Since we know that this is equal to 15 equal to that, and solve for ? 4, we can set it 11D

Integration 4 ????4 5 5 4=15 When integrating some equations, you might need to use the chain rule, but in reverse 4 Add 5 4 to both sides 5 4 ????4= 5 Multiply both sides by 4 ????4 Given that: 5???????4? ?? =15 5 = 20 ????4 ? Divide both sides by 20 4 0 0.25 = ????4 Take the 4th root Where 0 < ? <? 2, find the exact value of ?. 40.25 = ???? Inverse cos 40.25 = ? ??? 1 Calculate, with your calculator in radians Notice above, that when you differentiate ????, you get ???? in the answer This means that for ????, you should try differentiating it with the same power as in the answer 1 4? = ? 11D