Inductive and Deductive Reasoning
Inductive reasoning involves drawing general conclusions from specific observations, while deductive reasoning starts with general premises to derive specific conclusions. Induction uses experience or experimental evidence to make broad conclusions, while deduction follows from general to specific.
6 views • 7 slides
Dental Calculus Formation and Classification
Dental calculus, also known as tartar, is a mineralized bacterial plaque that forms on natural teeth and dental prostheses. It can be classified as supragingival or subgingival based on its relation to the gingival margin. This hard deposit is formed through the mineralization of dental plaque and c
5 views • 36 slides
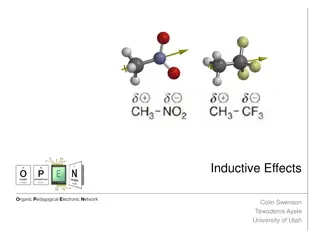
The Inductive and Electromeric Effects in Organic Chemistry
The inductive effect in organic chemistry is the polarization of a bond due to electron-donating or withdrawing effects of adjacent groups, leading to a degree of polarity in the bond. This effect is distance-dependent and can be either electron-withdrawing or electron-releasing. On the other hand,
0 views • 12 slides
Calculus Examples and Practice
Explore various calculus problems involving finding gradients, equations of tangents and normals, and analyzing curves. Practice determining gradients at specific points, solving for coordinates, and differentiating equations to find tangent and normal lines. Understand the relationship between grad
2 views • 11 slides
Inductive Effects in Organic Chemistry
Inductive effects play a crucial role in the reactivity and properties of organic molecules. This phenomenon involves the withdrawal or donation of electron density due to differences in electronegativity, impacting acidity, stability, and interactions with other molecules. Explore how inductive eff
0 views • 7 slides
Predicate Calculus: Symbols, Terms, and Variables
Predicate calculus extends propositional calculus by introducing symbols like truth values, constants, variables, and functions. It allows for precise manipulation of components within assertions, enabling the creation of general statements about classes of entities. Learn how predicates define rela
3 views • 14 slides
Inductive Inferences and Their Relation to Probability
Nevin Climenhaga delves into the world of inductive inferences, categorizing them into downward, upward, and sideways forms. He discusses connections with probability, confirmation, epistemic defeat, and more, emphasizing the distinction between direct and indirect forms of inferences.
0 views • 15 slides
Deductive and Inductive Reasoning
Explore the world of deductive and inductive arguments through examples of deductive reasoning based on definitions and math, including categorical syllogisms, hypothetical syllogisms, and disjunctive syllogisms. Delve into inductive reasoning and the key distinctions between deductive and inductive
1 views • 26 slides
Infinite Lottery Machines: An Inductive Approach
An in-depth exploration of the Material Theory of Induction applied to infinite lottery machines, discussing the challenges and strategies for making inductive inferences in such scenarios. The analysis covers probabilistic considerations, countable additivity issues, equal probability of outcomes,
0 views • 22 slides
Deductive and Inductive Reasoning in Problem-Solving
Explore the differences between deduction and induction in problem-solving approaches. Deductive reasoning starts with a general statement and moves to specifics, offering certainty and objectivity, while inductive reasoning begins with specifics and arrives at a generalization, providing flexibilit
0 views • 11 slides
Analyzing Texts Using Inductive Reasoning in Literary Interpretation
Utilizing inductive reasoning in literary interpretation involves examining specific details within a text to develop a debatable thesis statement supported by trends, patterns, and connections found in the material. This analytical approach requires a methodical breakdown of a writer's techniques t
0 views • 12 slides
Applications of Calculus in Optimization Problems
Calculus plays a crucial role in solving optimization problems to find maximum or minimum values in various real-life scenarios. This content provides examples of optimizing for maximum profit, area, distance, and volume using calculus concepts. From finding optimal dimensions for fencing to maximiz
0 views • 10 slides
Calculus: From MVT to FTC with Lin McMullin
Join Lin McMullin in exploring the transition from the Mean Value Theorem (MVT) to the Fundamental Theorem of Calculus (FTC). Discover the significance of MVT, Fermat's Theorem, Rolle's Theorem, and the Mean Value Theorem, all crucial concepts in calculus. Engage in graphical explorations, proving m
0 views • 45 slides
The Fundamental Theorem of Calculus
Explore the connection between differential calculus and the definite integral through the fundamental theorem of calculus, which allows for the evaluation of complex summations. Discover the properties of definite integrals and how to apply the theorem to find areas under curves. Practice evaluatin
0 views • 15 slides
Vector Calculus II Concepts with Examples by Prof. Somesh Kr. Malhotra
Explore advanced topics in vector calculus including gradient, divergence, curl, and theorems like the Divergence Theorem and Stokes' Theorem. Follow along with examples presented in Cartesian, spherical, and cylindrical coordinates to deepen your understanding of vector calculus concepts.
0 views • 29 slides
Programming by Example: A Journey into Inductive Synthesis
Delve into the realm of Programming by Example (PBE) and its motivating factors, distinctions from Programming by Demonstration (PBD), history of inductive learning, and the significance of generalization from observations. Explore how PBE and PBD fit into the landscape of inductive learning and mac
1 views • 32 slides
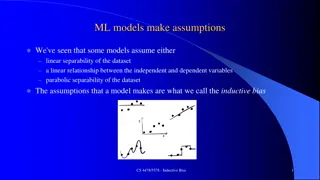
Inductive Bias in Machine Learning
Machine learning models rely on inductive bias, which are the assumptions made by algorithms to generalize from training data to unseen instances. Occam's Razor is a common example of inductive bias, favoring simpler hypotheses over complex ones. This bias helps algorithms make predictions and handl
0 views • 20 slides
Overfitting and Inductive Bias in Machine Learning
Overfitting can hinder generalization on novel data, necessitating the consideration of inductive bias. Linear regression struggles with non-linear tasks, highlighting the need for non-linear surfaces or feature pre-processing. Techniques like regularization in linear regression help maintain model
1 views • 37 slides
Introduction to Relational Calculus and Algebra in Database Management
Explore the fundamental concepts of relational calculus and algebra in the domain of database management. Understand the differences between declarative and imperative query languages and learn to retrieve information using practical examples and theoretical frameworks such as tuple relational calcu
0 views • 23 slides
Inductive Power Transfer System Market
The global inductive power transfer system (IPT) market is evaluated $27.5 billion in 2024, is projected to expand at a CAGR of 26.1%, to reach $280.5 billion by 2034.\n\nRead Report Overview: \/\/bisresearch.com\/industry-report\/inductive-power-tra
2 views • 3 slides
Propositional Calculus
Propositional calculus involves symbols representing true statements like "It is Monday" or "the car is red." Learn about connectives, semantics, and data-driven reasoning within this logical system. Predicate calculus expands on propositions by incorporating relationships between entities through p
0 views • 19 slides
Presented to you By:
Dive into essential strategies for conquering calculus anxiety, understanding derivative notation, and mastering key mathematical concepts. Explore practical tips and examples to enhance your skills and boost confidence in calculus. Overcome nervousness and confusion with expert guidance on function
0 views • 15 slides
Logic and Legal Reasoning
Inductive reasoning plays a crucial role in legal decision-making, especially in appellate cases. This article explores the concepts of inductive generalization and analogy in the context of legal reasoning. Examples and real-world cases demonstrate how legal principles are applied and generalized t
0 views • 14 slides
Computational Chemogenomics: Inductive Transfer vs. In Silico Drug Discovery
This article by J.B. Brown et al. explores the relationship between computational chemogenomics and inductive transfer in drug discovery. The authors discuss the nuances of leveraging data-driven approaches and machine learning in predicting molecular interactions and drug-protein interactions, high
0 views • 18 slides
Analyzing Inductive Arguments & Fallacies
Inductive arguments present conclusions based on likelihood, which can be strong, weak, or in-between. Learn about fallacies in reasoning, both formal and informal, and ways to spot and address them effectively.
1 views • 17 slides
Classical Mechanics and Mathematical Methods: Lecture 8 - Calculus of Variation
This content provides information on Lecture 8 focusing on the calculus of variation, including topics like the Brachistochrone problem, calculus of variation with constraints, and its applications in classical mechanics. Questions from students are addressed regarding assignments, integral equation
0 views • 33 slides
Methods of Teaching Mathematics by Dr. Meena Sharma
This content delves into the methods of teaching mathematics, specifically focusing on the inductive-deductive method as explained by Dr. Meena Sharma. It elaborates on both inductive and deductive methods, highlighting their differences and applications in teaching math effectively. The content dis
0 views • 5 slides
Applied Fractional Calculus Workshop Series
In this workshop series, delve into the realm of fractional calculus at the MESA Lab, UC Merced. Gain insights on research interests, achievements, and current focus, along with near-term goals. Explore the significance of fractional calculus and its applications in Mechatronics, Embedded Systems, a
0 views • 5 slides
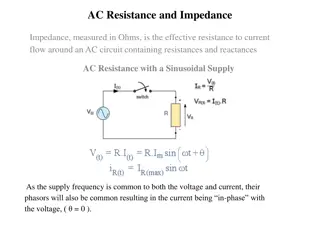
Understanding AC Resistance, Impedance, and Inductive Reactance
Explore the concept of AC resistance, impedance, and inductive reactance in electrical circuits. Learn how to calculate current, power, and impedance in AC circuits along with phasor diagrams. Discover the relationship between voltages and currents in sinusoidal waveforms.
0 views • 18 slides
Introduction to Stochastic Network Calculus in Electrical and Computer Engineering
Explore the world of Stochastic Network Calculus in the Department of Electrical and Computer Engineering at Xidian University. Learn about Network Calculus, Queueing Theory, and the foundations laid by R. Cruz. Discover how Deterministic and Stochastic Network Calculus provide different levels of s
0 views • 43 slides
Learning Decision Trees: Concepts, Inductive Learning, and Bias Analysis
Explore the concepts of decision trees in machine learning, including supervised learning, tree building, and generalization to multiple classes. Understand the expressiveness of decision trees and the importance of Occam's Razor in bias analysis while learning about inductive learning techniques.
1 views • 44 slides
Understanding Big O, Sorting Algorithms, and Inductive Proofs in Computer Science
Explore the concepts of Big O notation, sorting algorithms, and inductive proofs in computer science. Learn about loop invariants, insertion sort, and the differences between weak and strong inductive proofs. Discover how to analyze algorithms for termination, correctness, time complexity, and memor
0 views • 43 slides
Understanding Inductive and Deductive Reasoning
Explore the differences between inductive and deductive reasoning, where inductive involves making general statements from specific cases and deductive involves deriving specific instances from general statements. Learn how to identify and apply each type of reasoning through examples and explanatio
0 views • 13 slides
Key Issues in Inductive Learning and Programming by Example
Explore the key issues in inductive learning and programming by example, touching on topics such as the criticisms of synthesis, PBE vs PBD, historical perspectives, and important considerations in finding and verifying programs that match observations.
0 views • 30 slides
Discovering God's Love Through Inductive Bible Study
Explore John 3:16 and 2 Timothy 3:16 through inductive Bible study questions, understanding God's love, and the power of Scripture. Learn how to observe, interpret, and apply these verses in your life.
0 views • 15 slides
Calculus Preparation and Algebra Mastery for Science and Engineering
Discover the essential role of calculus in science and engineering education, emphasizing the significance of solid algebra preparation. Learn what it takes to excel in calculus and the background required for success. Gain insights from notable reports and examples to enhance your understanding and
0 views • 42 slides
Understanding the Properties of Continuous Functions in Advanced Calculus
Explore the key concepts in advanced calculus including the conditions and properties of continuous functions as presented by Vichar Shiwahre. Learn about continuity, Cauchy's definition, Borel's theorem, boundedness, the Mostest theorem, and more. Dive into the fascinating world of calculus with th
0 views • 22 slides
Understanding Extrema and First Derivative Test in Calculus
Explore the concept of extrema and the First Derivative Test in Calculus through examples and explanations. Learn about absolute maxima and minima, local maxima and minima, and the importance of algebraic accuracy. Develop a comprehensive understanding of finding extreme values and applying derivati
0 views • 27 slides
Understanding Antiderivatives in Calculus
Learn about antiderivatives in calculus, their importance in various fields, and how to find antiderivatives of functions. Discover the concept of anti-differentiation and examples to deepen your understanding of calculus concepts.
0 views • 8 slides
Enhancing Learning Through Inductive Thinking
Explore how inductive thinking boosts higher-order mental processes, effective learning, collaboration, and problem-solving in educational settings. Discover the power of inductive models in generating higher levels of information acquisition and retention. Uncover strategies for enhancing students'
0 views • 13 slides