Understanding Work, Energy, and Power in Physics

Slide Note

Work, Energy, and Power are fundamental concepts in physics. Work is the transfer of energy that results in displacement, requiring a force applied over a distance. Energy is the capacity to do work, while Power is the rate at which work is done. Examples illustrate how work is calculated in different scenarios, such as lifting objects against gravity or applying forces horizontally. Understanding these principles is crucial for comprehending the relationship between force, energy, and motion.

payleem Follow

Uploaded on Sep 13, 2024 | 0 Views

Download Presentation

Please find below an Image/Link to download the presentation.

The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author. Download presentation by click this link. If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.

E N D

Presentation Transcript

Work, Energy, and Power Work

What is Work and Energy? Energy(E)- the ability to do work Work (W)- A change in energy ( E) or the product of Force and distance (d) W=Fd Work is only being done when components of the applied force are parallel to the displacement Both are scalar and are measured in joules 1 joule=kg m2/s2 or 1 joule=N*m

When is work being done? If a hold a 30 kg object at a height of 1.5 meters is work being done? I am exerting energy . Work is NOT being done on the OBJECT, work is being done on muscles, but not the object The distance that the object has moved is zero so no work is done W=Fd W=F0

Example If the same 30 kg object was pushed to the right with an acceleration of 2m/s2 for 2.0 meters, how much work is done on the object? W=Fd Remember F=ma M=30 kg a=2 m/s2 d=2.0 m W=? F=ma F=30(2) = 60N W=Fd W=60(2) =120 J

Example If I were to lift the 30.0 kg object up off the ground to a height of 1.5 m, how much work is done on the object? When an object is being lifted against gravity, use g for the acceleration (9.8 m/s2) W=mgd m=30 kg g=9.8 m/s2 d=1.5 m W=? W=30(9.8)(1.5) W= 440 J

Clicker Question A 3.0 kg pineapple is held 1.2 m above the floor for 15 s. How much work is done on the pineapple? A)0.25 J B)54 J C)35 J D) 0 J

Clicker Question A 10.0 kg pumpkin is moved horizontally 5.00 m at a constant velocity across a level floor using a horizontal force of 3.00 N. How much work is done in moving the pumpkin? A) 30 J B) 294 J C) 15 J D) 0 J

Example- Honors A 50.0 kg banana box is pulled 11.0 m along a level surface by a rope. If the rope makes an angle with the floor of 35oand the tension in the rope is 90.0 N, how much work is done on the box? Use the force component that is parallel to the displacement! m=50.0 kg d=11.0 m Theta=35 degrees Fapplied=90.0 N W=? W=Fd F(x)=?

Example A 1385 kg car traveling at 61 km/h is brought to a stop while skidding 42 m. What is the work done on the car by frictional forces? m=1385 kg vi-=61 km/hr Vi=17 m/s x=42 m Vf=0 W=? W=Fd F=? F=ma

Nonconservative Forces The work produced by nonconservative forces are dependent on the paths taken Friction and air resistance are types of nonconservative forces A conservative force, such as gravity, are not dependent on the path taken

How does an angle impact work? If you have a 10.0 kg object, how much work is done on the object if it is lifted 1 meter straight off the ground? On a ramp that is 30 degrees off the ground (A)? 60 degrees(B)? 1.0m A B

How does an angle impact work? Wf = Ffd Ff = ukFN FN gets larger as the angle gets smaller So Ff gets smaller, But you still need to Add the Fg So the work Increases with a Higher angle 1.0m A B

Continued Work against gravity will not change, however! If they end at the same height the work against gravity will not change The amount of force needed will change according to the angle, but the distance will change as well to get to the same height So the work against gravity is the SAME

Work, Energy, and Power Potential Energy

Potential Energy Energy can either be potential energy or kinetic energy Potential energy is stored energy Examples Chemical Elastic (bungee cord, trampoline, bow) Electrical (static charges) Gravitational potential energy Energy can be converted into different forms by doing work

Gravitational Potential Energy Due to an object s position (height) measured relative to a reference point Gravitational Potential Energy Ep (or GPE) Ep=mgh m=mass g=9.8 m/s2 h=height

Example A 15.0 kg textbook is sitting on a 1.20 m tall table. If the book is lifted 0.80 m above the table, how much gravitational potential energy does it have: With respect of the table? With respect to the ground?

Clicker Question A 1400 kg roller coaster is moved to the top of a track that is 100 m above the lowest part of the track. What is the gravitional potential energy of the coaster? A) 2000 J B) 1.4 x 104 C) 34000 J D)1.4 x 106 J

Spring/Elastic Potential Energy The energy available for use in deformed elastic objects Rubber bands, springs in trampolines, pole-vault poles, muscles For springs, the distance compressed or stretched = x Spring constant (k) depends on stiffness of spring, measured in N/m Force needed to stretch the spring 1 meter

Example When a 2.00 kg mass is attached to a vertical spring, the spring is stretched 10.0 cm such that the mass is 50.0 cm above the table What is the gravitational potential energy associated with the mass relative to the table? What is the spring s elastic potential energy is the spring constant is 400.0 N/m?

Work, Energy and Power Kinetic Energy

Kinetic Energy Energy of motion scalar Ek=1/2 m v2 Ek= kinetic energy m=mass v=speed

Example A 60.0 kg student is running at a uniform speed of 5.70 m/s. What is the kinetic energy of the student? Ek=1/2 m v2 m=60 kg v=5.70 m/s Ek=? Ek=1/2(60)(5.7)2 Ek=975 J

Clicker Question The kinetic energy of a 2.1 kg rotten tomato is 1000 J. How fast is it moving? A) 15.4 m/s B) 31 m/s C) 961 m/s D) 4000 m/s

Work Energy Theorem If a net force is acting on an objet then the object must be accelerating The change in kinetic energy is proportional to the net force Ek=Fnetd d=distance

Example A sprinter exerts a net force of 260 N over a distance of 35 meters. What is his change in kinetic energy? Ek=Fnetd Fnet=260 N d= 35 m Ek=? Ek=260 (35) Ek=9100 J

Example A student pushes a 25 kg crate which is initially at rest with a force of 160 N over a distance of 15 meters. If there is 75 N of friction, what is the final speed of the crate? Ek=Fnetd Ek=1/2mv2 m=25 kg F applied=160 N d=15 m Ff=75 N

Work, Energy, and Power Conservation of Energy

Law of Conservation of Energy Energy cannot be created or destroyed, only converted into other forms of energy TOTAL energy is always conserved Potential energy can be converted to kinetic energy as an object moves When only conservative forces act on object potential energy is completely converted to kinetic energy When nonconservative forces like friction act on an object, some energy will be converted to heat

Mechanical Energy Mechanical Energy is the sum of kinetic energy and all forms of potential energy associated with an object ME=KE + PE When only conservative forces act on an object then mechanical energy is conserved

Law of Conservation of Energy (Quantitatively) Initial Energy= Final Energy Ei = Ef GPEi + Kei = GPEf + Kef mghi + mv2i = mghf + mv2f GPE=gravitational potential energy

Example A student falls from the building, if they reach the ground at 5.0 m/s , what height did they fall from? Vf=5.0 m/s Hi=? Vi=0 Hf=0 GPEi + KEi= GPEf + KEf

Example While jumping over The Great Wall of China an 82 kg skateboarder is needs to leave the ramp traveling at 22 m/s. A) How much potential energy is needed to jump over? B) What minimum height should the ramp be? m=82 kg Vf=22 m/s g=9.8 m/s2 GPE=? h=? Ei=Ef GPEi + KEi= GPEf + KEf GPEi =Kef GPE = mv2f

Clicker Question A 66 kg skateboarder jumps The Great Wall of China, clearly. At the peak of jump he is 18 m high and traveling at 12 m/s . Assuming he started at rest, find his initial height. A) 10 m B) 19 m C) 25 m D) 30 m

Clicker Question A 75 kg snowboarder slides up a frictionless rail to a height of 1.75m and slides across it at 2.50 m/s. How much kinetic energy did he have before he went up the rail? A) 1520 J B) 1380 J C) 200 J D) Impossible to solve

Roller Coasters Although not perfectly energy efficient, they are a fun way to view how work, gravitational potential and kinetic energy are exchanged

The Downhill skier When a nonconservative force is applied (friction) the work is negative because it is removing energy from the system What s this?

Work, Energy, and Power Power

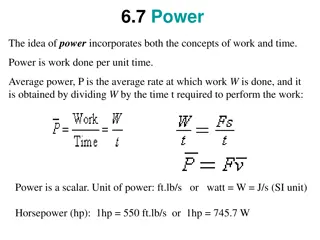
Power Power (P) is the rate of doing work Measured in J/s or Watts (W) Power= Work/time P=W/t or P= E/t

Clicker Question Mike performed 5 J of work in 10 seconds. Joe did 3 J of work in 5 seconds. Who produced the greater power? A) Mike B) Joe C) Both produced the same amount of power

Example Lover s Leap is a 122 m vertical climb. The record time of 4 min 25 s was achieved by Dan Osman (65 kg). What was his average power output during the climb? h=122 m t=4 min 25 s 265 s m=65 kg g=9.8 m/s2 P=? P=W/t or P= E/t

Example A 1.00x103 kg car accelerates from rest to a velocity of 15.0 m/s in 4.00 s. Calculate the power output of the car. Ignore friction. m=1.00 x 103 kg Vf=15 m/s t=4.00 s P=? P=W/t or P= E/t

Clicker Question A 68 kg student runs up a flight of stairs 3.2 m high in 4.8 seconds. Determine their power output while running up the stairs. A) 217.6 W B) 45.33 W C) 440 W D) There is no work in this problem, not enough info

Clicker Question A 642 kg formula 1 car can reach a speed of 27.78 m/s in 1.7 seconds. What is the power output of the car during this acceleration? A) 300,000 W B) 5000 W C) 150,000 W D) 3000 W

Another Useful Formula P=W/t P=Fd/t V=d/t So.. P=FV Note this formula is only useful when the velocity is held constant

Example A student uses 140 N to push a block up a ramp at a constant velocity of 2.2 m/s. What is their power output? F=140 N V=2.2 m/s P=? P=FV P=140 (2.2) P=310 W

Clicker Question An elevator motor has a power rating of 110 kW. How much force would it exert if it was lifting a load at a constant velocity of 3.0 m/s? A) 3700 N B) 37 N C) 330 N D) 4 N

Work, Energy, Power Efficiency

Efficiency A measure of how much of the energy that goes into a machine actually gets used Machines are useful because they allow us to use less force over a longer distance to do the same work