Converting Measurements using Ratio in Math Education

Explore the concept of converting measurements using ratio in Math education. Learn how to convert between customary and metric units through multiplication or division, and solve problems with ratio and proportion. Discover key vocabulary like Customary Unit, Metric Unit, Conversion, Ratio, and Proportion to enhance your understanding. Dive into sample problems and conversion rules to master this essential math skill.

• Math Education
• Measurement Conversion
• Ratio and Proportion
• Customary Unit
• Metric Unit

emilis Follow

Uploaded on Jul 16, 2024 | 2 Views

Download Presentation

Please find below an Image/Link to download the presentation.

The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author. Download presentation by click this link. If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.

Presentation Transcript

1. Ratio and Measurement Units Unit 1 Lesson 8 Math 6

2. Ratio and Measurement Units Students will be able to: Convert measurements using ratio through multiplication or division. Solve problems involving measurement conversions using the concept of ratio.

3. Ratio and Measurement Units Key Vocabulary: Customary Unit Metric Unit Conversion Convert Ratio Proportion

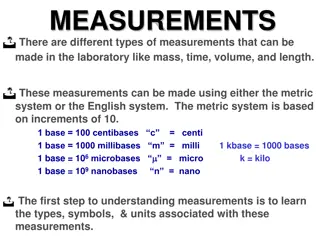
4. Ratio and Measurement Units Converting Measurements Using Ratio The concept of ratio and proportion are very helpful in converting measurements. These measurements include customary units such as feet, yard, inches, etc., and metric units such as meter, centimeter, millimeter, etc.

5. Ratio and Measurement Units Converting Measurements Using Ratio

6. Ratio and Measurement Units Table of Conversion Refer to table to convert one unit to another.

7. Ratio and Measurement Units Conversion Rules Using RATIO Example: How many gallons is equivalent to 40 quarts?

8. Ratio and Measurement Units Conversion Rules Using RATIO

9. Ratio and Measurement Units Conversion Rules Using RATIO

10. Ratio and Measurement Units Conversion Rules Using RATIO

11. Ratio and Measurement Units Sample Problem 1: How many feet is 72 inches?

12. Ratio and Measurement Units Converting Measurements Using A Conversion Factor Consider the previous example How many gallons is equivalent to 40 quarts? with its conversion factor 4 quarts = 1 gallon, if we treat it as an equation and divide both sides by 4 quarts here s what we get. 4 ?????? 4 ??????=1 ?????? 4 ??????

13. Ratio and Measurement Units Converting Measurements Using A Conversion Factor Cancelling 4 quarts gives us 1 on the left side of the equation and a ratio of 1 gallon is to 4 quarts on the right side. 1 ?????? 4 ?????? 1 = Now, we can go back to the problem

14. Ratio and Measurement Units How many gallons is equivalent to 40 quarts?

15. Ratio and Measurement Units How many gallons is equivalent to 40 quarts?

16. Ratio and Measurement Units How many gallons is equivalent to 40 quarts?

17. Ratio and Measurement Units Sample Problem 2: How many feet does 132 inches have? Use the conversion factor: 1 feet = 12 inches Solution: 1 ???? 12 ??? ??= 12 ??? ?? 12 ??? ?? 1 ???? 12 ??? ??= 1

18. Ratio and Measurement Units Sample Problem 2: How many feet does 132 inches have? Rule 1: Rule 2: 132 inches x 1 132 inches x 1 ???? 12 ??? ?? 1 ???? 12 ??? ?? Rule 3: 132 inches x 132 x 1 ???? 12 Rule 4: = 11 feet

19. Ratio and Measurement Units Solving Word Problems Using Raito to Convert Units The methods above can be used to solve problems involving unit conversions. Example: Matt rode 4 kilometers on his bike while his sister rode 6,000 meters. Who rode the farthest (in kilometers)?

20. Ratio and Measurement Units Solution: Since the problem requires us an answer in kilometers, we will convert the distance traveled by Matt s sister in kilometers. We know that 1 kilometer = 1000 meters.

21. Ratio and Measurement Units 1 ????????? 1000 ?????? Rule 1: 1 ????????? 1000 ??????= ? ?????????? 6000 ?????? Rule 2: Rule 3: 1000x = 6000 x = 6 kilometers, therefore Matt s sister travelled the farthest.

22. Ratio and Measurement Units Sample Problem 3: Sam is cutting a piece of rope that measures 70 cm. Jenny is cutting a piece of rope that measures 900 mm. How long are the two pieces of ropes combined together in centimeters?

23. Ratio and Measurement Units Solution: We know that 1 centimeter = 10 millimeters. Rule 1: 10 ??????????? Rule 2: 10 ???????????= 900 ??????????? Rule 3: 10x = 900 x = 90 cm, Jenny s rope is 90 cm. 1 ?????????? 1 ?????????? ? ??????????? Adding the two pieces of rope gives us 70 cm + 90 cm = 160cm